Текущая версия страницы пока не проверялась опытными участниками и может значительно отличаться от версии, проверенной 9 июня 2021 года; проверки требуют 7 правок.
Число́ Ре́йнольдса (
Число Рейнольдса также является критерием подобия течения вязкой жидкости.
Например, для прямых гладких труб критическое значение критерия Рейнольдса 



Установлено английским физиком Осборном Рейнольдсом (1842—1912) в 1883 году.
Определение[править | править код]
Число Рейнольдса определяется следующими соотношениями:
- где
— плотность среды, кг/м3;
— характерная скорость, м/с;
— гидравлический диаметр, м;
— динамическая вязкость среды, Па·с или кг/(м·с);
— кинематическая вязкость среды (
), м2/с;
— объёмный расход потока, м3/с;
— площадь сечения канала, например, трубы, м2.
Для каждого вида течения существует критическое число Рейнольдса, 
При 

Критическое значение числа Рейнольдса зависит от конкретного вида течения (например, течение в круглой трубе, обтекание шара и т. п.), различными возмущениями потока, такими как изменение направления и модуля вектора скорости потока, шероховатости стенок, близость местных сужений канала и др. Например, для течения (точнее, для стационарного изотермического потока) жидкости в прямой круглой трубе с очень гладкими стенками 
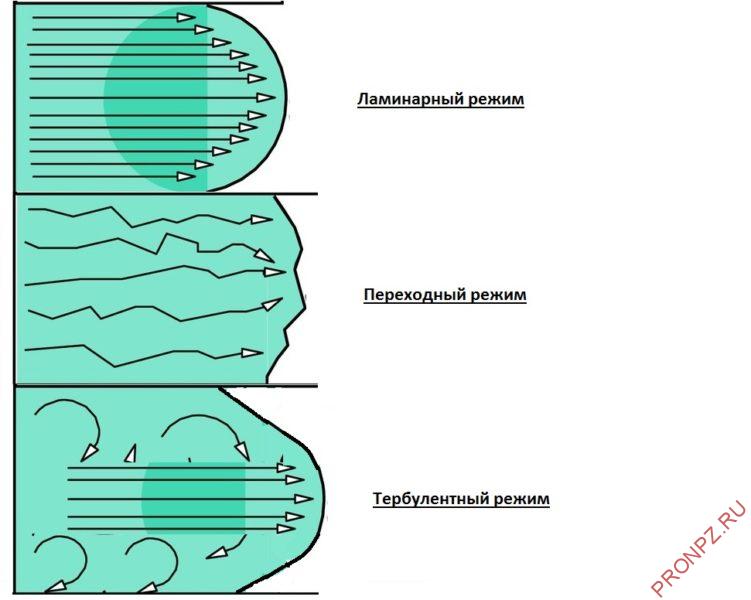
При значениях Re выше критического и до определённого предела наблюдается переходной (смешанный) режим течения жидкости, когда турбулентное течение более вероятно, но ламинарное в некоторых конкретных случаях тоже наблюдается — так называемая неустойчивая турбулентность. Числу 
Для газов 
Критерий назван в честь выдающегося английского физика Осборна Рейнольдса (1842—1912), автора многочисленных пионерских работ по гидродинамике.
Акустическое число Рейнольдса[править | править код]
В акустике пользуются числом Рейнольдса для количественной характеристики соотношения нелинейных и диссипативных членов в уравнении, описывающем распространение волны конечной амплитуды[4]. В этом случае число Рейнольдса принимает следующий вид:
- где
— плотность среды, кг/м3;
— амплитуда колебательной скорости, м/с;
— круговая частота, рад/с;
— скорость звука в среде, м/с;
— параметр диссипации.
Физический смысл[править | править код]
Число Рейнольдса есть мера отношения сил инерции, действующих в потоке, к силам вязкости. Плотность в числителе выражения 
Также число Рейнольдса можно рассматривать как отношение кинетической энергии жидкости к потерям энергии на характерной длине (ввиду внутреннего трения).
Если у потока число Рейнольдса многократно превышает критическое, то жидкость можно рассматривать как идеальную. В таком случае вязкостью жидкости можно пренебречь, так как толщина пограничного слоя мала по сравнению с характерным размером процесса, то есть силы вязкого трения существенны только в тонком слое, в потоке не наблюдается развитая турбулентность.
Примечания[править | править код]
- ↑ Монин А. С., Яглом А. М. Статистическая гидромеханика. — М.: Наука, главная редакция физико-математической литературы, 1965. — 640 с.
- ↑ Основы гидравлики. Глава 6. С. 144. Источник: сайт “Справочник химика 21 века”.
- ↑ Яворский Б. М., Детлаф А. А. Справочник по физике. — М.: «Наука», Главная редакция физико-математической литературы, 1968. — С. 339. — 940 с.
- ↑ Ультразвук, Советская энциклопедия, М., 1979, с. 303.
Литература[править | править код]
- Касаткин А. Г. Основные процессы и аппараты химической технологии. Изд. 8-е. Химия: Москва, 1971; с. 42 − 43; 118.
- Дытнерский Ю. И. Процессы и аппараты химической технологии. Часть 1. Теоретические основы процессов химической технологии. — М.: Химия, 1995. — 400 с. — 6500 экз. — ISBN 5-7245-1006-5.
Содержание
- 1 Калькулятор для расчета Re онлайн
- 1.1 Расчет по общей формуле
- 1.2 Расчет Re для воды
- 1.3 Расчет Re для воздуха
- 2 Формула
- 3 Физический смысл
- 4 Режимы течения
- 5 Критическое значение
- 6 Размерность
- 7 Течение в трубе
Калькулятор для расчета Re онлайн
Расчет по общей формуле
Расчет Re для воды
Расчет Re для воздуха
Формула
Расчетная формула числа Рейнольдса Re в общем виде:
- где
— плотность среды, кг/м3;
— характерная скорость, м/с;
— гидравлический диаметр, м;
— динамическая вязкость среды, Па·с или кг/(м·с);
— кинематическая вязкость среды (
), м2/с;
— объёмный расход потока, м3/с;
— площадь сечения канала, например, трубы, м2.
Для труб круглого сечения расчетная формула числа Рейнольдса Re будет:
![]()
Физический смысл
Физический смысл – число Рейнольдса Re характеризует смену режимов течения от ламинарного к турбулентному. Re является критерием подобия течения вязкой жидкости.
Критерий назван в честь выдающегося английского физика Осборна Рейнольдса (1842—1912).
В настоящее время не существует строгого научно доказанного объяснения этому явлению, однако наиболее достоверной гипотезой считается следующая: смена режимов движения жидкости определяется отношением сил инерции к силам вязкости в потоке жидкости. Если преобладают первые, то режим движения турбулентный, если вторые – ламинарный.
Режимы течения
Режим течения в динамическом пограничном слое зависит от числа Рейнольдса Re и может быть:
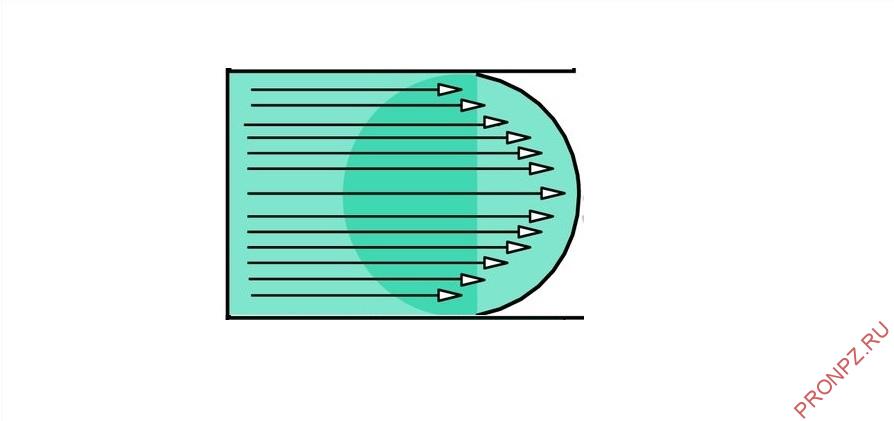
- Ламинарный режим – слоистое течение без перемешивания частиц жидкости и без пульсации скорости и давления, все линии тока направлены параллельно.
- Турбулентный режим – течение, сопровождающееся интенсивным перемешиванием жидкости с пульсациями скоростей и давлений, наряду с основным продольным перемещением жидкости наблюдаются поперечные перемещения и вращательные движения отдельных объемов жидкости.
Критическое значение
Переход к турбулентному режиму течения жидкости в пограничном слое определяется критическим значением числа Рейнольдса. Это обусловлено тем, что при возрастании скорости, участвующей в расчете числа Re, его значение растет.
Таким образом, переход от ламинарного режима к турбулентному наблюдается при определенной скорости движения жидкости. Эта скорость называется критической Vкр.
Значение критического числа Re для различных элементов гидропривода

Размерность
Числе Re не имеет единиц измерения. Re является безразмерным критерием подобия течения вязкой жидкости.
Течение в трубе
При ламинарном течении жидкости в прямой трубе или канале постоянного сечения все линии тока направлены параллельно оси трубы, при этом отсутствуют поперечные перемещения частиц жидкости.
При турбулентном течении в канале наряду с основным продольным перемещением жидкости в трубе наблюдаются поперечные перемещения и вращательные движения отдельных объемов жидкости.
Зависимость режима течения от значения числа Re в гладких трубах:
- <2100 – Ламинарный режим
- 2100 – 2300 – Переходный режим
- >2300 – Турбулентный режим
Обычно предполагается, что при числе Re выше 2300 образуется турбулентный режим.
Тем не менее, при значениях Re выше критического и до определённого предела наблюдается переходной (смешанный) режим течения жидкости, когда турбулентное течение более вероятно, но ламинарное в некоторых конкретных случаях тоже наблюдается — так называемая неустойчивая турбулентность. В трубах такой переходный интервал может достигать вплоть до Re = 2300—10 000.
2018-02-21
Расчет числа Рейнольдса
Число Рейнольдса – критерий гидродинамического подобия течения вязкой жидкости, отражающий отношение сил инерции к силам вязкого трения.
С помощью представленного калькулятора вы можете рассчитать число Рейнольдса (Re) и определить режим течения жидкости в режиме онлайн.
Расчетные формулы представлены ниже.
Результат расчета числа Рейнольдса
Дано: Скорость течения жидкости: 0.63 м/с, диаметр трубы: 100 мм, кинематическая вязкость жидкости: 1 ×10-6 м2/c
Вычислено: число Рейнольдса Re=63000
Определение режима течения
Режим течения жидкости при заданных условиях – турбулентный
Вычислить число Рейнольдса при других условиях
Критерий Рейнольдса
Для расчета числа Рейнольдса потока жидкости применяется формула:

где V – средняя скорость течения жидкости, м/с, ν – кинематическая вязкости, м2/с, Rг – гидравлический радиус, для круглой трубы Rг=d/4, d – диаметр трубы, м
Критерий Рейнольдса позволяет охарактеризовать режим течения жидкости.

Администрация сайта за результаты онлайн вычислений ответственности не несет.
Читайте также:

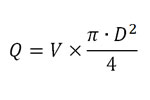
Все новости

The plume from this candle flame goes from laminar to turbulent. The Reynolds number can be used to predict where this transition will take place.


The transition from laminar (left) to turbulent (right) flow of water from a tap occurs as the Reynolds number increases.

A vortex street around a cylinder. This can occur around cylinders and spheres, for any fluid, cylinder size, and fluid speed provided that it has a Reynolds number between roughly 40 and 1000.[1]


In fluid mechanics, the Reynolds number (Re) is a dimensionless quantity that helps predict fluid flow patterns in different situations by measuring the ratio between inertial and viscous forces. At low Reynolds numbers, flows tend to be dominated by laminar (sheet-like) flow, while at high Reynolds numbers, flows tend to be turbulent. The turbulence results from differences in the fluid’s speed and direction, which may sometimes intersect or even move counter to the overall direction of the flow (eddy currents). These eddy currents begin to churn the flow, using up energy in the process, which for liquids increases the chances of cavitation.
The Reynolds number has wide applications, ranging from liquid flow in a pipe to the passage of air over an aircraft wing. It is used to predict the transition from laminar to turbulent flow and is used in the scaling of similar but different-sized flow situations, such as between an aircraft model in a wind tunnel and the full-size version. The predictions of the onset of turbulence and the ability to calculate scaling effects can be used to help predict fluid behavior on a larger scale, such as in local or global air or water movement, and thereby the associated meteorological and climatological effects.
The concept was introduced by George Stokes in 1851,[2] but the Reynolds number was named by Arnold Sommerfeld in 1908[3] after Osborne Reynolds (1842–1912), who popularized its use in 1883.[4][5]
Definition[edit]
The Reynolds number is the ratio of inertial forces to viscous forces within a fluid that is subjected to relative internal movement due to different fluid velocities. A region where these forces change behavior is known as a boundary layer, such as the bounding surface in the interior of a pipe. A similar effect is created by the introduction of a stream of high-velocity fluid into a low-velocity fluid, such as the hot gases emitted from a flame in air. This relative movement generates fluid friction, which is a factor in developing turbulent flow. Counteracting this effect is the viscosity of the fluid, which tends to inhibit turbulence. The Reynolds number quantifies the relative importance of these two types of forces for given flow conditions, and is a guide to when turbulent flow will occur in a particular situation.[6]
This ability to predict the onset of turbulent flow is an important design tool for equipment such as piping systems or aircraft wings, but the Reynolds number is also used in scaling of fluid dynamics problems and is used to determine dynamic similitude between two different cases of fluid flow, such as between a model aircraft, and its full-size version. Such scaling is not linear and the application of Reynolds numbers to both situations allows scaling factors to be developed.
With respect to laminar and turbulent flow regimes:
- laminar flow occurs at low Reynolds numbers, where viscous forces are dominant, and is characterized by smooth, constant fluid motion;
- turbulent flow occurs at high Reynolds numbers and is dominated by inertial forces, which tend to produce chaotic eddies, vortices and other flow instabilities.[7]
The Reynolds number is defined as[3]
where:
- ρ is the density of the fluid (SI units: kg/m3)
- u is the flow speed (m/s)
- L is a characteristic linear dimension (m) (see the below sections of this article for examples)
- μ is the dynamic viscosity of the fluid (Pa·s or N·s/m2 or kg/(m·s))
- ν is the kinematic viscosity of the fluid (m2/s).

The Reynolds number can be defined for several different situations where a fluid is in relative motion to a surface.[n 1] These definitions generally include the fluid properties of density and viscosity, plus a velocity and a characteristic length or characteristic dimension (L in the above equation). This dimension is a matter of convention – for example radius and diameter are equally valid to describe spheres or circles, but one is chosen by convention. For aircraft or ships, the length or width can be used. For flow in a pipe, or for a sphere moving in a fluid, the internal diameter is generally used today. Other shapes such as rectangular pipes or non-spherical objects have an equivalent diameter defined. For fluids of variable density such as compressible gases or fluids of variable viscosity such as non-Newtonian fluids, special rules apply. The velocity may also be a matter of convention in some circumstances, notably stirred vessels.
In practice, matching the Reynolds number is not on its own sufficient to guarantee similitude. Fluid flow is generally chaotic, and very small changes to shape and surface roughness of bounding surfaces can result in very different flows. Nevertheless, Reynolds numbers are a very important guide and are widely used.
History[edit]

Osborne Reynolds’s apparatus of 1883 demonstrating the onset of turbulent flow. The apparatus is still at the University of Manchester.

Diagram from Reynolds’s 1883 paper showing onset of turbulent flow.
Osborne Reynolds famously studied the conditions in which the flow of fluid in pipes transitioned from laminar flow to turbulent flow.
In his 1883 paper Reynolds described the transition from laminar to turbulent flow in a classic experiment in which he examined the behaviour of water flow under different flow velocities using a small stream of dyed water introduced into the centre of clear water flow in a larger pipe.
The larger pipe was glass so the behaviour of the layer of the dyed stream could be observed. At the end of this pipe, there was a flow control valve used to vary the water velocity inside the tube. When the velocity was low, the dyed layer remained distinct throughout the entire length of the large tube. When the velocity was increased, the layer broke up at a given point and diffused throughout the fluid’s cross-section. The point at which this happened was the transition point from laminar to turbulent flow.
From these experiments came the dimensionless Reynolds number for dynamic similarity—the ratio of inertial forces to viscous forces. Reynolds also proposed what is now known as the Reynolds averaging of turbulent flows, where quantities such as velocity are expressed as the sum of mean and fluctuating components. Such averaging allows for ‘bulk’ description of turbulent flow, for example using the Reynolds-averaged Navier–Stokes equations.
Flow in a pipe[edit]
For flow in a pipe or tube, the Reynolds number is generally defined as[8]
where
- DH is the hydraulic diameter of the pipe (the inside diameter if the pipe is circular) (m),
- Q is the volumetric flow rate (m3/s),
- A is the pipe’s cross-sectional area (A = πD2/4) (m2),
- u is the mean velocity of the fluid (m/s),
- μ (mu) is the dynamic viscosity of the fluid (Pa·s = N·s/m2 = kg/(m·s)),
- ν (nu) is the kinematic viscosity (ν = μ/ρ) (m2/s),
- ρ (rho) is the density of the fluid (kg/m3),
- W is the mass flowrate of the fluid (kg/s).
For shapes such as squares, rectangular or annular ducts where the height and width are comparable, the characteristic dimension for internal-flow situations is taken to be the hydraulic diameter, DH, defined as
where A is the cross-sectional area, and P is the wetted perimeter. The wetted perimeter for a channel is the total perimeter of all channel walls that are in contact with the flow.[9] This means that the length of the channel exposed to air is not included in the wetted perimeter.
For a circular pipe, the hydraulic diameter is exactly equal to the inside pipe diameter:
For an annular duct, such as the outer channel in a tube-in-tube heat exchanger, the hydraulic diameter can be shown algebraically to reduce to
where
- Do is the inside diameter of the outer pipe,
- Di is the outside diameter of the inner pipe.
For calculation involving flow in non-circular ducts, the hydraulic diameter can be substituted for the diameter of a circular duct, with reasonable accuracy, if the aspect ratio AR of the duct cross-section remains in the range 1/4 < AR < 4.[10]
Laminar–turbulent transition[edit]
In boundary layer flow over a flat plate, experiments confirm that, after a certain length of flow, a laminar boundary layer will become unstable and turbulent. This instability occurs across different scales and with different fluids, usually when Rex ≈ 5×105,[11] where x is the distance from the leading edge of the flat plate, and the flow velocity is the freestream velocity of the fluid outside the boundary layer.
For flow in a pipe of diameter D, experimental observations show that for “fully developed” flow,[n 2] laminar flow occurs when ReD < 2300 and turbulent flow occurs when ReD > 2900.[12][13] At the lower end of this range, a continuous turbulent-flow will form, but only at a very long distance from the inlet of the pipe. The flow in between will begin to transition from laminar to turbulent and then back to laminar at irregular intervals, called intermittent flow. This is due to the different speeds and conditions of the fluid in different areas of the pipe’s cross-section, depending on other factors such as pipe roughness and flow uniformity. Laminar flow tends to dominate in the fast-moving center of the pipe while slower-moving turbulent flow dominates near the wall. As the Reynolds number increases, the continuous turbulent-flow moves closer to the inlet and the intermittency in between increases, until the flow becomes fully turbulent at ReD > 2900.[12] This result is generalized to non-circular channels using the hydraulic diameter, allowing a transition Reynolds number to be calculated for other shapes of channel.[12]
These transition Reynolds numbers are also called critical Reynolds numbers, and were studied by Osborne Reynolds around 1895.[5] The critical Reynolds number is different for every geometry.[14]
Flow in a wide duct[edit]
For a fluid moving between two plane parallel surfaces—where the width is much greater than the space between the plates—then the characteristic dimension is equal to the distance between the plates.[15] This is consistent with the annular duct and rectangular duct cases above, taken to a limiting aspect ratio.
Flow in an open channel[edit]
For calculating the flow of liquid with a free surface, the hydraulic radius must be determined. This is the cross-sectional area of the channel divided by the wetted perimeter. For a semi-circular channel, it is a quarter of the diameter (in case of full pipe flow). For a rectangular channel, the hydraulic radius is the cross-sectional area divided by the wetted perimeter. Some texts then use a characteristic dimension that is four times the hydraulic radius, chosen because it gives the same value of Re for the onset of turbulence as in pipe flow,[16] while others use the hydraulic radius as the characteristic length-scale with consequently different values of Re for transition and turbulent flow.
Flow around airfoils[edit]
Reynolds numbers are used in airfoil design to (among other things) manage “scale effect” when computing/comparing characteristics (a tiny wing, scaled to be huge, will perform differently).[17] Fluid dynamicists define the chord Reynolds number R = Vc/ν, where V is the flight speed, c is the chord length, and ν is the kinematic viscosity of the fluid in which the airfoil operates, which is 1.460×10−5 m2/s for the atmosphere at sea level.[18] In some special studies a characteristic length other than chord may be used; rare is the “span Reynolds number”, which is not to be confused with span-wise stations on a wing, where chord is still used.[19]
Object in a fluid[edit]

The high viscosity of honey results in perfectly laminar flow when poured from a bucket, while the low surface tension allows it to remain sheet-like even after reaching the fluid below. Analogous to turbulence, when the flow meets resistance it slows and begins oscillating back and forth, piling upon itself.
The Reynolds number for an object moving in a fluid, called the particle Reynolds number and often denoted Rep, characterizes the nature of the surrounding flow and its fall velocity.
In viscous fluids[edit]

Creeping flow past a falling sphere: streamlines, drag force Fd and force by gravity Fg.
Where the viscosity is naturally high, such as polymer solutions and polymer melts, flow is normally laminar. The Reynolds number is very small and Stokes’ law can be used to measure the viscosity of the fluid. Spheres are allowed to fall through the fluid and they reach the terminal velocity quickly, from which the viscosity can be determined.
The laminar flow of polymer solutions is exploited by animals such as fish and dolphins, who exude viscous solutions from their skin to aid flow over their bodies while swimming. It has been used in yacht racing by owners who want to gain a speed advantage by pumping a polymer solution such as low molecular weight polyoxyethylene in water, over the wetted surface of the hull.
It is, however, a problem for mixing polymers, because turbulence is needed to distribute fine filler (for example) through the material. Inventions such as the “cavity transfer mixer” have been developed to produce multiple folds into a moving melt so as to improve mixing efficiency. The device can be fitted onto extruders to aid mixing.
Sphere in a fluid[edit]
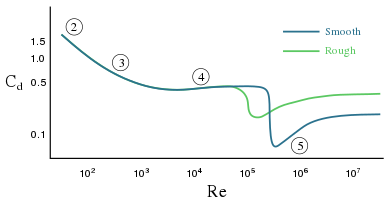
Drag coefficient Cd for a sphere as a function of Reynolds number Re, as obtained from laboratory experiments. The dark line is for a sphere with a smooth surface, while the lighter line is for the case of a rough surface (e.g. with small dimples). There is a range of fluid velocities where a rough-surfaced golf ball experiences less drag than a smooth ball. The numbers along the line indicate several flow regimes and associated changes in the drag coefficient:
- attached flow (Stokes flow) and steady separated flow,
- separated unsteady flow, having a laminar flow boundary layer upstream of the separation, and producing a vortex street,
- separated unsteady flow with a laminar boundary layer at the upstream side, before flow separation, with downstream of the sphere a chaotic turbulent wake,
- post-critical separated flow, with a turbulent boundary layer.
For a sphere in a fluid, the characteristic length-scale is the diameter of the sphere and the characteristic velocity is that of the sphere relative to the fluid some distance away from the sphere, such that the motion of the sphere does not disturb that reference parcel of fluid. The density and viscosity are those belonging to the fluid.[20] Note that purely laminar flow only exists up to Re = 10 under this definition.
Under the condition of low Re, the relationship between force and speed of motion is given by Stokes’ law.[21]
At higher Reynolds numbers the drag on a sphere depends on surface roughness. Thus, for example, adding dimples on the surface of a golf ball causes the boundary layer on the upstream side of the ball to transition from laminar to turbulent. The turbulent boundary layer is able to remain attached to the surface of the ball much longer than a laminar boundary and so creates a narrower low-pressure wake and hence less pressure drag. The reduction in pressure drag causes the ball to travel further.[22]
Rectangular object in a fluid[edit]
The equation for a rectangular object is identical to that of a sphere, with the object being approximated as an ellipsoid and the axis of length being chosen as the characteristic length scale. Such considerations are important in natural streams, for example, where there are few perfectly spherical grains. For grains in which measurement of each axis is impractical, sieve diameters are used instead as the characteristic particle length-scale. Both approximations alter the values of the critical Reynolds number.
Fall velocity[edit]
The particle Reynolds number is important in determining the fall velocity of a particle. When the particle Reynolds number indicates laminar flow, Stokes’ law can be used to calculate its fall velocity or settling velocity. When the particle Reynolds number indicates turbulent flow, a turbulent drag law must be constructed to model the appropriate settling velocity.
Packed bed[edit]
For fluid flow through a bed, of approximately spherical particles of diameter D in contact, if the voidage is ε and the superficial velocity is vs, the Reynolds number can be defined as[23]
or
or
The choice of equation depends on the system involved: the first is successful in correlating the data for various types of packed and fluidized beds, the second Reynolds number suits for the liquid-phase data, while the third was found successful in correlating the fluidized bed data, being first introduced for liquid fluidized bed system.[23]
Laminar conditions apply up to Re = 10, fully turbulent from Re = 2000.[20]
Stirred vessel[edit]
In a cylindrical vessel stirred by a central rotating paddle, turbine or propeller, the characteristic dimension is the diameter of the agitator D. The velocity V is ND where N is the rotational speed in rad per second. Then the Reynolds number is:
The system is fully turbulent for values of Re above 10000.[24]
Pipe friction[edit]

Pressure drops[25] seen for fully developed flow of fluids through pipes can be predicted using the Moody diagram which plots the Darcy–Weisbach friction factor f against Reynolds number Re and relative roughness ε/D. The diagram clearly shows the laminar, transition, and turbulent flow regimes as Reynolds number increases. The nature of pipe flow is strongly dependent on whether the flow is laminar or turbulent.
Similarity of flows[edit]
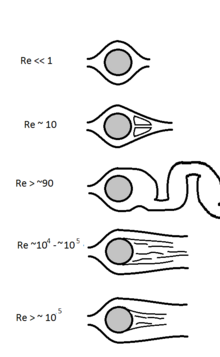
Qualitative behaviors of fluid flow over a cylinder depends to a large extent on Reynolds number; similar flow patterns often appear when the shape and Reynolds number is matched, although other parameters like surface roughness have a big effect.
In order for two flows to be similar, they must have the same geometry and equal Reynolds and Euler numbers. When comparing fluid behavior at corresponding points in a model and a full-scale flow, the following holds:
where 

The model numbers and design numbers should be in the same proportion, hence
This allows engineers to perform experiments with reduced scale models in water channels or wind tunnels and correlate the data to the actual flows, saving on costs during experimentation and on lab time. Note that true dynamic similitude may require matching other dimensionless numbers as well, such as the Mach number used in compressible flows, or the Froude number that governs open-channel flows. Some flows involve more dimensionless parameters than can be practically satisfied with the available apparatus and fluids, so one is forced to decide which parameters are most important. For experimental flow modeling to be useful, it requires a fair amount of experience and judgment of the engineer.
An example where the mere Reynolds number is not sufficient for the similarity of flows (or even the flow regime – laminar or turbulent) are bounded flows, i.e. flows that are restricted by walls or other boundaries. A classical example of this is the Taylor–Couette flow, where the dimensionless ratio of radii of bounding cylinders is also important, and many technical applications where these distinctions play an important role.[26][27] Principles of these restrictions were developed by Maurice Marie Alfred Couette and Geoffrey Ingram Taylor and developed further by Floris Takens and David Ruelle.
- Typical values of Reynolds number[28][29]
- Bacterium ~ 1 × 10−4
- Ciliate ~ 1 × 10−1
- Smallest fish ~ 1
- Blood flow in brain ~ 1 × 102
- Blood flow in aorta ~ 1 × 103
- Onset of turbulent flow ~ 2.3 × 103 to 5.0 × 104 for pipe flow to 106 for boundary layers
- Typical pitch in Major League Baseball ~ 2 × 105
- Person swimming ~ 4 × 106
- Fastest fish ~ 1 × 108
- Blue whale ~ 4 × 108
- A large ship (Queen Elizabeth 2) ~ 5 × 109
- Atmospheric tropical cyclone ~ 1 x 1012
Smallest scales of turbulent motion[edit]
In a turbulent flow, there is a range of scales of the time-varying fluid motion. The size of the largest scales of fluid motion (sometimes called eddies) are set by the overall geometry of the flow. For instance, in an industrial smoke stack, the largest scales of fluid motion are as big as the diameter of the stack itself. The size of the smallest scales is set by the Reynolds number. As the Reynolds number increases, smaller and smaller scales of the flow are visible. In a smokestack, the smoke may appear to have many very small velocity perturbations or eddies, in addition to large bulky eddies. In this sense, the Reynolds number is an indicator of the range of scales in the flow. The higher the Reynolds number, the greater the range of scales. The largest eddies will always be the same size; the smallest eddies are determined by the Reynolds number.
What is the explanation for this phenomenon? A large Reynolds number indicates that viscous forces are not important at large scales of the flow. With a strong predominance of inertial forces over viscous forces, the largest scales of fluid motion are undamped—there is not enough viscosity to dissipate their motions. The kinetic energy must “cascade” from these large scales to progressively smaller scales until a level is reached for which the scale is small enough for viscosity to become important (that is, viscous forces become of the order of inertial ones). It is at these small scales where the dissipation of energy by viscous action finally takes place. The Reynolds number indicates at what scale this viscous dissipation occurs.
In physiology[edit]
Poiseuille’s law on blood circulation in the body is dependent on laminar flow.[30] In turbulent flow the flow rate is proportional to the square root of the pressure gradient, as opposed to its direct proportionality to pressure gradient in laminar flow.
Using the definition of the Reynolds number we can see that a large diameter with rapid flow, where the density of the blood is high, tends towards turbulence. Rapid changes in vessel diameter may lead to turbulent flow, for instance when a narrower vessel widens to a larger one. Furthermore, a bulge of atheroma may be the cause of turbulent flow, where audible turbulence may be detected with a stethoscope.
Complex systems[edit]
Reynolds number interpretation has been extended into the area of arbitrary complex systems. Such as financial flows,[31] nonlinear networks,[citation needed] etc. In the latter case, an artificial viscosity is reduced to a nonlinear mechanism of energy distribution in complex network media. Reynolds number then represents a basic control parameter which expresses a balance between injected and dissipated energy flows for an open boundary system. It has been shown that Reynolds critical regime separates two types of phase space motion: accelerator (attractor) and decelerator.[32] High Reynolds number leads to a chaotic regime transition only in frame of strange attractor model.
Derivation[edit]
The Reynolds number can be obtained when one uses the nondimensional form of the incompressible Navier–Stokes equations for a newtonian fluid expressed in terms of the Lagrangian derivative:
Each term in the above equation has the units of a “body force” (force per unit volume) with the same dimensions of a density times an acceleration. Each term is thus dependent on the exact measurements of a flow. When one renders the equation nondimensional, that is when we multiply it by a factor with inverse units of the base equation, we obtain a form that does not depend directly on the physical sizes. One possible way to obtain a nondimensional equation is to multiply the whole equation by the factor
where
- V is the mean velocity, v or v, relative to the fluid (m/s),
- L is the characteristic length (m),
- ρ is the fluid density (kg/m3).
If we now set
we can rewrite the Navier–Stokes equation without dimensions:
where the term μ/ρLV = 1/Re.
Finally, dropping the primes for ease of reading:

Universal sedimentation equation — drag coefficient, a function of Reynolds number and shape factor, 2D diagram

Universal sedimentation equation — drag coefficient, a function of Reynolds number and shape factor, 3D diagram
This is why mathematically all Newtonian, incompressible flows with the same Reynolds number are comparable. Notice also that in the above equation, the viscous terms vanish for Re → ∞. Thus flows with high Reynolds numbers are approximately inviscid in the free stream.
Relationship to other dimensionless parameters[edit]
There are many dimensionless numbers in fluid mechanics. The Reynolds number measures the ratio of advection and diffusion effects on structures in the velocity field, and is therefore closely related to Péclet numbers, which measure the ratio of these effects on other fields carried by the flow, for example, temperature and magnetic fields. Replacement of the kinematic viscosity ν = μ/ρ in Re by the thermal or magnetic diffusivity results in respectively the thermal Péclet number and the magnetic Reynolds number. These are therefore related to Re by-products with ratios of diffusivities, namely the Prandtl number and magnetic Prandtl number.
See also[edit]
- Reynolds transport theorem – 3D generalization of the Leibniz integral rule
- Drag coefficient – Dimensionless parameter to quantify fluid resistance
- Deposition (geology) – Geological process in which sediments, soil and rocks are added to a landform or landmass
- Kelvin–Helmholtz instability
References[edit]
Footnotes[edit]
- ^ The definition of the Reynolds number is not to be confused with the Reynolds equation or lubrication equation.
- ^ Full development of the flow occurs as the flow enters the pipe, the boundary layer thickens and then stabilizes after several diameters distance into the pipe.
Citations[edit]
- ^ Tansley & Marshall 2001, pp. 3274–3283.
- ^ Stokes 1851, pp. 8–106.
- ^ a b Sommerfeld 1908, pp. 116–124.
- ^ Reynolds 1883, pp. 935–982.
- ^ a b Rott 1990, pp. 1–11.
- ^ Falkovich 2018.
- ^ Hall, Nancy (5 May 2015). “Boundary Layer”. Glenn Research Center. Retrieved 17 September 2019.
- ^ “Reynolds Number”. Engineeringtoolbox.com. 2003.
- ^ Holman 2002.
- ^ Fox, McDonald & Pritchard 2004, p. 348.
- ^ Incropera & DeWitt 1981.
- ^ a b c Schlichting & Gersten 2017, pp. 416–419.
- ^ Holman 2002, p. 207.
- ^ Potter, Wiggert & Ramadan 2012, p. 105.
- ^ Seshadri, K (February 1978). “Laminar flow between parallel plates with the injection of a reactant at high reynolds number”. International Journal of Heat and Mass Transfer. 21 (2): 251–253. doi:10.1016/0017-9310(78)90230-2.
- ^ Streeter 1965.
- ^ Lissaman 1983, pp. 223–239.
- ^ “International Standard Atmosphere”. eng.cam.ac.uk. Retrieved 17 September 2019.
- ^ Ehrenstein & Eloy 2013, pp. 321–346.
- ^ a b Rhodes 1989, p. 29.
- ^ Dusenbery 2009, p. 49.
- ^ “Golf Ball Dimples & Drag”. Aerospaceweb.org. Retrieved 11 August 2011.
- ^ a b Dwivedi 1977, pp. 157–165.
- ^ Sinnott, Coulson & Richardson 2005, p. 73.
- ^ “Major Head Loss – Friction Loss”. Nuclear Power. Retrieved 17 September 2019.
- ^ “Laminar, transitional and turbulent flow”. rheologic.net. Retrieved 17 September 2019.
- ^ Manneville & Pomeau 2009, p. 2072.
- ^ Patel, Rodi & Scheuerer 1985, pp. 1308–1319.
- ^ Dusenbery 2009, p. 136.
- ^ Helps, E. P. W.; McDonald, D. A. (1954-06-28). “Observations on laminar flow in veins”. The Journal of Physiology. 124 (3): 631–639. doi:10.1113/jphysiol.1954.sp005135. PMC 1366298. PMID 13175205.
- ^ Los 2006, p. 369.
- ^ Gómez Blázquez, Alberto (2016-06-23). “Aerodynamic analysis of the flat floor”.
Sources[edit]
- Bird, R. Byron; Stewart, Warren E.; Lightfoot, Edwin N. (2006). Transport Phenomena. John Wiley & Sons. ISBN 978-0-470-11539-8.
- Dusenbery, David B. (2009). Living at Micro Scale. Cambridge, Massachusetts: Harvard University Press. ISBN 9780674031166.
- Dwivedi, P. N. (1977). “Particle-fluid mass transfer in fixed and fluidized beds”. Industrial & Engineering Chemistry Process Design and Development. 16 (2): 157–165. doi:10.1021/i260062a001.
- Ehrenstein, Uwe; Eloy, Christophe (2013). “Skin friction on a moving wall and its implications for swimming animals” (PDF). Journal of Fluid Mechanics. 718: 321–346. Bibcode:2013JFM…718..321E. doi:10.1017/jfm.2012.613. ISSN 0022-1120. S2CID 56331294. Archived from the original (PDF) on 2019-03-02.
- Falkovich, Gregory (2018). Fluid Mechanics. Cambridge University Press. ISBN 978-1-107-12956-6.
- Fox, R. W.; McDonald, A. T.; Pritchard, Phillip J. (2004). Introduction to Fluid Mechanics (6th ed.). Hoboken: John Wiley and Sons. p. 348. ISBN 978-0-471-20231-8.
- Holman, J. P. (2002). Heat Transfer (Si Units ed.). McGraw-Hill Education (India) Pvt Limited. ISBN 978-0-07-106967-0.
- Incropera, Frank P.; DeWitt, David P. (1981). Fundamentals of heat transfer. New York: Wiley. ISBN 978-0-471-42711-7.
- Lissaman, P. B. S. (1983). “Low-Reynolds-Number Airfoils”. Annu. Rev. Fluid Mech. 15 (15): 223–39. Bibcode:1983AnRFM..15..223L. CiteSeerX 10.1.1.506.1131. doi:10.1146/annurev.fl.15.010183.001255.
- Los, Cornelis (2006). Financial Market Risk: Measurement and Analysis. Routledge. ISBN 978-1-134-46932-1.
- Manneville, Paul; Pomeau, Yves (25 March 2009). “Transition to turbulence”. Scholarpedia. 4 (3): 2072. Bibcode:2009SchpJ…4.2072M. doi:10.4249/scholarpedia.2072.
- Patel, V. C.; Rodi, W.; Scheuerer, G. (1985). “Turbulence Models for Near-Wall and Low Reynolds Number Flows—A Review”. AIAA Journal. 23 (9): 1308–1319. Bibcode:1985AIAAJ..23.1308P. doi:10.2514/3.9086.
- Potter, Merle C.; Wiggert, David C.; Ramadan, Bassem H. (2012). Mechanics of Fluids (4th, SI units ed.). Cengage Learning. ISBN 978-0-495-66773-5.
- Reynolds, Osborne (1883). “An experimental investigation of the circumstances which determine whether the motion of water shall be direct or sinuous, and of the law of resistance in parallel channels”. Philosophical Transactions of the Royal Society. 174: 935–982. Bibcode:1883RSPT..174..935R. doi:10.1098/rstl.1883.0029. JSTOR 109431.
- Rhodes, M. (1989). Introduction to Particle Technology. Wiley. ISBN 978-0-471-98482-5.
- Rott, N. (1990). “Note on the history of the Reynolds number” (PDF). Annual Review of Fluid Mechanics. 22 (1): 1–11. Bibcode:1990AnRFM..22….1R. doi:10.1146/annurev.fl.22.010190.000245. S2CID 54583669. Archived from the original (PDF) on 2019-02-25.
- Schlichting, Hermann; Gersten, Klaus (2017). Boundary-Layer Theory. Springer. ISBN 978-3-662-52919-5.
- Sinnott, R. K.; Coulson, John Metcalfe; Richardson, John Francis (2005). Chemical Engineering Design. Vol. 6 (4th ed.). Elsevier Butterworth-Heinemann. ISBN 978-0-7506-6538-4.
- Sommerfeld, Arnold (1908). “Ein Beitrag zur hydrodynamischen Erkläerung der turbulenten Flüssigkeitsbewegüngen (A Contribution to Hydrodynamic Explanation of Turbulent Fluid Motions)” (PDF). International Congress of Mathematicians . 3: 116–124. Archived from the original (PDF) on 2016-11-15.
- Stokes, George (1851). “On the Effect of the Internal Friction of Fluids on the Motion of Pendulums”. Transactions of the Cambridge Philosophical Society. 9: 8–106. Bibcode:1851TCaPS…9….8S.
- Streeter, Victor Lyle (1965). Fluid mechanics (3rd ed.). New York: McGraw-Hill. OCLC 878734937.
- Tansley, Claire E.; Marshall, David P. (2001). “Flow past a Cylinder on a Plane, with Application to Gulf Stream Separation and the Antarctic Circumpolar Current” (PDF). Journal of Physical Oceanography. 31 (11): 3274–3283. Bibcode:2001JPO….31.3274T. doi:10.1175/1520-0485(2001)031<3274:FPACOA>2.0.CO;2. Archived from the original (PDF) on 2011-04-01.
Further reading[edit]
- Batchelor, G. K. (1967). An Introduction to Fluid Dynamics. Cambridge University Press. pp. 211–215.
- Brezina, Jiri, 1979, Particle size and settling rate distributions of sand-sized materials: 2nd European Symposium on Particle Characterisation (PARTEC), Nürnberg, West Germany.
- Brezina, Jiri, 1980, Sedimentological interpretation of errors in size analysis of sands; 1st European Meeting of the International Association of Sedimentologists, Ruhr University at Bochum, Federal Republic of Germany, March 1980.
- Brezina, Jiri, 1980, Size distribution of sand – sedimentological interpretation; 26th International Geological Congress, Paris, July 1980, Abstracts, vol. 2.
- Fouz, Infaz “Fluid Mechanics,” Mechanical Engineering Dept., University of Oxford, 2001, p. 96
- Hughes, Roger “Civil Engineering Hydraulics,” Civil and Environmental Dept., University of Melbourne 1997, pp. 107–152
- Jermy M., “Fluid Mechanics A Course Reader,” Mechanical Engineering Dept., University of Canterbury, 2005, pp. d5.10.
- Purcell, E. M. “Life at Low Reynolds Number”, American Journal of Physics vol 45, pp. 3–11 (1977)[1]
- Truskey, G. A., Yuan, F, Katz, D. F. (2004). Transport Phenomena in Biological Systems Prentice Hall, pp. 7. ISBN 0-13-042204-5. ISBN 978-0-13-042204-0.
- Zagarola, M. V. and Smits, A. J., “Experiments in High Reynolds Number Turbulent Pipe Flow.” AIAA paper #96-0654, 34th AIAA Aerospace Sciences Meeting, Reno, Nevada, January 15–18, 1996.
- Isobel Clark, 1977, ROKE, a Computer Program for Non-Linear Least Squares Decomposition of Mixtures of Distributions; Computer & Geosciences (Pergamon Press), vol. 3, p. 245 – 256.
- B. C. Colby and R. P. Christensen, 1957, Some Fundamentals of Particle Size Analysis; St. Anthony Falls Hydraulic Laboratory, Minneapolis, Minnesota, USA, Report Nr. 12/December, 55 pages.
- Arthur T. Corey, 1949, Influence of Shape on the Fall Velocity of Sand Grains; M. S. Thesis, Colorado Agricultural and Mechanical College, Fort Collins, Colorado, USA, December 102 pages.
- Joseph R. Curray, 1961, Tracing sediment masses by grain size modes; Proc. Internat. Association of Sedimentology, Report of the 21st Session Norden, Internat. Geol. Congress, p. 119 – 129.
- Burghard Walter Flemming & Karen Ziegler, 1995, High-resolution grain size distribution patterns and textural trends in the back-barrier environment of Spiekeroog Island (Southern North Sea); Senckenbergiana Maritima, vol. 26, No. 1+2, p. 1 – 24.
- Robert Louis Folk, 1962, Of skewnesses and sands; Jour. Sediment. Petrol., vol. 8, No. 3/September, p. 105 – 111
- FOLK, Robert Louis & William C. WARD, 1957: Brazos River bar: a study in the significance of grain size parameters; Jour. Sediment. Petrol., vol. 27, No. 1/March, p. 3 – 26
- George Herdan, M. L. Smith & W. H. Hardwick (1960): Small Particle Statistics. 2nd revised edition, Butterworths (London, Toronto, etc.), 418 pp.
- Douglas Inman, 1952: Measures for describing the size distribution of sediments. Jour. Sediment. Petrology, vol. 22, No. 3/September, p. 125 – 145
- Miroslaw Jonasz, 1991: Size, shape, composition, and structure of microparticles from light scattering; in SYVITSKI, James P. M., 1991, Principles, Methods, and Application of Particle Size Analysis; Cambridge Univ. Press, Cambridge, 368 pp., p. 147.
- William C. Krumbein, 1934: Size frequency distribution of sediments; Jour. Sediment. Petrol., vol. 4, No. 2/August, p. 65 – 77.
- Krumbein, William Christian & Francis J. Pettijohn, 1938: Manual of Sedimentary Petrography; Appleton-Century-Crofts, Inc., New York; 549 pp.
- John S. McNown & Pin-Nam Lin, 1952, Sediment concentration and fall velocity; Proc. of the 2nd Midwestern Conf. on Fluid Mechanics, Ohio State University, Columbus, Ohio; State Univ. of Iowa Reprints in Engineering, Reprint No. 109/1952, p. 401 – 411.
- McNownn, John S. & J. Malaika, 1950, Effects of Particle Shape of Settling Velocity at Low Reynolds’ Numbers; American Geophysical Union Transactions, vol. 31, No. 1/February, p. 74 – 82.
- Gerard V. Middleton 1967, Experiments on density and turbidity currents, III; Deposition; Canadian Jour. of Earth Science, vol. 4, p. 475 – 505 (PSI definition: p. 483 – 485).
- Osborne Reynolds, 1883: An experimental investigation of the circumstances which determine whether the motion of water shall be direct or sinuous, and of the law of resistance in parallel channels. Phil. Trans. Roy. Soc., 174, Papers, vol. 2, p. 935 – 982
- E. F. Schultz, R. H. Wilde & M. L. Albertson, 1954, Influence of Shape on the Fall Velocity of Sedimentary Particles; Colorado Agricultural & Mechanical College, Fort Collins, Colorado, MRD Sediment Series, No. 5/July (CER 54EFS6), 161 pages.
- H. J. Skidmore, 1948, Development of a stratified-suspension technique for size-frequency analysis; Thesis, Department of Mechanics and Hydraulics, State Univ. of Iowa, p. 2 (? pages).
- James P. M. Syvitski, 1991, Principles, Methods, and Application of Particle Size Analysis; Cambridge Univ. Press, Cambridge, 368 pp.
External links[edit]
- The Reynolds number – The Feynman Lectures on Physics
- The Reynolds Number at Sixty Symbols
- Reynolds mini-biography and picture of original apparatus at Manchester University.
Содержание
Определение гидравлического диаметра
Круг является самой простой формой, поэтому проще всего производить вычисления при работе с круглыми поперечными сечениями. Когда жидкость течет по некруглому каналу, мы переводим поперечное сечение в круглое для удобства расчетов. Этот недавно полученный диаметр круглого поперечного сечения называется гидравлический диаметр. Обозначается как Dh. Следовательно, мы можем получить те же результаты для некруглого воздуховода, что и для круглого воздуховода, используя концепцию гидравлического диаметра.
Уравнение гидравлического диаметра
Гидравлический диаметр можно найти по формуле, приведенной ниже:
Дч = 4А/П
Где,
Dh гидравлический диаметр
A – площадь некруглого поперечного сечения
P – смоченный периметр некруглого поперечного сечения
Гидравлический диаметр зависит от гидравлического радиуса Rh, который можно найти, разделив площадь поперечного сечения A на смоченный периметр P.
Отметим, что Dh = 4RhЭто соотношение отличается от обычного соотношения между диаметром и радиусом (т.е. D = 2R). Эта разница возникает только при преобразовании некруглых сечений в круглые.
Примечание. Закон сохранения количества движения соблюдается при расчете гидравлического диаметра. Кроме того, гидравлический диаметр отличается от обычного диаметра. Dh то же самое только для круглых каналов.

Гидравлический диаметр и число Рейнольдса
Число Рейнольдса используется в гидромеханике и теплопередача, чтобы найти тип потока, ламинарный или турбулентный. Гидравлический диаметр используется в формуле для расчета числа Рейнольдса.
Число Рейнольдса – это отношение сил инерции к силам вязкости. Это безразмерное число названо в честь ирландского ученого Осборна Рейнольдса, который популяризировал эту концепцию в 1883 году.
Это число показывает влияние вязкости на контроль скорости течения жидкости. Линейный профиль вязкости развивается при ламинарном течении. При ламинарном течении жидкость течет так, как будто она течет параллельными слоями. Эти слои не пересекаются друг с другом и перемещаются без каких-либо разрывов между ними. Этот тип потока обычно происходит на малых скоростях. При малых скоростях смешивания двух слоев не происходит и жидкость течет слоями, наложенными друг на друга.
Ламинарный поток помогает нам измерять поток высоковязких жидкостей, поскольку этот тип потока дает линейную зависимость между скоростью потока и падение давления. Благоприятный условия ламинарного течения высокая вязкость и низкая скорость. При более высоких скоростях частицы жидкости начинают вести себя по-другому, что приводит к перемешиванию слоев жидкости. Такое перемешивание приводит к возникновению турбулентности, отсюда и название турбулентного течения. Турбулентный поток желателен, когда требуется надлежащее перемешивание жидкости. Одним из таких примеров является смешивание топлива с окислителем в ракетных двигателях. Турбулентность способствует тщательному перемешиванию жидкости.
Число Рейнольдса можно рассчитать из уравнения, приведенного ниже:
Где,
Re – число Рейнольдса
u – средняя скорость (в м / с)
ν – кинематическая вязкость (в м2/ с)
Dh – гидравлический диаметр (в м)
В круглой трубе
Ламинарный поток, Re <2000
Переходный поток, 2000 <Re <4000
Турбулентный поток, Re> 4000
Для плоской тарелки
Ламинарный поток, Re <5,00,000
Турбулентный поток, Re > 5,00,000 XNUMX XNUMX

Гидравлический диаметр круглой трубы | гидравлический диаметр цилиндра
Круглые трубы чаще всего используются для транспортировки жидкости / газа из одного места в другое (даже на большие расстояния). Водопроводы – это реальный пример круглых каналов, которые используются для транспортировки жидкости. Эти трубы могут проходить на большие расстояния, например, от станций фильтрации воды до домов, а также на короткие расстояния, например, от резервуара с грунтовой водой до резервуара для воды на террасе. Гидравлический диаметр круглой трубы определяется следующим образом:
Dh = 4πR2/2πR = 2R
Где,
R – радиус круглого сечения.

Гидравлический диаметр прямоугольного воздуховода
Прямоугольные воздуховоды используются, когда возникает проблема с зазором. Кроме того, прямоугольные воздуховоды просты в изготовлении и снижают потери давления. В кондиционерах используются прямоугольные воздуховоды, чтобы избежать потерь давления. Гидравлический диаметр прямоугольного воздуховода определяется по формуле:
Dh = 4ab/2(a+b) = 2ab/ a+b
Где,
a и b – длины большей и меньшей сторон.

Для квадратного сечения
a = b
Дх = 2а2/2а = а
Где,
а – длина каждой стороны квадрата.
Гидравлический диаметр затрубного пространства
Иногда для увеличения / уменьшения скорости теплопередачи через кольцевую трубу пропускают две жидкости, так что одна жидкость выходит за пределы другой. На скорость теплопередачи влияет действие двух жидкостей. Гидравлический диаметр кольцевого пространства определяется по формуле:
Где D и d – диаметры внешнего и внутреннего круга соответственно.

Гидравлический диаметр треугольника
Где,
l – длина каждой стороны.

Гидравлический диаметр эллипса
Дх = 4wh(64-16е2)/ш+ч(64-3е4)
Где, е = что/ш+ч
Гидравлический диаметр пластинчатого теплообменника | гидравлический диаметр кожухотрубного теплообменника
Теплообменники – это тепловые устройства, используемые для передачи тепла от одной жидкости к другой с целью уменьшения / увеличения температуры жидкости по желанию. Существует множество типов теплообменников, из которых наиболее часто используются пластинчатые и кожухотрубные теплообменники. Жидкости могут проходить через теплообменник двумя способами. В первом типе и горячие, и холодные жидкости нагнетаются в одном и том же направлении, поэтому он называется теплообменником с параллельным потоком. Во втором типе жидкости проходят через трубку в противоположных направлениях, поэтому он называется противоточным теплообменником.
Исходя из этого и спроектированы испаритель и конденсатор. В испарителе температура горячей жидкости остается неизменной, в то время как холодная жидкость становится теплее. В конденсаторе температура холодной жидкости остается прежней, а температура более горячей жидкости снижается.
Скорость передачи в теплообменнике определяется следующим соотношением:
Для горячей жидкости: Qh = мh Cph (Thi – Тho )
Для холодной жидкости: Qc = мc Cpc (Tco – Тci )
Сохраняя энергию,
Тепло, потерянное горячей жидкостью = тепло, полученное холодной жидкостью.
=> Qh = QcГде,
Qh означает потерю тепла горячей жидкостью
Qc обозначает тепло, получаемое холодной жидкостью
Thi температура горячей жидкости на входе
Tho температура горячей жидкости на выходе
Tci температура холодной жидкости на входе
Tco температура холодной жидкости на выходе
mh масса горячей жидкости (в кг)
mc масса холодной жидкости (в кг)
Cph – удельная теплоемкость горячей жидкости (в Дж / К-кг)
Cpc – удельная теплоемкость холодной жидкости (в Дж / К-кг)
В пластинчатых теплообменниках тепло проходит через секцию и разделяет горячую и холодную жидкости. Этот тип теплообменника используется во многих промышленных приложениях. Они используются в Тепловой насос, системы охлаждения масла, система охлаждения двигателя, системы хранения тепла и т. д.
Пластинчатый теплообменник имеет прямоугольное / квадратное поперечное сечение, следовательно, гидравлический диаметр определяется следующим образом:
Дч = 2аб/а+б
Где,
a и b – длина более короткой стороны и более длинной стороны соответственно.

Изображение кредита: https://commons.wikimedia.org/wiki/File:Plate_frame_1.svg
В оболочке и трубке тип теплообменник, трубы установлены в цилиндрической оболочке. По этим трубкам проходят как горячие, так и холодные жидкости таким образом, что одна жидкость течет вне другой жидкости. За счет этого происходит передача тепла от одной жидкости к другой. Теплообменник кожухового типа широко используется в промышленности, в основном в химических процессах и применениях, где требуется высокое давление.
Оболочка трубы теплообменник имеет кольцевое поперечное сечение, следовательно, гидравлический диаметр определяется выражением
Dh = Dd
Кожухотрубный теплообменник
Изображение кредита: Теплообменник прямотрубный 2-х ходовой
Эквивалентный диаметр и гидравлический диаметр
Эквивалентный диаметр и гидравлический диаметр различаются по значениям. Диаметр круглого воздуховода, который дает то же самое давление потери в прямоугольном канале для равного расхода называются эквивалентным диаметром. Несмотря на то, что круглые воздуховоды имеют наименьшую площадь поверхности для данной потери давления, они не подходят для изготовления. Прямоугольные воздуховоды просты в изготовлении, поэтому они используются в практических случаях. Когда скорость потока и падение давления известно, то для проектирования прямоугольного воздуховода мы используем диаграмму трения, чтобы найти эквивалентный диаметр, а затем требуемые размеры, фиксируя определенные параметры, такие как соотношение сторон или длина любой стороны.
Отношение длины более короткой стороны к более длинной стороне называется соотношением сторон.
АР = а/б
Мы можем найти эквивалентный диаметр по уравнению эквивалентного диаметра Хюбшера. Это показано ниже –
De = 1.30 (аб)0.625/(а+б)0.25
Где,
a и b – длина более короткой стороны и длинной стороны соответственно.
Недавние исследования пришли к выводу, что эквивалентный диаметр, полученный из эмпирических соотношений, не является надежным при расчете потерь давления в трубах. Следовательно, мы используем гидравлический диаметр во всех случаях.
В чем разница между гидравлическим диаметром, эквивалентным диаметром и характеристической длиной в гидромеханике и теплопередаче?
Гидравлический диаметр, как обсуждалось ранее, представляет собой новый диаметр, полученный из некруглого воздуховода, так что характеристики потока остаются такими же. Гидравлический диаметр используется для расчета числа Рейнольдса, которое помогает нам понять, является ли поток ламинарным, переходным или турбулентным.
Диаметр круглого воздуховода, который дает то же самое давление потери в прямоугольном канале для равного расхода называются эквивалентным диаметром.
Потеря давления в трубе определяется уравнением Дарси-Вейсбаха:
Где,
ρ – плотность жидкости (кг / м ^ 3)
D – гидравлический диаметр трубы (в м)
l – длина трубы (в м)
v – средняя скорость потока (в м / с). Характеристическая длина – это, по сути, объем системы, деленный на ее площадь поверхности.
В некоторых случаях он может быть равен гидравлическому диаметру.
Математически,
Lc V =поверхность/Aповерхность
Для квадратного воздуховода
Lc = a
Для прямоугольного воздуховода
Lc = 2ab/a+b
В теплопередаче характеристическая длина используется для расчета числа Нуссельта. Отношение конвективной теплопередачи к кондуктивной теплопередаче называется числом Нуссельта. Он показывает, какой тип теплопередачи преобладает.
Число Нуссельта, Nu определяется как
Nu = hLc/k
где,
h – конвективное тепловое сопротивление
L – характерная длина
k это теплопроводность
Число Нуссельта, равное 1, представляет теплопередача при чистой теплопроводности по мере увеличения числа Нуссельта теплопередача за счет конвекции продолжает увеличиваться. При значении числа Нуссельта, приближающемся к 100-1000, преобладает конвективный теплообмен. Значение числа Нуссельта не может быть меньше 1, оно может быть больше 1 или равно 1. Значение числа Нуссельта всегда постоянно для полностью развитых ламинарный поток. Для сложной формы вычисляются локальные числа Нуссельта для поверхности, а затем вычисляется среднее число Нуссельта с использованием этих локальных чисел Нуссельта. Среднее число Нуссельта используется для получения дальнейших выводов.
В чем разница между гидравлическим радиусом и гидравлической глубиной / средней гидравлической глубиной?
Существует заблуждение, что гидравлический радиус и гидравлическая глубина одинаковы. Оба они имеют разное значение и имеют индивидуальное значение при измерении свойств жидкости. Понятие гидравлического радиуса и гидравлической глубины подробно обсуждается ниже.
Отношение площади поперечного сечения потока к смоченному периметру называется гидравлическим радиусом.
Rh = A / P
Отношение площади поперечного сечения потока к свободной поверхности воды или ширине верхней поверхности называется гидравлической глубиной.
Hd = A / T
где,
A – площадь поперечного сечения потока
T – ширина до верхней или свободной поверхности.
Математически средняя гидравлическая глубина и гидравлический радиус одинаковы.
Каково физическое значение гидравлического диаметра в науках о жидкости и теплоте?
На практике число Рейнольда используется для проверки поведения или характера потока жидкости. Это, в свою очередь, помогает нам найти число Нуссельта, которое затем используется для определения скорости передачи тепла из закрытого трубопровода.
Следовательно, число Рейнольдса – очень важное безразмерное число, которое играет жизненно важную роль как в науках о жидкости, так и в тепловых науках. Но чтобы найти число Рейнольда, сначала нам нужно найти гидравлический диаметр закрытого водовода. Для некруглого поперечного сечения гидравлический диаметр обеспечивает такое значение диаметра, что его характеристики потока эквивалентны характеристикам круглого поперечного сечения.
Отношение конвективной теплопередачи к кондуктивной теплопередаче называется числом Нуссельта.
Число Нуссельта определяется следующим соотношением:
Для ламинарный поток: Nu = 0.332 Re0.5 Pr0.33
Для турбулентного потока: Nu = 0.039 Re0.8 Pr0.33
Где,
Re обозначает число Рейнольдса
Pr обозначает число Прандтля
Отношение коэффициента диффузии по импульсу к коэффициенту температуропроводности называется Число Прандтля. Он назван в честь немецкого ученого Людвига Прандтля. Это безразмерное число помогает нам в вычислениях, связанных с принудительная и естественная конвекция тепла. Его значение состоит в том, что он помогает нам изучить связь между переносом импульса и способностью переноса тепла жидкостью.
Число Прандтля рассчитывается по формуле, приведенной ниже:
Pr = μCп / к
Где,
Pr – число Прандтля
µ является динамическим вязкость
Cp – теплоемкость
Обратите внимание, что число Нуссельта также можно найти с помощью соотношения: Nu = hLc / k, когда мы знаем значения конвективного и кондуктивного теплового сопротивления.
Проще говоря, гидравлический диаметр является основой для определения поведения потока и скорости потока. тепло переход от жидкости, которая течет в закрытом трубопроводе. При этом он также упрощает расчеты путем преобразования некруглого канала в круглый.


















