Деление c остатком — арифметическая операция, играющая большую роль в арифметике, теории чисел, алгебре и криптографии. Чаще всего эта операция определяется для целых или натуральных чисел следующим образом[1]. Пусть 






Таким образом, результатами деления с остатком являются два целых числа: 





Нахождение неполного частного также называют целочисленным делением, а нахождение остатка от деления называют взятием остатка или, неформально, делением по модулю (однако последний термин стоит избегать, так как он может привести к путанице с делением в кольце или группе вычетов по аналогии со сложением или умножением по модулю).
- Примеры
-
- Проверка:
- Проверка:
-
- Проверка:
- Проверка:
-
- Проверка:
- Проверка:
-
- Проверка:
- Проверка:
Операция деления с остатком может быть определена не только для целых чисел, но и для других математических объектов (например, для многочленов), см. ниже.
Определение[править | править код]
Оставаясь строго в рамках натуральных чисел, приходится различать деление с остатком и деление нацело, поскольку нулевой остаток не является натуральным числом; кроме того, неполное частное при делении меньшего числа на большее должно равняться нулю, что тоже выводит за рамки натуральных чисел. Все эти искусственные ограничения неоправданно усложняют формулировки, поэтому в источниках обычно либо рассматривается расширенный натуральный ряд, включающий ноль[2], либо теория сразу формулируется для целых чисел, как указано выше[1].
Для вычисления неполного частного от деления 



когда
.
где полускобки 


Для отрицательного делителя нужно округлять частное в большую сторону:
когда
.
Операция «mod» и связь со сравнениями[править | править код]
Величина остатка может быть получена бинарной операцией «взятия остатка» от деления 

Не следует путать это обозначение с обозначением сравнения по модулю 

однако обратная импликация, вообще говоря, неверна. А именно, это сравнение не подразумевает выполнения неравенства 

В программировании[править | править код]
| Язык | Неполное частное |
Остаток | Знак остатка |
|---|---|---|---|
| ActionScript | % |
Делимое | |
| Ada | mod |
Делитель | |
rem |
Делимое | ||
| Бейсик | |
MOD |
Не определено |
| Си (ISO 1990) | / |
% |
Не определено |
| Си (ISO 1999) | / |
% |
Делимое[3] |
| C++ (ISO 2003) | / |
% |
Не определено[4] |
| C++ (ISO 2011) | / |
% |
Делимое[5] |
| C# | / |
% |
Делимое |
| ColdFusion | MOD |
Делимое | |
| Common Lisp | mod |
Делитель | |
rem |
Делимое | ||
| D | / |
% |
Делимое[6] |
| Delphi | div |
mod |
Делимое |
| Eiffel | // |
\ |
Делимое |
| Erlang | div |
rem |
Делимое |
| Euphoria | remainder |
Делимое | |
| Microsoft Excel (англ.) | QUOTIENT() |
MOD()
|
Делитель |
| Microsoft Excel (рус.) | ЧАСТНОЕ() |
ОСТАТ()
|
|
| FileMaker | Div() |
Mod() |
Делитель |
| Fortran | mod |
Делимое | |
modulo |
Делитель | ||
| GML (Game Maker) | div |
mod |
Делимое |
| Go | / |
% |
Делимое |
| Haskell | div
|
mod |
Делитель |
quot
|
rem |
Делимое | |
| J | |~ |
Делитель | |
| Java | /
|
%
|
Делимое[7] |
Math.floorDiv
|
Math.floorMod
|
Делитель (1.8+) | |
| JavaScript | .toFixed(0) | % |
Делимое |
| Lua | % |
Делитель | |
| Mathematica | Quotient
|
Mod |
Делитель |
| MATLAB | idivide(?, ?, 'floor') |
mod |
Делитель |
idivide |
rem |
Делимое | |
| MySQL | DIV |
MOD% |
Делимое |
| Oberon | DIV |
MOD |
+ |
| Objective Caml | mod |
Не определено | |
| Pascal | div |
mod |
Делимое[8] |
| Perl | Нет | % |
Делитель |
| PHP | Нет[9] | % |
Делимое |
| PL/I | mod |
Делитель (ANSI PL/I) | |
| Prolog (ISO 1995) | mod |
Делитель | |
| PureBasic | / |
Mod% |
Делимое |
| Python | // |
% |
Делитель |
| QBasic | |
MOD |
Делимое |
| R | %/% | %% |
Делитель |
| RPG | %REM |
Делимое | |
| Ruby | /
|
% |
Делитель |
| Scheme | modulo |
Делитель | |
| SenseTalk | modulo |
Делитель | |
rem |
Делимое | ||
| Tcl | % |
Делитель | |
| Verilog (2001) | % |
Делимое | |
| VHDL | mod |
Делитель | |
rem |
Делимое | ||
| Visual Basic | |
Mod |
Делимое |
Нахождение остатка от деления часто используется в компьютерной технике и телекоммуникационном оборудовании для создания контрольных чисел и получения случайных чисел в ограниченном диапазоне, например в конгруэнтном генераторе случайных чисел.
Обозначения операции взятия остатка в различных языках программирования представлены в таблице справа.
Например, в Паскале операция mod вычисляет остаток от деления, а операция div осуществляет целочисленное деление, при котором остаток от деления отбрасывается:
78 mod 33 = 12 78 div 33 = 2
Знак остатка[править | править код]
Операция взятия остатка в языках программирования может возвращать отрицательный результат (для отрицательного делимого или делителя). Тут есть два варианта:
- Знак остатка совпадает со знаком делимого: неполное частное округляет к нулю.
- Знак остатка совпадает со знаком делителя: неполное частное округляет к
.
Если в языке есть оба типа остатков, каждому из них соответствует своя операция неполного частного. Обе операции имеют жизненный смысл.
Операция div в x86/x64 делит регистровую пару rdx:rax на любой другой регистр или число из памяти[10]. Неполное частное и остаток выходят по первому варианту — округляют к нулю.
Как запрограммировать, если такой операции нет?[править | править код]
Неполное частное можно вычислить через деление и взятие целой части: ![q=left[{frac {a}{b}}right]](https://wikimedia.org/api/rest_v1/media/math/render/svg/8a5108d8efbb2b9259f9487fc6f9f7bb61aa7337)
![[x]](https://wikimedia.org/api/rest_v1/media/math/render/svg/07548563c21e128890501e14eb7c80ee2d6fda4d)
При отсутствии команды mod остаток программируется как 
Если 


Неполное частное и неотрицательный остаток от деления на степень двойки 


Обобщения[править | править код]
Вещественные числа[править | править код]
Если два числа 



Формально:
- если
, то
, где
.
- Пример
Деление 7,9 на 2,1 с остатком даёт:
(неполное частное);
(остаток).
Гауссовы целые числа[править | править код]
Гауссово число — это комплексное число вида 



,
где частное 

Однако, в отличие от целых чисел, остаток от деления определяется неоднозначно. Например, 

Многочлены[править | править код]
При делении с остатком двух многочленов 

, причём
.
- Пример
(остаток 3), так как:
.
См. также[править | править код]
- Алгоритм Евклида
- Делимость
- Наибольший общий делитель
- Непрерывная дробь
- Сравнение по модулю
Примечания[править | править код]
- ↑ 1 2 Деление // Математическая энциклопедия (в 5 томах). — М.: Советская Энциклопедия, 1979. — Т. 2.
- ↑ Потапов М. К., Александров В. В., Пасиченко П. И. Алгебра и анализ элементарных функций. М.: Наука, 1981, 560 с., С. 9.
- ↑ ISO/IEC 9899:TC2: When integers are divided, the result of the
/operator is the algebraic quotient with any fractional part discarded. [This is often called «truncation toward zero».]; в списке изменений 1999→TC1 и TC1→TC2 данное изменение не числится. - ↑ «ISO/IEC 14882:2003 : Programming languages — C++», 5.6.4: International Organization for Standardization, International Electrotechnical Commission, 2003. «the binary % operator yields the remainder from the division of the first expression by the second. …. If both operands are nonnegative then the remainder is nonnegative; if not, the sign of the remainder is implementation-defined».
- ↑ N3242=11-0012 (Working draft), текст совпадает с C99
- ↑ D language specification (англ.). dlang.org. Дата обращения: 29 октября 2017. Архивировано из оригинала 3 октября 2017 года.
- ↑ Арнолд, Кен, Гослинг, Дж., Холмс, Д. Язык программирования Java. — 3-е изд. — М., СПб., Киев: Вильямс, 2001. — С. 173—174. — ISBN 5-8459-0215-0.
- ↑ Стандарт 1973 года: div — division with truncation.
- ↑ PHP: Arithmetic Operators — Manual. Дата обращения: 27 ноября 2014. Архивировано 19 ноября 2014 года.
- ↑ DIV — Unsigned Divide
Быстрая задача на вычисление остатка от деления 13! на 17. Конечно, понятно, что остаток не может быть равен 0, ведь 17 – простое число и не представимо никаким образом как произведение множителей от 1 до 13.
Да, в компьютерный век вычислить “какой-то” 13! проще простого. Однако, я хочу рассказать Вам про метод, который позволит Вам делать вычисление остатков на бумаге. Поехали:
Операция “mod” – выдает остаток от деления числа на другое. Примечательно, что результат выполнения этой операции может быть и отрицательным (я буду часто использовать это). Итак, используем определение модулярной арифметики:
Теперь начинаем приводить множители в удобный для вычисления вид и получаем результат:
Просто постарайтесь не запутаться в вычислениях. Например, в третьей строчке я специально умножил 8,2 и 2, чтобы получить 32, которое по модулю 17 равно 15 и т.д. Как приводить множители – дело вкуса. В итоге получаем, что остаток от деления равен 3. Можете проверить на калькуляторе. Спасибо за внимание!
Читайте также:
Теги: sql, java, оператор, mod, запросы, таблицы, деление, operator, остаток
Оператор mod обозначается символом % и является оператором деления по модулю. Он возвращает остаток от деления 1-го операнда на 2-й и широко используется в разных языках программирования для решения ряда задач.
Оператор mod в Java
В Java operator mod может работать как с целыми числами (byte/int/short/long), так и с плавающей точкой (byte/int/short/long). Давайте приведём пример работы оператора mod при делении:
class Modulus { public static void main (String args []) { int x = 42; double у = 42.3; System.out.print("x mod 10 = " + x % 10); System.out.println("y mod 10 = " + у % 10); } }После выполнения этой программы вы получите результат следующего вида:
х mod 10 = 2 у mod 10 = 2.3Как видим, оператор mod — это прекрасный способ найти остаток от деления. Зачем это может понадобиться на практике? Например, при нахождении кратности числа и определении, является ли некоторое число, введённое с клавиатуры, чётным. Также с помощью оператора mod можно узнать, делится ли одно число на другое без остатка или определить последнюю цифру числа. В общем, оператор mod очень полезен при решении различных задач по программированию.
Оператор mod в SQL
Не менее интересно использование mod в базах данных. Аналогично, mod находит остаток от деления. При этом вместо mod можно задействовать операцию %, делающую то же самое. Синтаксис в SQL следующий:
SELECT MOD(что_делить, на_что_делить) FROM имя_таблицы WHERE условиеНо можно написать и иначе, используя %:
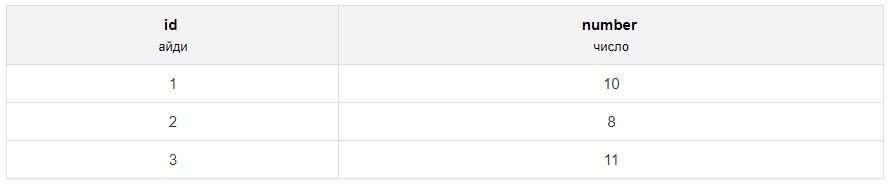
SELECT что_делить % на_что_делить FROM имя_таблицы WHERE условиеДавайте приведём пример использования mod в базах данных. Вот, например, таблица numbers:
Найдём остаток от деления столбца number на три:
SELECT *, MOD(number, 3) as mod FROM numbersВ результате запрос SQL выберет следующие строки:
Но, как мы уже говорили выше, этот же запрос можно без проблем переписать:
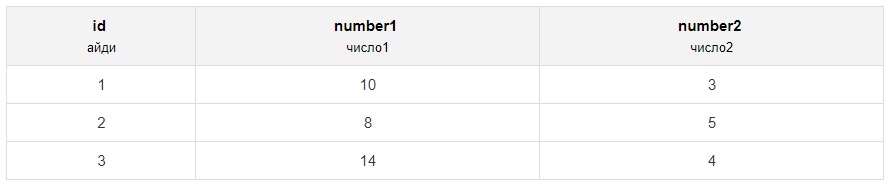
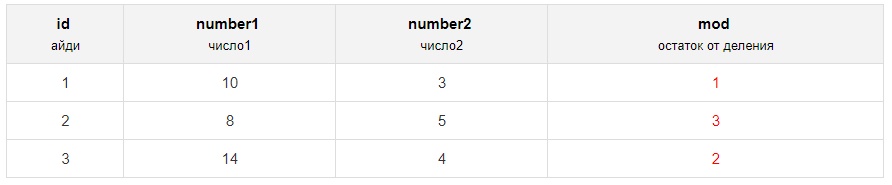
SELECT id, number % 3 as mod FROM numbersИдём дальше. Теперь возьмём таблицу посложнее:
Здесь найдём остаток от деления столбца number1 на number2:
SELECT *, MOD(number1, number2) as mod FROM numbersПолучим следующие строки:
Опять же, этот же самый запрос можно оформить иначе:
SELECT *, number1 % number2 as mod FROM numbersА где вы используете mod? Пишите в комментариях!
Время на прочтение
4 мин
Количество просмотров 229K

Приготовьтесь, вас ждёт крайне педантичная статья, которая вполне может спасти вас на собеседовании или сэкономить несколько часов при вылавливании бага в продакшне!
Я сейчас активно работаю над вторым сезоном «Руководства для самозванца» и пишу о шифре RSA для SSH, который, очевидно, является самым загружаемым фрагментом кода в истории IT.
Хочется полностью разобраться в этой истории. Кто придумал этот шифр, как он работает, почему работает и будет ли работать в будущем. Сейчас я раскопал одну чертовски интересную историю. Я не криптоманьяк и вижу, как других буквально засасывает в эту область. Но мне это тоже интересно, потому что повсюду есть маленькие норки, а меня как сороку привлекают блестящие штучки в глубоких норках. Я также очень хорош в метафорах.
В любом случае: на прошлой неделе я узнал что-то странное и хочу поделиться: оказывается, mod и остаток от деления — не одно и то же. Действительно забавно то, что некоторые читатели при этих словах выпрыгивают со своих кресел и орут: «А ведь именно это я всегда пытался сказать вам и всем остальным!»
Позовите ребят из секты «mod не остаток»! Это для вас.
Что такое mod?
Я должен был изучить это, как и в прошлый раз, когда всплыла такая тема. Это одна из тех вещей, которые ты знаешь, но не запоминаешь. Когда вы применяете mod, то делите одно число на другое и берёте остаток. Итак: 5 mod 2 будет 1, потому что 5/2=2 с остатком 1.
Термин mod означает операцию modulo, с модулем 2 в данном случае. Большинство языков программирования используют % для обозначения такой операции: 5 % 2 = 1.
Вот где мы попадаем в странную серую область.
Математика циферблата
Помню, как учил это в школе, а потом забыл. Существует тип математики, называемый «модульной арифметикой», которая имеет дело с циклическими структурами. Самый простой способ представить это — циферблат с циклом 12. Для математика циферблат — это mod 12. Если хотите понять, можно ли равномерно разделить 253 часа на дни, то можете применить операцию 253 mod 24, результатом будет 13, поэтому ответ «нет»! Мы можем ответить «да» только если результат 0.
Другой вопрос, который вы можете задать: «Если я выеду в 6 вечера, сколько времени будет по приезду через 16 часов?». Это будет 6 + 16 mod 12, то есть 10.
Криптографы любят mod, потому что при использовании с действительно большими числами можно создать нечто, известное как «односторонние функции». Это специальные функции, которые позволяют легко вычислить что-то в одном направлении, но не в обратном.
Если я скажу вам, что 9 является результатом возведения в квадрат, вы можете легко определить, что на входе было 3. Перед вами весь процесс от начала до конца. Если я скажу, что 9 является результатом mod 29, то будет сложнее понять, что на входе.
Криптографам нравится эта идея, потому что они могут использовать деление с остатком с гигантскими простыми числами для генерации криптографических ключей. Это совсем другая история: если хотите прочитать об этом, то можете купить книгу или, ещё лучше, поддержать мои усилия написать её.
Впрочем, не будем отклоняться от темы.
Остатки и математика циферблата
Теперь переходим к сути: modulo и простой остаток одинаковы, когда числа положительны, но отличаются в случае отрицательных чисел.
Рассмотрим такую задачу:
const x = 19 % 12;
console.log(x);
Каково значение x? Делим числа и получаем 7 как остаток от 12. Это верный ответ. Как насчет такого:
const y = 19 % -12;
console.log(y);Используя обычную математику, мы можем умножить -12 на -1, что даёт 12, и у нас по-прежнему остаётся 7, поэтому наш ответ снова 7.
JavaScript с этим согласен:

C# тоже согласен:
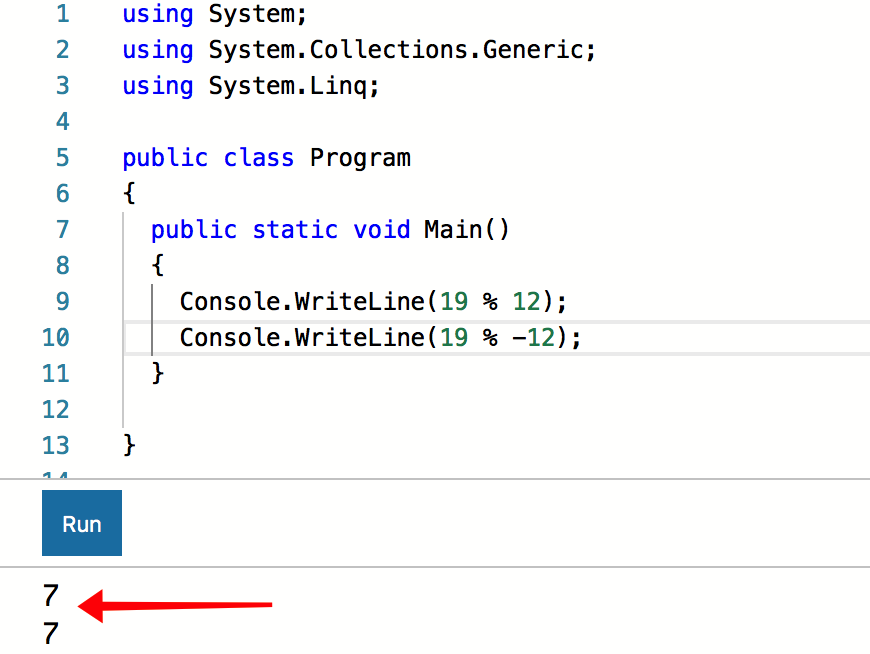
Google согласен с первым утверждением, но не согласен со вторым:
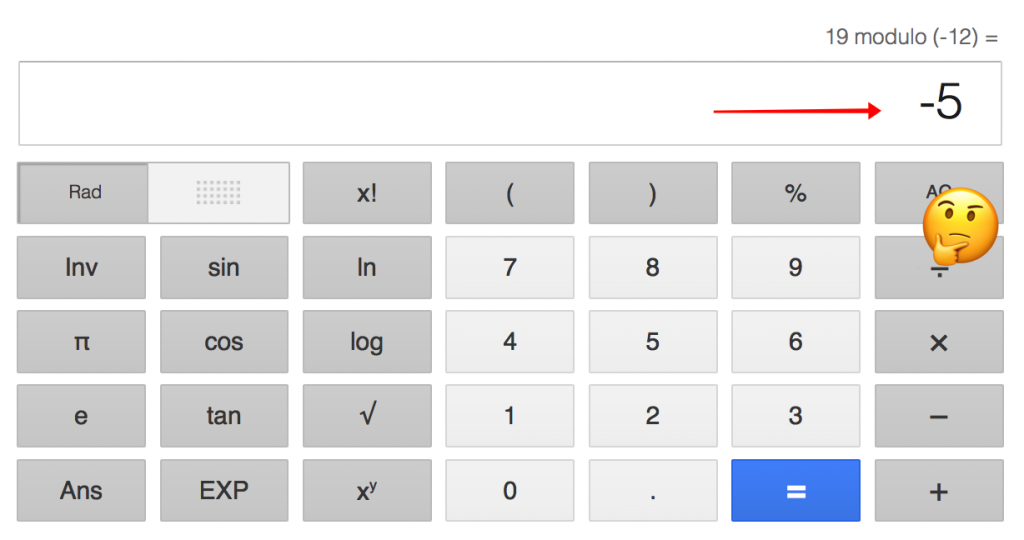
Ruby согласен с Google:

Во имя Дейкстры, что здесь происходит?
Вращение часов назад
Чтобы ответить на вопрос, следует понять разницу между остатком и modulo. Программисты объединяют эти операции, но не должны этого делать, потому что они дают одинаковый результат только в случае, если делитель (в нашем случае 12) положителен. Вы можете легко отправить баги в продакшн, если делитель отрицательный.
Но почему существует разница? Рассмотрим положительный делитель 19 mod 12 на часах:
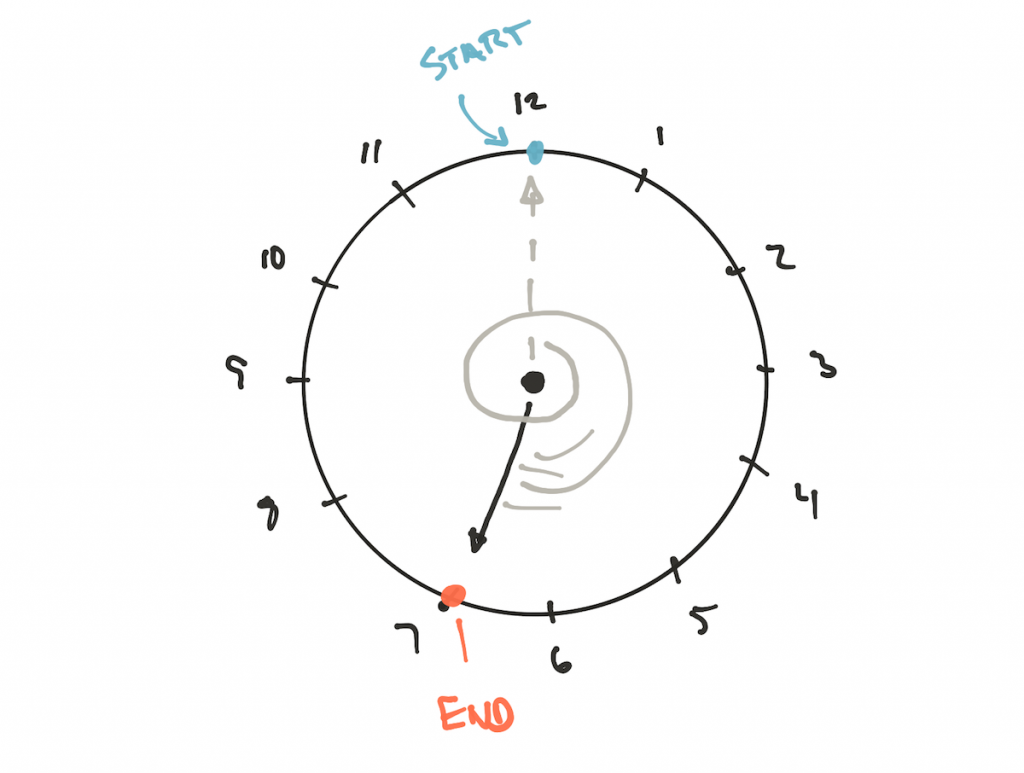
Конечный результат 7. Мы это знаем и мы можем доказать математически. Но что насчёт 19 mod -12? Здесь нужно использовать другие часы:
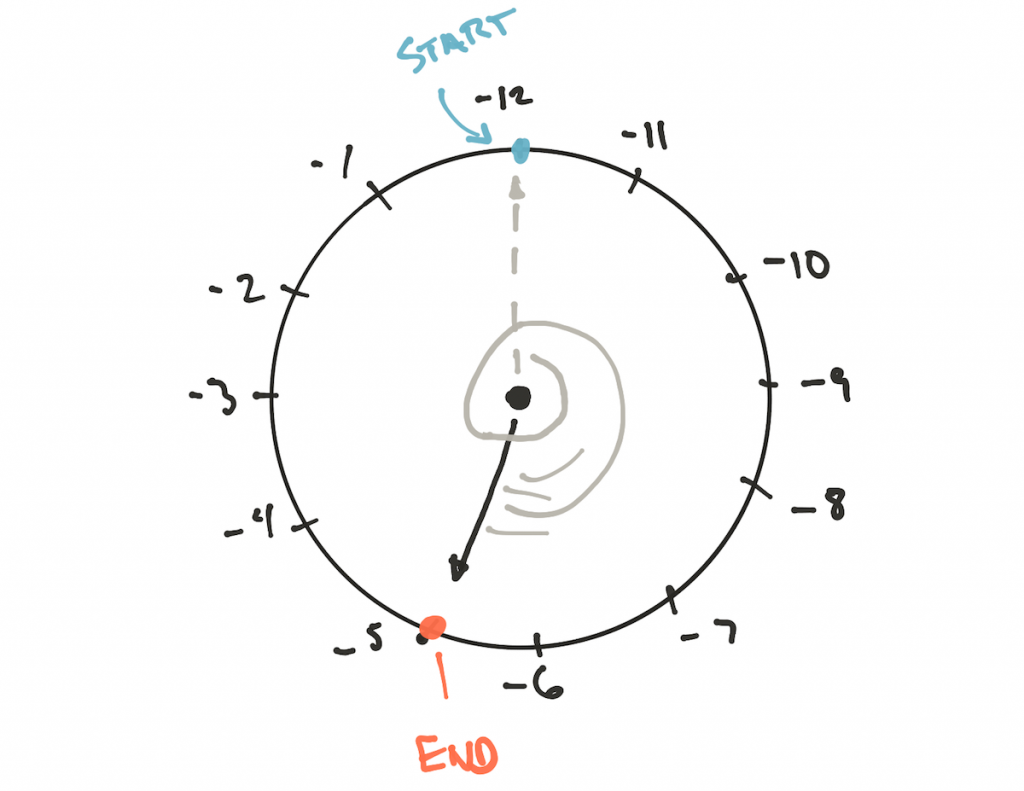
Модуль равен -12, и мы не можем игнорировать или изменить его, умножив на -1, поскольку модульная арифметика так не работает. Единственный способ правильно рассчитать результат — переставить метки на часах так, чтобы мы двигались от -12 или вращали часы против часовой стрелки, что даёт тот же результат.
Почему не начать метки с -1, двигаясь к -2, и т.д.? Потому что в таком случае мы будем двигаться назад и постоянно уменьшать результат, пока не достигнем -12, и в этот момент сделаем прыжок +12, а modulo так не работает.
Это известная вещь
Прежде чем назвать меня сумасшедшим и начать гуглить тему: это известный факт. На самом деле MDN (Mozilla Developer Network) даже дошла до того, чтобы назвать % операцией «остатка» (remainder), а не modulo:
Оператор remainder возвращает остаток от деления одного операнда на другой. Он всегда принимает знак делимого.
Вот что Эрик Липперт, один из богов C#, говорит о modulo в C#:
Однако это совсем не то, что оператор % реально делает в C#. Оператор % не является каноническим оператором modulus, это оператор остатка.
А как на вашем языке?
Ну и что?
Могу понять, если вы дочитали досюда, а теперь чешете голову и задаётесь вопросом, стоит ли беспокоиться. Думаю, что стоит по двум причинам:
- Я представляю, как этот вопрос займёт меня врасплох на собеседовании.
- Я представляю, как этот попадёт в продакшн, а разработчики будут несколько часов выяснять, почему математика не работает.
Это также забавный факт на случай, если рядом появится ваш педантичный друг-программист.
From Wikipedia, the free encyclopedia
In computing, the modulo operation returns the remainder or signed remainder of a division, after one number is divided by another (called the modulus of the operation).
Given two positive numbers a and n, a modulo n (often abbreviated as a mod n) is the remainder of the Euclidean division of a by n, where a is the dividend and n is the divisor.[1]
For example, the expression “5 mod 2” would evaluate to 1, because 5 divided by 2 has a quotient of 2 and a remainder of 1, while “9 mod 3” would evaluate to 0, because 9 divided by 3 has a quotient of 3 and a remainder of 0; there is nothing to subtract from 9 after multiplying 3 times 3.
Although typically performed with a and n both being integers, many computing systems now allow other types of numeric operands. The range of values for an integer modulo operation of n is 0 to n − 1 inclusive (a mod 1 is always 0; a mod 0 is undefined, possibly resulting in a division by zero error in some programming languages). See Modular arithmetic for an older and related convention applied in number theory.
When exactly one of a or n is negative, the naive definition breaks down, and programming languages differ in how these values are defined.
Variants of the definition[edit]
In mathematics, the result of the modulo operation is an equivalence class, and any member of the class may be chosen as representative; however, the usual representative is the least positive residue, the smallest non-negative integer that belongs to that class (i.e., the remainder of the Euclidean division).[2] However, other conventions are possible. Computers and calculators have various ways of storing and representing numbers; thus their definition of the modulo operation depends on the programming language or the underlying hardware.
In nearly all computing systems, the quotient q and the remainder r of a divided by n satisfy the following conditions:
-
(1)
However, this still leaves a sign ambiguity if the remainder is non-zero: two possible choices for the remainder occur, one negative and the other positive, and two possible choices for the quotient occur. In number theory, the positive remainder is always chosen, but in computing, programming languages choose depending on the language and the signs of a or n.[a] Standard Pascal and ALGOL 68, for example, give a positive remainder (or 0) even for negative divisors, and some programming languages, such as C90, leave it to the implementation when either of n or a is negative (see the table under § In programming languages for details). a modulo 0 is undefined in most systems, although some do define it as a.
-

Quotient (q) and
remainder (r) as functions of dividend (a), using truncated division
Many implementations use truncated division, for which the quotient is defined by
where [] is the integral part function (rounding toward zero), i.e. the truncation to zero significant digits.
Thus according to equation (1), the remainder has the same sign as the dividend: -

Quotient and remainder using floored division
Donald Knuth[3] promotes floored division, for which the quotient is defined by
where ⌊⌋ is the floor function (rounding down).
Thus according to equation (1), the remainder has the same sign as the divisor: -

Quotient and remainder using Euclidean division
Raymond T. Boute[4] promotes Euclidean division, for which the quotient is defined by
where sgn is the sign function, ⌊⌋ is the floor function (rounding down), and ⌈⌉ is the ceiling function (rounding up).
Thus according to equation (1), the remainder is non negative: -
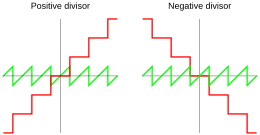
Quotient and remainder using rounded division
Common Lisp and IEEE 754 use rounded division, for which the quotient is defined by
where round is the round function (rounding half to even).
Thus according to equation (1), the remainder falls betweenand
, and its sign depends on which side of zero it falls to be within these boundaries:
-
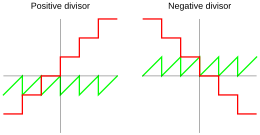
Quotient and remainder using ceiling division
Common Lisp also uses ceiling division, for which the quotient is defined by
where ⌈⌉ is the ceiling function (rounding up).
Thus according to equation (1), the remainder has the opposite sign of that of the divisor:
As described by Leijen,
Boute argues that Euclidean division is superior to the other ones in terms of regularity and useful mathematical properties, although floored division, promoted by Knuth, is also a good definition. Despite its widespread use, truncated division is shown to be inferior to the other definitions.
— Daan Leijen, Division and Modulus for Computer Scientists[5]
However, truncated division satisfies the identity 
Notation[edit]
This section is about the binary mod operation. For the (mod m) notation, see congruence relation.
Some calculators have a mod() function button, and many programming languages have a similar function, expressed as mod(a, n), for example. Some also support expressions that use “%”, “mod”, or “Mod” as a modulo or remainder operator, such as a % n or a mod n.
For environments lacking a similar function, any of the three definitions above can be used.
Common pitfalls[edit]
When the result of a modulo operation has the sign of the dividend (truncated definition), it can lead to surprising mistakes.
For example, to test if an integer is odd, one might be inclined to test if the remainder by 2 is equal to 1:
bool is_odd(int n) { return n % 2 == 1; }
But in a language where modulo has the sign of the dividend, that is incorrect, because when n (the dividend) is negative and odd, n mod 2 returns −1, and the function returns false.
One correct alternative is to test that the remainder is not 0 (because remainder 0 is the same regardless of the signs):
bool is_odd(int n) { return n % 2 != 0; }
Another alternative is to use the fact that for any odd number, the remainder may be either 1 or −1:
bool is_odd(int n) { return n % 2 == 1 || n % 2 == -1; }
A simpler alternative is to treat the result of n % 2 as if it is a boolean value, where any non-zero value is true:
bool is_odd(int n) { return n % 2; }
Performance issues[edit]
Modulo operations might be implemented such that a division with a remainder is calculated each time. For special cases, on some hardware, faster alternatives exist. For example, the modulo of powers of 2 can alternatively be expressed as a bitwise AND operation (assuming x is a positive integer, or using a non-truncating definition):
x % 2n == x & (2n - 1)
Examples:
x % 2 == x & 1x % 4 == x & 3x % 8 == x & 7
In devices and software that implement bitwise operations more efficiently than modulo, these alternative forms can result in faster calculations.[7]
Compiler optimizations may recognize expressions of the form expression % constant where constant is a power of two and automatically implement them as expression & (constant-1), allowing the programmer to write clearer code without compromising performance. This simple optimization is not possible for languages in which the result of the modulo operation has the sign of the dividend (including C), unless the dividend is of an unsigned integer type. This is because, if the dividend is negative, the modulo will be negative, whereas expression & (constant-1) will always be positive. For these languages, the equivalence x % 2n == x < 0 ? x | ~(2n - 1) : x & (2n - 1) has to be used instead, expressed using bitwise OR, NOT and AND operations.
Optimizations for general constant-modulus operations also exist by calculating the division first using the constant-divisor optimization.
Properties (identities)[edit]
Some modulo operations can be factored or expanded similarly to other mathematical operations. This may be useful in cryptography proofs, such as the Diffie–Hellman key exchange. Some of these properties require that a and n are integers.
- Identity:
- (a mod n) mod n = a mod n.
- nx mod n = 0 for all positive integer values of x.
- If p is a prime number which is not a divisor of b, then abp−1 mod p = a mod p, due to Fermat’s little theorem.
- Inverse:
- [(−a mod n) + (a mod n)] mod n = 0.
- b−1 mod n denotes the modular multiplicative inverse, which is defined if and only if b and n are relatively prime, which is the case when the left hand side is defined: [(b−1 mod n)(b mod n)] mod n = 1.
- Distributive:
- (a + b) mod n = [(a mod n) + (b mod n)] mod n.
- ab mod n = [(a mod n)(b mod n)] mod n.
- Division (definition): a/b mod n = [(a mod n)(b−1 mod n)] mod n, when the right hand side is defined (that is when b and n are coprime), and undefined otherwise.
- Inverse multiplication: [(ab mod n)(b−1 mod n)] mod n = a mod n.
In programming languages[edit]
| Language | Operator | Integer | Floating-point | Definition |
|---|---|---|---|---|
| ABAP | MOD
|
Yes | Yes | Euclidean |
| ActionScript | %
|
Yes | No | Truncated |
| Ada | mod
|
Yes | No | Floored[8] |
rem
|
Yes | No | Truncated[8] | |
| ALGOL 68 | ÷×, mod
|
Yes | No | Euclidean |
| AMPL | mod
|
Yes | No | Truncated |
| APL | |[b]
|
Yes | No | Floored |
| AppleScript | mod
|
Yes | No | Truncated |
| AutoLISP | (rem d n)
|
Yes | No | Truncated |
| AWK | %
|
Yes | No | Truncated |
| bash | %
|
Yes | No | Truncated |
| BASIC | Mod
|
Yes | No | Undefined |
| bc | %
|
Yes | No | Truncated |
| C C++ |
%, div
|
Yes | No | Truncated[c] |
fmod (C)std::fmod (C++)
|
No | Yes | Truncated[11] | |
remainder (C)std::remainder (C++)
|
No | Yes | Rounded | |
| C# | %
|
Yes | Yes | Truncated |
Math.IEEERemainder
|
No | Yes | Rounded[12] | |
| Clarion | %
|
Yes | No | Truncated |
| Clean | rem
|
Yes | No | Truncated |
| Clojure | mod
|
Yes | No | Floored[13] |
rem
|
Yes | No | Truncated[14] | |
| COBOL | FUNCTION MOD
|
Yes | No | Floored[d] |
| CoffeeScript | %
|
Yes | No | Truncated |
%%
|
Yes | No | Floored[15] | |
| ColdFusion | %, MOD
|
Yes | No | Truncated |
| Common Lisp | mod
|
Yes | Yes | Floored |
rem
|
Yes | Yes | Truncated | |
| Crystal | %, modulo
|
Yes | Yes | Floored |
remainder
|
Yes | Yes | Truncated | |
| D | %
|
Yes | Yes | Truncated[16] |
| Dart | %
|
Yes | Yes | Euclidean[17] |
remainder()
|
Yes | Yes | Truncated[18] | |
| Eiffel | \
|
Yes | No | Truncated |
| Elixir | rem/2
|
Yes | No | Truncated[19] |
Integer.mod/2
|
Yes | No | Floored[20] | |
| Elm | modBy
|
Yes | No | Floored[21] |
remainderBy
|
Yes | No | Truncated[22] | |
| Erlang | rem
|
Yes | No | Truncated |
math:fmod/2
|
No | Yes | Truncated (same as C)[23] | |
| Euphoria | mod
|
Yes | No | Floored |
remainder
|
Yes | No | Truncated | |
| F# | %
|
Yes | Yes | Truncated |
Math.IEEERemainder
|
No | Yes | Rounded[12] | |
| Factor | mod
|
Yes | No | Truncated |
| FileMaker | Mod
|
Yes | No | Floored |
| Forth | mod
|
Yes | No | Implementation defined |
fm/mod
|
Yes | No | Floored | |
sm/rem
|
Yes | No | Truncated | |
| Fortran | mod
|
Yes | Yes | Truncated |
modulo
|
Yes | Yes | Floored | |
| Frink | mod
|
Yes | No | Floored |
| GLSL | %
|
Yes | No | Undefined[24] |
mod
|
No | Yes | Floored[25] | |
| GameMaker Studio (GML) | mod, %
|
Yes | No | Truncated |
| GDScript (Godot) | %
|
Yes | No | Truncated |
fmod
|
No | Yes | Truncated | |
posmod
|
Yes | No | Floored | |
fposmod
|
No | Yes | Floored | |
| Go | %
|
Yes | No | Truncated[26] |
math.Mod
|
No | Yes | Truncated[27] | |
big.Int.Mod
|
Yes | No | Euclidean[28] | |
| Groovy | %
|
Yes | No | Truncated |
| Haskell | mod
|
Yes | No | Floored[29] |
rem
|
Yes | No | Truncated[29] | |
Data.Fixed.mod' (GHC)
|
No | Yes | Floored | |
| Haxe | %
|
Yes | No | Truncated |
| HLSL | %
|
Yes | Yes | Undefined[30] |
| J | |[b]
|
Yes | No | Floored |
| Java | %
|
Yes | Yes | Truncated |
Math.floorMod
|
Yes | No | Floored | |
| JavaScript TypeScript |
%
|
Yes | Yes | Truncated |
| Julia | mod
|
Yes | Yes | Floored[31] |
%, rem
|
Yes | Yes | Truncated[32] | |
| Kotlin | %, rem
|
Yes | Yes | Truncated[33] |
mod
|
Yes | Yes | Floored[34] | |
| ksh | %
|
Yes | No | Truncated (same as POSIX sh) |
fmod
|
No | Yes | Truncated | |
| LabVIEW | mod
|
Yes | Yes | Truncated |
| LibreOffice | =MOD()
|
Yes | No | Floored |
| Logo | MODULO
|
Yes | No | Floored |
REMAINDER
|
Yes | No | Truncated | |
| Lua 5 | %
|
Yes | Yes | Floored |
| Lua 4 | mod(x,y)
|
Yes | Yes | Truncated |
| Liberty BASIC | MOD
|
Yes | No | Truncated |
| Mathcad | mod(x,y)
|
Yes | No | Floored |
| Maple | e mod m (by default), modp(e, m)
|
Yes | No | Euclidean |
mods(e, m)
|
Yes | No | Rounded | |
frem(e, m)
|
Yes | Yes | Rounded | |
| Mathematica | Mod[a, b]
|
Yes | No | Floored |
| MATLAB | mod
|
Yes | No | Floored |
rem
|
Yes | No | Truncated | |
| Maxima | mod
|
Yes | No | Floored |
remainder
|
Yes | No | Truncated | |
| Maya Embedded Language | %
|
Yes | No | Truncated |
| Microsoft Excel | =MOD()
|
Yes | Yes | Floored |
| Minitab | MOD
|
Yes | No | Floored |
| Modula-2 | MOD
|
Yes | No | Floored |
REM
|
Yes | No | Truncated | |
| MUMPS | #
|
Yes | No | Floored |
| Netwide Assembler (NASM, NASMX) | %, div (unsigned)
|
Yes | No | — |
%% (signed)
|
Yes | No | Implementation-defined[35] | |
| Nim | mod
|
Yes | No | Truncated |
| Oberon | MOD
|
Yes | No | Floored-like[e] |
| Objective-C | %
|
Yes | No | Truncated (same as C99) |
| Object Pascal, Delphi | mod
|
Yes | No | Truncated |
| OCaml | mod
|
Yes | No | Truncated[36] |
mod_float
|
No | Yes | Truncated[37] | |
| Occam | |
Yes | No | Truncated |
| Pascal (ISO-7185 and -10206) | mod
|
Yes | No | Euclidean-like[f] |
| Programming Code Advanced (PCA) | |
Yes | No | Undefined |
| Perl | %
|
Yes | No | Floored[g] |
POSIX::fmod
|
No | Yes | Truncated | |
| Phix | mod
|
Yes | No | Floored |
remainder
|
Yes | No | Truncated | |
| PHP | %
|
Yes | No | Truncated[39] |
fmod
|
No | Yes | Truncated[40] | |
| PIC BASIC Pro | \
|
Yes | No | Truncated |
| PL/I | mod
|
Yes | No | Floored (ANSI PL/I) |
| PowerShell | %
|
Yes | No | Truncated |
| Programming Code (PRC) | MATH.OP - 'MOD; ()'
|
Yes | No | Undefined |
| Progress | modulo
|
Yes | No | Truncated |
| Prolog (ISO 1995) | mod
|
Yes | No | Floored |
rem
|
Yes | No | Truncated | |
| PureBasic | %, Mod(x,y)
|
Yes | No | Truncated |
| PureScript | `mod`
|
Yes | No | Euclidean[41] |
| Pure Data | %
|
Yes | No | Truncated (same as C) |
mod
|
Yes | No | Floored | |
| Python | %
|
Yes | Yes | Floored |
math.fmod
|
No | Yes | Truncated | |
| Q# | %
|
Yes | No | Truncated[42] |
| R | %%
|
Yes | Yes | Floored[43] |
| Racket | modulo
|
Yes | No | Floored |
remainder
|
Yes | No | Truncated | |
| Raku | %
|
No | Yes | Floored |
| RealBasic | MOD
|
Yes | No | Truncated |
| Reason | mod
|
Yes | No | Truncated |
| Rexx | //
|
Yes | Yes | Truncated |
| RPG | %REM
|
Yes | No | Truncated |
| Ruby | %, modulo()
|
Yes | Yes | Floored |
remainder()
|
Yes | Yes | Truncated | |
| Rust | %
|
Yes | Yes | Truncated |
rem_euclid()
|
Yes | Yes | Euclidean[44] | |
| SAS | MOD
|
Yes | No | Truncated |
| Scala | %
|
Yes | No | Truncated |
| Scheme | modulo
|
Yes | No | Floored |
remainder
|
Yes | No | Truncated | |
| Scheme R6RS | mod
|
Yes | No | Euclidean[45] |
mod0
|
Yes | No | Rounded[45] | |
flmod
|
No | Yes | Euclidean | |
flmod0
|
No | Yes | Rounded | |
| Scratch | mod
|
Yes | Yes | Floored |
| Seed7 | mod
|
Yes | Yes | Floored |
rem
|
Yes | Yes | Truncated | |
| SenseTalk | modulo
|
Yes | No | Floored |
rem
|
Yes | No | Truncated | |
sh (POSIX) (includes bash, mksh, &c.)
|
%
|
Yes | No | Truncated (same as C)[46] |
| Smalltalk | \
|
Yes | No | Floored |
rem:
|
Yes | No | Truncated | |
| Snap! | mod
|
Yes | No | Floored |
| Spin | //
|
Yes | No | Floored |
| Solidity | %
|
Yes | No | Floored |
| SQL (SQL:1999) | mod(x,y)
|
Yes | No | Truncated |
| SQL (SQL:2011) | %
|
Yes | No | Truncated |
| Standard ML | mod
|
Yes | No | Floored |
Int.rem
|
Yes | No | Truncated | |
Real.rem
|
No | Yes | Truncated | |
| Stata | mod(x,y)
|
Yes | No | Euclidean |
| Swift | %
|
Yes | No | Truncated[47] |
remainder(dividingBy:)
|
No | Yes | Rounded[48] | |
truncatingRemainder(dividingBy:)
|
No | Yes | Truncated[49] | |
| Tcl | %
|
Yes | No | Floored |
| tcsh | %
|
Yes | No | Truncated |
| Torque | %
|
Yes | No | Truncated |
| Turing | mod
|
Yes | No | Floored |
| Verilog (2001) | %
|
Yes | No | Truncated |
| VHDL | mod
|
Yes | No | Floored |
rem
|
Yes | No | Truncated | |
| VimL | %
|
Yes | No | Truncated |
| Visual Basic | Mod
|
Yes | No | Truncated |
| WebAssembly | i32.rem_u, i64.rem_u (unsigned)
|
Yes | No | —[50] |
i32.rem_s, i64.rem_s (signed)
|
Yes | No | Truncated[51] | |
| x86 assembly | IDIV
|
Yes | No | Truncated |
| XBase++ | %
|
Yes | Yes | Truncated |
Mod()
|
Yes | Yes | Floored | |
| Zig | %,
|
Yes | Yes | Truncated[52] |
| Z3 theorem prover | div, mod
|
Yes | No | Euclidean |
In addition, many computer systems provide a divmod functionality, which produces the quotient and the remainder at the same time. Examples include the x86 architecture’s IDIV instruction, the C programming language’s div() function, and Python’s divmod() function.
Generalizations[edit]
Modulo with offset[edit]
Sometimes it is useful for the result of a modulo n to lie not between 0 and n − 1, but between some number d and d + n − 1. In that case, d is called an offset. There does not seem to be a standard notation for this operation, so let us tentatively use a modd n. We thus have the following definition:[53] x = a modd n just in case d ≤ x ≤ d + n − 1 and x mod n = a mod n. Clearly, the usual modulo operation corresponds to zero offset: a mod n = a mod0 n. The operation of modulo with offset is related to the floor function as follows:
(To see this, let 




The modulo with offset a modd n is implemented in Mathematica as Mod[a, n, d] .[53]
Implementing other modulo definitions using truncation[edit]
Despite the mathematical elegance of Knuth’s floored division and Euclidean division, it is generally much more common to find a truncated division-based modulo in programming languages. Leijen provides the following algorithms for calculating the two divisions given a truncated integer division:[5]
/* Euclidean and Floored divmod, in the style of C's ldiv() */ typedef struct { /* This structure is part of the C stdlib.h, but is reproduced here for clarity */ long int quot; long int rem; } ldiv_t; /* Euclidean division */ inline ldiv_t ldivE(long numer, long denom) { /* The C99 and C++11 languages define both of these as truncating. */ long q = numer / denom; long r = numer % denom; if (r < 0) { if (denom > 0) { q = q - 1; r = r + denom; } else { q = q + 1; r = r - denom; } } return (ldiv_t){.quot = q, .rem = r}; } /* Floored division */ inline ldiv_t ldivF(long numer, long denom) { long q = numer / denom; long r = numer % denom; if ((r > 0 && denom < 0) || (r < 0 && denom > 0)) { q = q - 1; r = r + denom; } return (ldiv_t){.quot = q, .rem = r}; }
For both cases, the remainder can be calculated independently of the quotient, but not vice versa. The operations are combined here to save screen space, as the logical branches are the same.
See also[edit]
- Modulo (disambiguation) – many uses of the word modulo, all of which grew out of Carl F. Gauss’s introduction of modular arithmetic in 1801.
- Modulo (mathematics), general use of the term in mathematics
- Modular exponentiation
- Turn (angle)
Notes[edit]
- ^ Mathematically, these two choices are but two of the infinite number of choices available for the inequality satisfied by a remainder.
- ^ a b Argument order reverses, i.e.,
α|ωcomputes, the remainder when dividing
ωbyα. - ^ C99 and C++11 define the behavior of
%to be truncated.[9] The standards before then leave the behavior implementation-defined.[10] - ^ As implemented in ACUCOBOL, Micro Focus COBOL, and possible others.
- ^ Divisor must be positive, otherwise undefined.
- ^ As discussed by Boute, ISO Pascal’s definitions of
divandmoddo not obey the Division Identity of D = d · (D / d) + D % d, and are thus fundamentally broken. - ^ Perl usually uses arithmetic modulo operator that is machine-independent. For examples and exceptions, see the Perl documentation on multiplicative operators.[38]
References[edit]
- ^ Weisstein, Eric W. “Congruence”. mathworld.wolfram.com. Retrieved 2020-08-27.
- ^ Caldwell, Chris. “residue”. Prime Glossary. Retrieved August 27, 2020.
- ^ Knuth, Donald. E. (1972). The Art of Computer Programming. Addison-Wesley.
- ^ Boute, Raymond T. (April 1992). “The Euclidean definition of the functions div and mod”. ACM Transactions on Programming Languages and Systems. ACM Press (New York, NY, USA). 14 (2): 127–144. doi:10.1145/128861.128862. hdl:1854/LU-314490. S2CID 8321674.
- ^ a b Leijen, Daan (December 3, 2001). “Division and Modulus for Computer Scientists” (PDF). Retrieved 2014-12-25.
- ^ Peterson, Doctor (5 July 2001). “Mod Function and Negative Numbers”. Math Forum – Ask Dr. Math. Archived from the original on 2019-10-22. Retrieved 22 October 2019.
- ^ Horvath, Adam (July 5, 2012). “Faster division and modulo operation – the power of two”.
- ^ a b “ISO/IEC 8652:2012 – Information technology — Programming languages — Ada”. ISO, IEC. 2012. sec. 4.5.5 Multiplying Operators.
- ^ “C99 specification (ISO/IEC 9899:TC2)” (PDF). 2005-05-06. sec. 6.5.5 Multiplicative operators. Retrieved 16 August 2018.
- ^ “ISO/IEC 14882:2003: Programming languages – C++”. International Organization for Standardization (ISO), International Electrotechnical Commission (IEC). 2003. sec. 5.6.4.
the binary % operator yields the remainder from the division of the first expression by the second. …. If both operands are nonnegative then the remainder is nonnegative; if not, the sign of the remainder is implementation-defined
- ^ “ISO/IEC 9899:1990: Programming languages – C”. ISO, IEC. 1990. sec. 7.5.6.4.
The fmod function returns the value x – i * y, for some integer i such that, if y is nonzero, the result has the same sign as x and magnitude less than the magnitude of y.
- ^ a b dotnet-bot. “Math.IEEERemainder(Double, Double) Method (System)”. learn.microsoft.com. Retrieved 2022-10-04.
- ^ “clojure.core – Clojure v1.10.3 API documentation”. clojure.github.io. Retrieved 2022-03-16.
- ^ “clojure.core – Clojure v1.10.3 API documentation”. clojure.github.io. Retrieved 2022-03-16.
- ^ CoffeeScript operators
- ^ “Expressions – D Programming Language”. dlang.org. Retrieved 2021-06-01.
- ^ “operator % method – num class – dart:core library – Dart API”. api.dart.dev. Retrieved 2021-06-01.
- ^ “remainder method – num class – dart:core library – Dart API”. api.dart.dev. Retrieved 2021-06-01.
- ^ “Kernel — Elixir v1.11.3”. hexdocs.pm. Retrieved 2021-01-28.
- ^ “Integer — Elixir v1.11.3”. hexdocs.pm. Retrieved 2021-01-28.
- ^ “Basics – core 1.0.5”. package.elm-lang.org. Retrieved 2022-03-16.
- ^ “Basics – core 1.0.5”. package.elm-lang.org. Retrieved 2022-03-16.
- ^ “Erlang — math”. erlang.org. Retrieved 2021-06-01.
- ^ “GLSL Language Specification, Version 4.50.7” (PDF). section 5.9 Expressions.
If both operands are non-negative, then the remainder is non-negative. Results are undefined if one or both operands are negative.
- ^ “GLSL Language Specification, Version 4.50.7” (PDF). section 8.3 Common Functions.
- ^ “The Go Programming Language Specification – The Go Programming Language”. go.dev. Retrieved 2022-02-28.
- ^ “math package – math – pkg.go.dev”. pkg.go.dev. Retrieved 2022-02-28.
- ^ “big package – math/big – pkg.go.dev”. pkg.go.dev. Retrieved 2022-02-28.
- ^ a b “6 Predefined Types and Classes”. www.haskell.org. Retrieved 2022-05-22.
- ^ “Operators”. Microsoft. Retrieved 2021-07-19.
The % operator is defined only in cases where either both sides are positive or both sides are negative. Unlike C, it also operates on floating-point data types, as well as integers.
- ^ “Mathematics · The Julia Language”. docs.julialang.org. Retrieved 2021-11-20.
- ^ “Mathematics · The Julia Language”. docs.julialang.org. Retrieved 2021-11-20.
- ^ “rem – Kotlin Programming Language”. Kotlin. Retrieved 2021-05-05.
- ^ “mod – Kotlin Programming Language”. Kotlin. Retrieved 2021-05-05.
- ^ “Chapter 3: The NASM Language”. NASM – The Netwide Assembler version 2.15.05.
- ^ “OCaml library : Stdlib”. ocaml.org. Retrieved 2022-02-19.
- ^ “OCaml library : Stdlib”. ocaml.org. Retrieved 2022-02-19.
- ^ Perl documentation
- ^ “PHP: Arithmetic Operators – Manual”. www.php.net. Retrieved 2021-11-20.
- ^ “PHP: fmod – Manual”. www.php.net. Retrieved 2021-11-20.
- ^ “EuclideanRing”.
- ^ QuantumWriter. “Expressions”. docs.microsoft.com. Retrieved 2018-07-11.
- ^ “R: Arithmetic Operators”. search.r-project.org. Retrieved 2022-12-24.
- ^ “F32 – Rust”.
- ^ a b r6rs.org
- ^ “Shell Command Language”. pubs.opengroup.org. Retrieved 2021-02-05.
- ^ “Apple Developer Documentation”. developer.apple.com. Retrieved 2021-11-20.
- ^ “Apple Developer Documentation”. developer.apple.com. Retrieved 2021-11-20.
- ^ “Apple Developer Documentation”. developer.apple.com. Retrieved 2021-11-20.
- ^ “Numerics — WebAssembly 1.1 (Draft 2022-03-02)”. webassembly.github.io. Retrieved 2022-03-16.
- ^ “Numerics — WebAssembly 1.1 (Draft 2022-03-02)”. webassembly.github.io. Retrieved 2022-03-16.
- ^ “Zig Documentation”. Zig Programming Language. Retrieved 2022-12-18.
- ^ a b “Mod”. Wolfram Language & System Documentation Center. Wolfram Research. 2020. Retrieved April 8, 2020.
External links[edit]
- Modulorama, animation of a cyclic representation of multiplication tables (explanation in French)











![{displaystyle q=left[{frac {a}{n}}right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/85f537bcf683b9359bc7c937287b3095c9ae6d15)
![{displaystyle r=a-nleft[{frac {a}{n}}right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0be02e2b4b3fd15eb8415bc7a03ead062bb6fc28)









