Загрузить PDF
Загрузить PDF
Найти третий угол треугольника, если вам известны значения двух других углов, очень легко. Все, что вам нужно сделать,- это вычесть сумму двух известных углов из 180°. Тем не менее, есть несколько других способов нахождения третьего угла треугольника (в зависимости от заданной вам задачи).
-

1
Сложите известные значения двух углов. Запомните: сумма углов в треугольнике всегда равна 180°. Поэтому, если вы знаете два из трех углов треугольника, то вы легко вычислите третий угол. Первое, что нужно сделать,- это сложить известные значения двух углов. Например, даны углы 80° и 65°. Сложите их: 80° + 65° = 145°.
-

2
Вычтите сумму из 180°. Сумма углов в треугольнике равна 180°. Поэтому третий угол равен: 180° – 145° = 35°.
-

3
Запишите ответ. Теперь вы знаете, что третий угол равен 35°. Если вы сомневаетесь, просто проверьте ответ. Сумма трех углов должна быть равна 180°: 80° + 65° + 35° = 180°.
Реклама
-

1
Запишите задачу. Иногда вместо точных значений двух углов треугольника в задаче даны только несколько переменных, или переменные и значение угла. Например: найдите угол «х», если два других угла треугольника равны 2x и 24°.
-

2
Сложите все значения (переменные и числа). х + 2x + 24° = 3x + 24
-

3
Вычтите сумму из 180°. Приравняйте полученное уравнение к 0. Вот как это делается:
- 180° – (3x + 24°) = 0
- 180° – 3x – 24° = 0
- 156° – 3x = 0
-

4
Найдите х. Для этого обособьте члены с переменной на одной стороне уравнения, а числа – на другой: 156° = 3x. Теперь разделите обе части уравнения на 3, чтобы получить х = 52°. Это означает, что третий угол треугольника равен 52°. Другой угол, данный в условии как 2x, равен: 2*52° = 104°.
-

5
Проверьте ответ. Для этого сложите числовые значения всех трех углов (сумма должна быть равна 180°): 52° + 104° + 24° = 180°.
Реклама
-

1
Найдите третий угол равнобедренного треугольника. Равнобедренные треугольники имеют две равные стороны и два равных угла, прилежащих к этим сторонам. Если вы знаете один из равных углов в равнобедренном треугольнике, то вы можете найти угол между равными сторонами. Вот как это сделать:
- Если один из равных углов 40°, то и другой равный угол 40°. Вы можете найти третий угол, вычтя сумму 40° + 40° = 80° из 180°: 180° – 80° = 100°.
-

2
Найдите третий угол равностороннего треугольника. В равностороннем треугольнике все стороны равны и все углы равны. Это означает, что любой угол в равностороннем треугольнике равен 60°. Проверьте это: 60° + 60° + 60° = 180°.
-

3
Найдите третий угол прямоугольного треугольника. Например, дан прямоугольный треугольник, в котором один из углов равен 30°. Если это прямоугольный треугольник, то один из его углов равен 90°. Все, что вам нужно сделать, это сложить известные углы (30° + 90° = 120°) и вычесть эту сумму из 180°, то есть 180° – 120° = 60°. Третий угол равен 60°.
Реклама
Предупреждения
- Ошибка при сложении или вычитании приведет к неправильному ответу. Поэтому обязательно проверяйте ответ, даже когда вы уверены, что он правильный.
Реклама
Об этой статье
Эту страницу просматривали 82 823 раза.
Была ли эта статья полезной?
Download Article
Download Article
Finding the third angle of a triangle when you know the measurements of the other two angles is easy. All you’ve got to do is subtract the other angle measurements from 180° to get the measurement of the third angle. However, there are a few other ways to find the measurement of the third angle of a triangle, depending on the problem you’re working with. If you want to know how to find that elusive third angle of a triangle, see Step 1 to get started.
-

1
Add up the two known angle measurements. All you have to know is that all of the angles in a triangle always add up to 180°. This is true 100% of the time. So, if you know two of the three measurements of the triangle, then you’re only missing one piece of the puzzle. The first thing you can do is add up the angle measurements you know. In this example, the two angle measurements you know are 80° and 65°. Add them up (80° + 65°) to get 145°.[1]
-

2
Subtract this number from 180°. The angles in a triangle add up to 180°. Therefore, the remaining angle must make the sum up the angles up to 180°. In this example, 180° – 145° = 35°.[2]
Advertisement
-

3
Write down your answer. You now know that the third angle measures 35°. If you’re doubting yourself, just check your work. The three angles should add up to 180° for the triangle to exist. 80° + 65° + 35° = 180°. You’re all done.[3]
Advertisement
-

1
Write down the problem. Sometimes, instead being lucky enough to know the measurements of two of the angles of a triangle, you’ll only be given a few variables, or some variables and an angle measurement. Let’s say you’re working with this problem: Find the measurements of angle “x” of the triangle whose measurements are “x,” “2x,” and 24. First, just write it down.[4]
-

2
Add up all of the measurements. It’s the same principle that you would follow if you did know the measurements of the two angles. Simply add up the measurements of the angles, combining the variables. So, x + 2x + 24° = 3x + 24°.[5]
-

3
Subtract the measurements from 180°. Now, subtract these measurements from 180° to get closer to solving the problem. Make sure you set the equation equal to 0. Here’s what it would look like:
- 180° – (3x + 24°) = 0
- 180° – 3x – 24° = 0
- 156° – 3x = 0
-

4
Solve for x. Now, just put the variables on one side of the equation and the numbers on the other side. You’ll get 156° = 3x. Now, divide both sides of the equation by 3 to get x = 52°. This means that the measurement of the third angle of the triangle is 52°. The other angle, 2x, is 2 x 52°, or 104°.[6]
-

5
Check your work. If you want to make sure that this is a valid triangle, just add up the three angle measurements to make sure that they add up to 180°. That’s 52° + 104° + 24° = 180°. You’re all done.
Advertisement
-

1
Find the third angle of an isosceles triangle. Isosceles triangles have two equal sides and two equal angles. The equal sides are marked by one hash mark on each of them, indicating that the angles across from each side are equal. If you know the angle measurement of one equal angle of an isosceles triangle, then you’ll know the measurement of the other equal angle. Here’s how to find it:[7]
- If one of the equal angles is 40°, then you’ll know that the other angle is also 40°. You can find the third side, if needed, by subtracting 40° + 40° (which is 80°) from 180°. 180° – 80° = 100°, which is the measurement of the remaining angle.
-

2
Find the third angle of an equilateral triangle. An equilateral triangle has all equal sides and all equal angles. It will typically be marked by two hash marks in the middle of each of its sides. This means that the angle measurement of any angle in an equilateral triangle is 60°. Check your work. 60° + 60° + 60° = 180°.[8]
-

3
Find the third angle of a right triangle. Let’s say you know you have a right triangle, with one of the other angles being 30°. If it’s a right triangle, then you know that one of the angles measures exactly 90°. The same principles apply. All you have to do is add up the measurements of the sides you know (30° + 90° = 120°) and subtract that number from 180°. So, 180° – 120° = 60°. The measurement of that third angle is 60°.[9]
Advertisement
Add New Question
-
Question
In a right angle triangle, if one of the other two angles is 35 degrees, find the remaining angle.

Take the 90, add it to 35. This gives you 125 degrees. Triangles can only ever add up to 180, thus take the difference of 125 and 180 (180-125). This will give you the third remaining angle, which in this case is 55.
-
Question
If one angle of a right triangle is 50 degrees, what will be the measurement of the third angle?

A right angle triangle always consists of one 90 degree angle, and every triangle must equal 180 degrees. Here is the work for this problem: 90 degrees (representing the right angle) + 50 degrees equals 140 degrees. 180 minus 140 equals 40. Therefore, the remaining angle would be 40 degrees.
-
Question
What if there is only one number?

Substitute a letter, and work it out like an algebraic equation that you have to solve.
See more answers
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
Advertisement
Video
Thanks for submitting a tip for review!
-
Making a mistake with addition and subtraction will result in a wrong answer. It’s always a good idea to check, even if it doesn’t appear to be wrong.
Advertisement
References
About This Article
Article SummaryX
To find the third angle of a triangle, start by adding the other 2 angles together. Then, subtract that number from 180 to find the third angle. If the 2 known angles have variables, start by adding all of the measurements, including the variable used for the unknown angle. Then, subtract those numbers and variables from 180 and set the equation equal to 0. Finally, solve for the variable to find the third angle. If you want to learn the angles are on specific kinds of triangles, keep reading the article!
Did this summary help you?
Thanks to all authors for creating a page that has been read 262,790 times.
Did this article help you?
Решение треугольников онлайн
С помощю этого онлайн калькулятора можно решить треугольники, т.е. найти неизвестные элементы (стороны, углы) треугольника. Теоретическую часть и численные примеры смотрите ниже.
Решение треугольников − это нахождение всех его элементов (трех сторон и трех углов) по трем известным элементам (сторонам и углам). В статье Треугольники. Признаки равенства треугольников рассматриваются условия, при которых два треугольника оказываются равными друг друга. Как следует из статьи, треугольник однозначно определяется тремя элементами. Это:
- Три стороны треугольника.
- Две стороны треугольника и угол между ними.
- Две стороны и угол противостоящий к одному из этих сторон треугольника.
- Одна сторона и любые два угла.
Заметим, что если у треугольника известны два угла, то легко найти третий угол, т.к. сумма всех углов треугольника равна 180°.
Решение треугольника по трем сторонам
Пусть известны три стороны треугольника a, b, c (Рис.1). Найдем 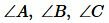 .
.
Решение:
Из теоремы косинусов имеем:
Откуда
Из (1) и (2) находим cosA, cosB и углы A и B (используя калькулятор). Далее, угол C находим из выражения
Пример 1. Известны стороны треугольника ABC: 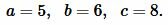 Найти
Найти 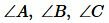 (Рис.1).
(Рис.1).
Решение. Из формул (1) и (2) находим:
Используя онлайн калькулятор для arcsin и arccos находим углы A и B:
И, наконец, находим угол C:
Решение треугольника по двум сторонам и углу между ними
Пусть известны стороны треугольника a и b и угол между ними C (Рис.2). Найдем сторону c и углы A и B.
Решение:
Найдем сторону c используя теорему косинусов:
Далее, из формулы
найдем cosA:
Далее из (3) с помощью калькулятора находим угол A.
Поскольку уже нам известны два угла то находим третий:
Пример 2. Известны две стороны треугольника ABC: 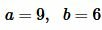 и
и 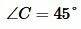 (Рис.2). Найти сторону c и углы A и B.
(Рис.2). Найти сторону c и углы A и B.
Решение. Иcпользуя теорму косинусов найдем сторону c:
Вычисления выше легко производить инженерным онлайн калькулятором.
Из формулы (3) найдем cosA:
Используя онлайн калькулятор для arcsin и arccos или инженерный онлайн калькулятор находим угол A:
Поскольку уже нам известны два угла то находим третий:
Решение треугольника по стороне и любым двум углам
Пусть известна сторона треугольника a и углы A и B (Рис.4). Найдем стороны b и c и угол C.
Решение:
Так как, уже известны два угла, то можно найти третий:
Далее, для находждения сторон b и c воспользуемся тероемой синусов:
Откуда
Пример 3. Известна одна сторона треугольника ABC: 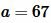 и углы
и углы 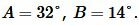 (Рис.3). Найти стороны b и c и угол С.
(Рис.3). Найти стороны b и c и угол С.
Решение. Поскольку известны два угла, то легко можно найти третий угол С:
Найдем сторону b. Из теоремы синусов имеем:
Найдем сторону с. Из теоремы синусов имеем:
Ответ:
Эль А
Гуру
(4568),
закрыт
11 лет назад
Как найти 3ий угол треугольника, зная его 2 других угла? Если есть какая-то формула, подскажите, пожалуйста! Заранее спасибо!
Лучший ответ
Елена Хусаинова
Мудрец
(14191)
11 лет назад
сумма всех трех углов=180 градусов
угол3=180-угол1-угол2
Остальные ответы
Наталия Байдакова
Знаток
(400)
11 лет назад
сумма углов треугольника – 180
простым вычитанием и находится=)
Nebelyng
Мыслитель
(7613)
11 лет назад
Сумма углов 180 градусов, вычти два известных
злато-серебро
Оракул
(87902)
11 лет назад
Полезно запомнить теорему:
Сумма углов n-угольника равна 180°(n-2), где n – количество сторон.
В треугольнике сторон три. 180° (3-2)=180°
В четырехугольнике сторон четыре. 180°(4-2)=360. И так далее.. .
