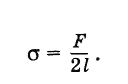Текущая версия страницы пока не проверялась опытными участниками и может значительно отличаться от версии, проверенной 11 января 2023 года; проверки требуют 3 правки.
Монета, лежащая на поверхности воды благодаря силе поверхностного натяжения
Когезия формирует водяные капли, поверхностное натяжение делает их почти сферическими, а адгезия держит их на поверхности другого вещества
| Механика сплошных сред |
|---|
 |
| Сплошная среда |
|
Классическая механика Закон сохранения массы · Закон сохранения импульса |
|
Теория упругости Напряжение · Тензор · Твёрдые тела · Упругость · Пластичность · Закон Гука · Реология · Вязкоупругость |
|
Гидродинамика Жидкость · Гидростатика · Гидродинамика · Вязкость · Ньютоновская жидкость · Неньютоновская жидкость · Поверхностное натяжение |
|
Основные уравнения Уравнение непрерывности · Уравнение Эйлера · Уравнение Громеки — Лэмба · Уравнение Бернулли · Уравнения Навье — Стокса · Уравнение вихря · Уравнение диффузии · Закон Гука |
| См. также: Портал:Физика |
Пове́рхностное натяже́ние — термодинамическая характеристика поверхности раздела двух находящихся в равновесии фаз, определяемая работой обратимого изотермокинетического образования единицы площади этой поверхности раздела при условии, что температура, объём системы и химические потенциалы всех компонентов в обеих фазах остаются постоянными.
Поверхностное натяжение имеет двойной физический смысл — энергетический (термодинамический) и силовой (механический). Энергетическое (термодинамическое) определение: поверхностное натяжение — это удельная работа увеличения поверхности при её растяжении при условии постоянства температуры. Силовое (механическое) определение: поверхностное натяжение — это сила, действующая на единицу длины линии, которая ограничивает поверхность жидкости[1].
Сила поверхностного натяжения направлена по касательной к поверхности жидкости, перпендикулярно к участку контура, на который она действует и пропорциональна длине этого участка. Коэффициент пропорциональности

Но более правильно дать определение поверхностному натяжению, как энергии в джоулях на разрыв единицы поверхности (м²). В этом случае появляется ясный физический смысл понятия поверхностного натяжения.
В 1983 году было доказано теоретически и подтверждено данными из справочников[2], что понятие поверхностного натяжения жидкости однозначно является частью понятия внутренней энергии (хотя и специфической: для симметричных молекул близких по форме к шарообразным). Приведенные в этой журнальной статье формулы позволяют для некоторых веществ теоретически рассчитывать значения поверхностного натяжения жидкости по другим физико-химическим свойствам, например, по теплоте парообразования или по внутренней энергии[3][4]).
В 1985 году аналогичный взгляд на физическую природу поверхностного натяжения как части внутренней энергии при решении другой физической задачи был опубликован В. Вайскопфом в США[5].
Поверхностное натяжение возникает на границе газообразных, жидких и твёрдых тел. Обычно под термином «поверхностное натяжение» имеется в виду поверхностное натяжение жидких тел на границе жидкость — газ. В случае жидкой поверхности раздела поверхностное натяжение правомерно также рассматривать как силу, действующую на единицу длины контура поверхности и стремящуюся сократить поверхность до минимума при заданных объёмах фаз.
Прибор для измерения поверхностного натяжения называется тензиометр.
Проявления[править | править код]
Водомерка на поверхности воды.
Так как увеличение площади поверхности раздела жидкость — газ требует совершения работы, жидкость «стремится» уменьшить площадь своей поверхности:
- в невесомости порция жидкости принимает сферическую форму (сфера имеет наименьшую площадь поверхности среди всех тел одинакового объёма). То же самое происходит с каплей жидкости, помещаемой внутрь другой, несмешивающейся жидкости с первой жидкостью той же плотности (опыт Плато).
- ламинарная струя воды образует цилиндр, который затем разбивается на шаровидные капли из-за неустойчивости Рэлея — Плато.
- небольшие предметы со средней плотностью большей плотности жидкости способны «плавать» на поверхности жидкости, так как их вес оказывается уравновешенным силой поверхностного натяжения.
- некоторые насекомые (например, водомерки) способны передвигаться по воде, удерживаясь на её поверхности за счёт сил поверхностного натяжения.
- На многих поверхностях, именуемых несмачиваемыми (гидрофобными), вода (или другая жидкость) собирается в капли.
- Поплавок Ван Дер Месбрюгге – поплавок, имеющий кольцо. Если нажать на поплавок, то он будет удерживаться в нажатом состоянии благодаря силам поверхностного натяжения, они действуют на кольцо, и в свою очередь уравновешивают гидростатические силы, стремящиеся вытолкнуть поплавок из воды.
Теория[править | править код]
Пояснение возникновения силы поверхностного натяжения. Молекулы на границе раздела испытывают силы, стремящиеся втянуть их в жидкость, так как со стороны газа на них не действуют силы притяжения.
Площадь поверхности[править | править код]
Поверхность жидкости обладает свободной энергией:
- где
— коэффициент поверхностного натяжения,
— полная площадь поверхности жидкости[6].
Так как свободная энергия изолированной системы стремится к минимуму, то жидкость (в отсутствие внешних полей) стремится принять форму, имеющую минимальную площадь поверхности. Таким образом задача о форме жидкости сводится к изопериметрической задаче при заданных дополнительных условиях (начальное распределение, объём и т. п.). Свободная капля стремится принять форму шара, однако при более сложных начальных условиях задача о форме поверхности жидкости становится математически исключительно сложной.
Формула Лапласа[править | править код]
Рассмотрим тонкую жидкую плёнку, толщиной которой можно пренебречь. Стремясь минимизировать свою свободную энергию, плёнка создаёт разность давления с разных сторон. Этим объясняется образование мыльных пузырей: плёнка сжимается до тех пор, пока давление внутри пузыря не будет превышать атмосферное на величину добавочного давления поверхностного натяжения плёнки. Добавочное давление в точке поверхности зависит от средней кривизны в этой точке и задаётся формулой Лапласа:
Здесь 
Для случая поверхности кругового цилиндра радиуса 
Так как 
Из формулы Лапласа следует, что свободная мыльная плёнка, натянутая на рамку произвольной формы и не образующая пузырей, будет иметь среднюю кривизну, равную 0.
Зависимость от температуры[править | править код]
С увеличением температуры величина поверхностного натяжения уменьшается и равна нулю при критической температуре. Наиболее известная эмпирическая зависимость поверхностного натяжения от температуры была предложена Лорандом Этвёшом, так называемое правило Этвёша. В настоящее время получен вывод теоретической зависимости поверхностного натяжения от температуры в области до критических температур, подтверждающей правило Этвёша[7].
Способы определения[править | править код]
Способы определения поверхностного натяжения делятся на статические и динамические. В статических методах поверхностное натяжение определяется у сформировавшейся поверхности, находящейся в равновесии. Динамические методы связаны с разрушением поверхностного слоя. В случае измерения поверхностного натяжения растворов (особенно полимеров или ПАВ) следует пользоваться статическими методами. В ряде случаев равновесие на поверхности может наступать в течение нескольких часов (например, в случае концентрированных растворов полимеров с высокой вязкостью). Динамические методы могут быть применены для определения равновесного поверхностного натяжения и динамического поверхностного натяжения.
Например, для раствора мыла после перемешивания поверхностное натяжение 58 мДж/м², а после отстаивания — 35 мДж/м². То есть поверхностное натяжение меняется.
До установления равновесного оно будет динамическое.
Статические методы:
- Метод измерения высоты поднятия мениска в капилляре.
- Метод Вильгельми.
- Метод лежачей капли.
- Метод определения по форме висячей капли.
- Метод вращающейся капли.
Динамические методы:
- Метод дю Нуи (метод отрыва кольца).
- Сталагмометрический, или метод счета капель.
- Метод максимального давления пузырька.
- Метод осциллирующей струи.
- Метод стоячих волн.
- Метод бегущих волн.
Методы[править | править код]
Полностью стандартизованные методы измерений описываются в соответствующих ASTM, ГОСТ и т. д.
Метод вращающейся капли[править | править код]
Сущностью метода является измерение диаметра капли жидкости, вращающейся в более тяжелой жидкости[8]. Этот способ измерения годится для измерения низких или сверхнизких значений межфазного натяжения. Он широко применяется для микроэмульсий, измерения эффективности поверхностно-активных веществ (ПАВ) в нефтедобыче, а также для определения адсорбционных свойств.
Метод Дю Нуи (метод отрыва кольца)[править | править код]
Метод является классическим. Сущность метода вытекает из названия. Кольцо из платиновой проволоки плоскость которого параллельна поверхности жидкости медленно поднимают из жидкости, смачивающей его, усилие в момент отрыва кольца от поверхности и есть сила поверхностного натяжения и может быть пересчитано в поверхностную энергию. Метод подходит для измерения поверхностного натяжения ПАВ, трансформаторных масел и т. д.
Метод капиллярных волн[править | править код]
При возмущении жидкости колеблющейся пластиной, лежащей на её поверхности, по поверхности жидкости распространяются капиллярные волны. Если осветить кювету с жидкостью импульсным источником света (стробоскопом) с частотой вспышек равной частоте колебания пластины возмущения, то будет наблюдаться зрительно неподвижная волновая картина. По измеренной длине волны можно рассчитать величину поверхностного натяжения по формуле:
- где
— поверхностное натяжение;
— плотность жидкости;
— длина волны;
— частота колебания пластины;
— ускорение свободного падения.
Поверхностное натяжение некоторых жидкостей на границе с воздухом[править | править код]
| Вещество | Температура °C | Поверхностное натяжение(10−3 Н/м) |
|---|---|---|
| Хлорид натрия 6 M водный раствор | 20 | 82,55 |
| Хлорид натрия | 801 | 115 |
| Глицерин | 30 | 64,7 |
| Олово | 400 | 518 |
| Азотная кислота 70 % | 20 | 59,4 |
| Анилин | 20 | 42,9 |
| Ацетон | 20 | 23,7 |
| Бензол | 20 | 29,0 |
| Вода | 20 | 72,86 |
| Глицерин | 20 | 59,4 |
| Нефть | 20 | 26 |
| Ртуть | 20 | 486,5 |
| Серная кислота 85 % | 20 | 57,4 |
| Спирт этиловый | 20 | 22,8 |
| Уксусная кислота | 20 | 27,8 |
| Эфир этиловый | 20 | 16,9 |
| Раствор мыла | 20 | 43 |
Проявления[править | править код]
-
Мыльный пузырь
-
Маргаритка
-
На фотографии виден эффект, получивший название «слёзы вина»
-
Капля воды на листе
-
Вода набегает на сухую поверхность асфальта
| Видео, демонстрирующее передвижение водомерки по поверхности воды за счёт поверхностного натяжения. |
См. также[править | править код]
- Тензиометр
- Формула Жюрена
Ссылки[править | править код]
- [www.xumuk.ru/colloidchem/19.html Методы определения поверхностного натяжения]
- Видео о физической природе поверхностного натяжения жидкости как части внутренней энергии Архивная копия от 20 декабря 2020 на Wayback Machine (рус.)
Примечания[править | править код]
- ↑ Сумм Б. Д. Основы коллоидной химии
- ↑ (Cтатья: Журнал физической химии. 1983, № 10, с. 2528—2530). Дата обращения: 16 февраля 2014. Архивировано 21 февраля 2014 года.
- ↑ Хайдаров Г. Г., Хайдаров А. Г., Машек А. Ч. Физическая природа поверхностного натяжения жидкости // Вестник Санкт-Петербургского университета. Серия 4 (Физика, химия) 2011. Выпуск 1. с.3-8. Дата обращения: 16 февраля 2014. Архивировано из оригинала 22 февраля 2014 года.
- ↑ Хайдаров Г. Г., Хайдаров А. Г., Машек А. Ч., Майоров Е. Е. Влияние температуры на поверхностное натяжения // Вестник Санкт-Петербургского университета. Серия 4 (Физика, химия). 2012. Выпуск 1. с.24-28. Дата обращения: 16 февраля 2014. Архивировано из оригинала 22 февраля 2014 года.
- ↑ Weisskopf V. F. American Journal of Physics 53 (1985) 19-20.; V. F. Weisskopf, American Journal of Physics 53 (1985) 618—619.
- ↑ Обратите внимание, что плёнка, вроде стенки мыльного пузыря, имеет две стороны, так что площадь поверхности жидкости в два раза больше площади плёнки.
- ↑ Журнал «Вестник Санкт-Петербургского университета», 2012, вып. 1, с. 24—28
- ↑ Тензиометр SITE100. Дата обращения: 19 ноября 2008. Архивировано 3 апреля 2009 года.
|
|
Некоторые внешние ссылки в этой статье ведут на сайты, занесённые в спам-лист. Эти сайты могут нарушать авторские права, быть признаны неавторитетными источниками или по другим причинам быть запрещены в Википедии. Редакторам следует заменить такие ссылки ссылками на соответствующие правилам сайты или библиографическими ссылками на печатные источники либо удалить их (возможно, вместе с подтверждаемым ими содержимым). Список проблемных доменов
|
Содержание:
Поверхностное натяжение жидкости:
В отличие от газов жидкости имеют свободную поверхность. Молекулы, расположенные на поверхности жидкости, и молекулы внутри жидкости находятся в разных условиях:
a) молекулы внутри жидкости окружены другими молекулами жидкости со всех сторон. Молекула 1 внутри жидкости испытывает действие соседних молекул со всех сторон, поэтому равнодействующая сил притяжения, действующих на нее, равна нулю (f; молекула 1);
b) молекулы на поверхности жидкости испытывают действие со стороны соседних молекул жидкости только сбоку и снизу. Притяжение со стороны молекул газа (пара жидкости или воздуха) над жидкостью во много раз слабее, чем со стороны молекул жидкости, поэтому не принимаются во внимание (f; молекула 2). В результате каждая из равнодействующих сил
Сила поверхностного натяжения
Сила поверхностного натяжения – это сила, направленная по касательной к поверхности жидкости, перпендикулярно к линии, ограничивающей поверхность жидкости, и стремящаяся сократить площадь поверхности жидкости. Сила поверхностного натяжения прямо пропорциональна длине границы соприкосновения свободной поверхности жидкости с твердым телом:
Здесь 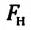
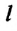
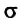
Коэффициент поверхностного натяжения
Коэффициент поверхностного натяжения – численно равен силе поверхностного натяжения, приходящейся на единицу длины линии, ограничивающей поверхность жидкости:
Значение коэффициента поверхностного натяжения зависит от вида жидкости и ее температуры, то есть с увеличением температуры жидкости коэффициент его поверхностного натяжения уменьшается и при критической температуре равен нулю. Единица коэффициента поверхностного натяжения в СИ:
Смачивающая и несмачивающая жидкость. При внимательном рассмотрении можно увидеть искривление поверхности жидкости на границе между жидкостью и твердым телом.
Мениск – это искривление свободной поверхности жидкости в месте ее соприкосновении с поверхностью твердого тела (или другой жидкости). Угол между поверхностью мениска и поверхностью твердого тела называется краевым углом.
Значение краевого угла 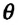
Смачивающая жидкость —это жидкость, у которой краевой угол острый. Сила взаимного притяжения между молекулами смачивающей жидкости и твердого тела больше, чем силы взаимного притяжения между молекулами самой жидкости. В результате свободная поверхность жидкости в сосуде становится вогнутой, например, вода в стеклянном сосуде – смачивающая жидкость (g).
Несмачивающая жидкость — это жидкость, у которой краевой угол тупой. Сила взаимного притяжения между молекулами несмачивающей жидкости и твердого тела меньше, чем сила взаимного притяжения между молекулами самой жидкости. В результате свободная поверхность жидкости в сосуде бывает выпуклой, например, ртуть в стеклянном сосуде — несмачивающая жидкость (i).
Капиллярные явления
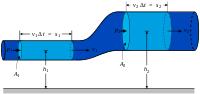
В повседневной жизни встречаются и используются тела, с легкостью впитывающие в себя воду, например, полотенце, промокательная бумага, сахар, кирпич, растения и др. Это свойство в телах объясняется существованием в них большого количества очень узких трубочек – капилляров.
Капилляр – это узкая трубка (канал) диаметром меньше 
Капиллярными явлениями называют явления подъема смачивающей и опускания несмачивающей жидкости по капилляру относительно общего уровня жидкости под действием сил поверхностного натяжения (j).
В таблице 6.4 дана зависимость между величинами, характеризующими жидкость, поднимающуюся в капилляре.
Таблица 6.4
|
Характеристики жидкости, поднимающейся в капилляре |
Формула |
| Вес жидкости, поднимающейся в капилляре |
Где |
| Масса жидкости, поднимающейся в капилляре | 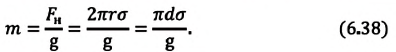 |
| Высота жидкости, поднимающейся в капилляре |
Если жидкость полностью смачиваемая, то получаем в Где |
| Давление жидкости, поднимающейся в капилляре | 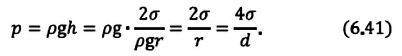 |
Поверхностное натяжение жидкости
Некоторые виды пауков могут передвигаться по поверхности воды не проваливаясь, как будто эта поверхность покрыта невидимой тонкой пленкой. такое же впечатление создается, если наблюдать за вытеканием воды из маленького отверстия — вода течет не тоненькой струйкой, а образует капли. Бумажная салфетка впитывает воду, едва коснувшись ее поверхности. какая сила является причиной всех этих явлений?
Каковы особенности поверхностного слоя жидкости
На свободной поверхности жидкости молекулы находятся в особых условиях, отличающихся от условий, в которых находятся молекулы внутри жидкости. Рассмотрим две молекулы — А и Б (рис. 33.1): молекула А находится внутри жидкости, а молекула Б — на ее поверхности. Молекула А окружена другими молекулами жидкости равномерно, поэтому силы, действующие на молекулу А со стороны молекул, попадающих в сферу межмолекулярного взаимодействия, скомпенсированы, то есть их равнодействующая равна нулю.
Молекула Б с одной стороны окружена молекулами жидкости, а с другой — молекулами газа. Со стороны жидкости на нее действует гораздо больше молекул, чем со стороны газа, поэтому равнодействующая F межмолекулярных сил направлена в глубь жидкости. Чтобы молекула из глубины попала в поверхностный слой, нужно совершить работу против межмолекулярных сил. Это означает, что молекулы поверхностного слоя жидкости (по сравнению с молекулами внутри жидкости) обладают избыточной потенциальной энергией. Эта избыточная энергия является частью внутренней энергии жидкости и называется поверхностной энергией (Wпов). Очевидно, что чем больше площадь S поверхности жидкости, тем больше поверхностная энергия: W S пов = σ , где σ (сигма) — коэффициент пропорциональности, который называют поверхностным натяжением жидкости.
Поверхностное натяжение жидкости — физическая величина, которая характеризует данную жидкость и равна отношению поверхностной энергии к площади поверхности жидкости:
Единица поверхностного натяжения в СИ — ньютон на метр:
Поверхностное натяжение жидкости определяется силами межмолекулярного взаимодействия, поэтому оно зависит:
- от природы жидкости: у летучих жидкостей (эфир, спирт, бензин) поверхностное натяжение меньше, чем у нелетучих (ртуть, жидкие металлы);
- температуры жидкости: чем выше температура жидкости, тем меньше поверхностное натяжение;
- присутствия в составе жидкости поверхностно активных веществ — их наличие уменьшает поверхностное натяжение;
- свойств газа, с которым жидкость граничит. В таблицах обычно приводят значение поверхностного натяжения на границе жидкости и воздуха при определенной температуре (табл. 1).
Таблица 1
Поверхностное натяжение σ некоторых жидкостей
Что такое сила поверхностного натяжения
Поскольку поверхностный слой жидкости обладает избыточной потенциальной энергией (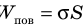
Наличие сил поверхностного натяжения делает поверхность жидкости похожей на натянутую резиновую пленку, однако упругие силы в резиновой пленке зависят от площади ее поверхности (от того, насколько пленка деформирована), а поверхность жидкости всегда «натянута» одинаково, то есть силы поверхностного натяжения не зависят от площади поверхности жидкости. Наличие сил поверхностного натяжения можно доказать с помощью такого опыта. Если проволочный каркас с закрепленной на нем нитью опустить в мыльный раствор, каркас затянется мыльной пленкой, а нить приобретет произвольную форму (рис. 33.2, а).
Если осторожно проткнуть иглой мыльную пленку по одну сторону от нити, сила поверхностного натяжения мыльного раствора, действующая с другой стороны, натянет нить (рис. 33.2, б). Опустим в мыльный раствор проволочную рамку, одна из сторон которой подвижна. На рамке образуется мыльная пленка (рис. 33.3). Будем растягивать эту пленку, действуя на перекладину (подвижную сторону рамки) с некоторой силой 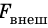
Если под действием этой силы перекладина переместится на ∆x , то внешние силы совершат работу: 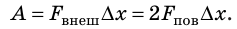
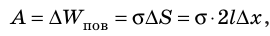
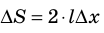
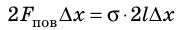
Таким образом, поверхностное натяжение σ численно равно силе поверхностного натяжения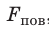
С одним из методов определения поверхностного натяжения жидкости вы ознакомитесь, выполняя лабораторную работу № 7.
- Заказать решение задач по физике
Где проявляется поверхностное натяжение
В жизни вы постоянно сталкиваетесь с проявлениями сил поверхностного натяжения. Так, благодаря ему на поверхности воды удерживаются легкие предметы (рис. 33.4) и некоторые насекомые.
Рис. 33.4. Монетка удерживается на поверхности воды благодаря силе поверхностного натяжения. (Чтобы провести такой опыт, монетку нужно потереть между пальцев и осторожно опустить на поверхность воды.)
Когда вы ныряете, ваши волосы расходятся во все стороны, но как только вы окажетесь над водой, волосы слипнутся, так как в этом случае площадь свободной поверхности воды намного меньше, чем при раздельном расположении прядей в воде. По этой же причине можно лепить фигуры из влажного песка: вода, обволакивая песчинки, прижимает их друг к другу.
Рис. 33.5. Капля удерживается около небольшого отверстия до тех пор, пока сила поверхностного натяжения уравновешивает силу тяжести
Стремлением жидкости уменьшить площадь поверхности объясняется и тот факт, что в условиях невесомости вода принимает форму шара, — при заданном объеме шарообразной форме соответствует наименьшая площадь поверхности. Форму шара приобретают тонкие мыльные пленки (мыльные пузыри). Поверхностным натяжением объясняется образование пены: пузырек газа, достигнув поверхности жидкости, имеет над собой тонкий слой жидкости; если пузырек мал, то архимедовой силы недостаточно, чтобы разорвать двойной поверхностный слой, и пузырек «застревает» вблизи поверхности. Благодаря поверхностному натяжению жидкость не выливается из маленького отверстия тоненькой струйкой, а капает (рис. 33.5), дождь не проливается через ткань зонта или палатки и т. д.
Почему одни жидкости собираются в капли, а другие растекаются
Наличие сил поверхностного натяжения проявляется в сферической форме мелких капелек росы, в каплях воды, разбегающихся по раскаленной плите, в капельках ртути на поверхности стекла. Однако при соприкосновении с твердым телом сферическая форма капли, как правило, не сохраняется. Форма свободной поверхности жидкости зависит также от сил взаимодействия молекул жидкости с молекулами твердого тела.
Если силы взаимодействия между молекулами жидкости больше, чем силы взаимодействия между молекулами жидкости и твердого тела, жидкость не смачивает поверхность твердого тела (рис. 33.6). Например, ртуть не смачивает стекло, а вода не смачивает покрытую сажей поверхность.
Рис. 33.6. Капля несмачивающей жидкости принимает форму, близкую к сферической, а поверхность жидкости вблизи стенки сосуда является выпуклой
Если же капельку ртути поместить на цинковую пластину, то капелька будет стремиться растечься по поверхности пластины; так же ведет себя и капелька воды на стекле (рис. 33.7). Если силы взаимодействия между молекулами жидкости меньше сил взаимодействия между молекулами жидкости и твердого тела, жидкость смачивает поверхность твердого тела.
Рис. 33.7. Капля смачивающей жидкости стремится растечься по поверхности твердого тела, а вблизи стенки сосуда поверхность жидкости принимает вогнутую форму
Почему жидкость поднимается в капиллярах
В природе часто встречаются тела, пронизанные многочисленными мелкими капиллярами (от лат. capillaris — волосяной) — узкими каналами произвольной формы. Такую структуру имеют бумага, дерево, почва, многие ткани и строительные материалы. В цилиндрических капиллярах искривленная поверхность жидкости представляет собой часть сферы, которую называют мениском. У смачивающей жидкости образуется вогнутый мениск (рис. 33.8, а), а у несмачивающей — выпуклый (рис. 33.8, б).
Рис. 33.8. капиллярные явления: а — смачивающая жидкость поднимается по капилляру; б — несмачивающая жидкость опускается в капилляре
Поверхность жидкости стремится к минимуму потенциальной энергии, а искривленная поверхность обладает большей площадью по сравнению с площадью сечения капилляра, поэтому поверхность жидкости стремится выровняться и под ней возникает избыточное (отрицательное или положительное) давление — лапласово давление (
Под вогнутой поверхностью (жидкость смачивает капилляр) лапласово давление отрицательное и жидкость втягивается в капилляр. Так поднимаются влага и питательные вещества в стеблях растений, керосин по фитилю, влага в почве. Вследствие лапласового давления салфетки или ткань впитывают воду, брюки в дождливую погоду сильно намокают снизу и т. д. Под выпуклой поверхностью (жидкость не смачивает капилляр) лапласово давление положительное и жидкость в капилляре опускается. Чем меньше радиус капилляра, тем больше высота подъема (или опускания) жидкости (см. задачу ниже).
Пример решения задачи
Капиллярную трубку радиусом r одним концом опустили в жидкость, смачивающую внутреннюю поверхность капилляра. На какую высоту поднимется жидкость в капилляре, если плотность жидкости ρ, а ее поверхностное натяжение σ ? Чему равно лапласово давление под вогнутой поверхностью капилляра? Смачивание считайте полным.
Решение:
На жидкость в капилляре действуют сила тяжести и сила поверхностного натяжения (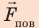
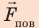
Поиск математической модели, решение
Поскольку m V = ρ , а объем воды в цилиндрическом капилляре 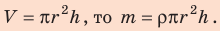
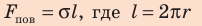
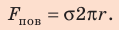
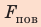

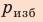
где R — радиус кривизны мениска (при полном смачивании r=R).
Ответ: (Данные выводы следует запомнить!)
- Высота подъема жидкости в капилляре прямо пропорциональна поверхностному натяжению жидкости и обратно пропорциональна плотности жидкости и радиусу капилляра:
.
- Лапласово давление (избыточное давление) под сферической поверхностью жидкости прямо пропорционально поверхностному натяжению жидкости и обратно пропорционально радиусу кривизны мениска:
.
Выводы:
- Свойства паров в физике
- Кипение жидкостей в физике
- Электромагнитные явления в физике
- Электромагнитные волны и их свойства
- Расчет количества теплоты при нагревании и охлаждении
- Удельная теплота сгорания топлива
- Плавление и кристаллизация в физике
- Испарение жидкостей в физике
Surface tension experimental demonstration with soap
Surface tension is the tendency of liquid surfaces at rest to shrink into the minimum surface area possible. Surface tension is what allows objects with a higher density than water such as razor blades and insects (e.g. water striders) to float on a water surface without becoming even partly submerged.
At liquid–air interfaces, surface tension results from the greater attraction of liquid molecules to each other (due to cohesion) than to the molecules in the air (due to adhesion).[1]
There are two primary mechanisms in play. One is an inward force on the surface molecules causing the liquid to contract.[2][3] Second is a tangential force parallel to the surface of the liquid.[3] This tangential force is generally referred to as the surface tension. The net effect is the liquid behaves as if its surface were covered with a stretched elastic membrane. But this analogy must not be taken too far as the tension in an elastic membrane is dependent on the amount of deformation of the membrane while surface tension is an inherent property of the liquid–air or liquid–vapour interface.[4]
Because of the relatively high attraction of water molecules to each other through a web of hydrogen bonds, water has a higher surface tension (72.8 millinewtons (mN) per meter at 20 °C) than most other liquids. Surface tension is an important factor in the phenomenon of capillarity.
Surface tension has the dimension of force per unit length, or of energy per unit area.[4] The two are equivalent, but when referring to energy per unit of area, it is common to use the term surface energy, which is a more general term in the sense that it applies also to solids.
In materials science, surface tension is used for either surface stress or surface energy.
Causes[edit]
Diagram of the cohesive forces on molecules of a liquid
Due to the cohesive forces, a molecule located away from the surface is pulled equally in every direction by neighbouring liquid molecules, resulting in a net force of zero. The molecules at the surface do not have the same molecules on all sides of them and therefore are pulled inward. This creates some internal pressure and forces liquid surfaces to contract to the minimum area.[2]
There is also a tension parallel to the surface at the liquid-air interface which will resist an external force, due to the cohesive nature of water molecules.[2][3]
The forces of attraction acting between molecules of the same type are called cohesive forces, while those acting between molecules of different types are called adhesive forces. The balance between the cohesion of the liquid and its adhesion to the material of the container determines the degree of wetting, the contact angle and the shape of meniscus. When cohesion dominates (specifically, adhesion energy is less than half of cohesion energy) the wetting is low and the meniscus is convex at a vertical wall (as for mercury in a glass container). On the other hand, when adhesion dominates (adhesion energy more than half of cohesion energy) the wetting is high and the similar meniscus is concave (as in water in a glass).
Surface tension is responsible for the shape of liquid droplets. Although easily deformed, droplets of water tend to be pulled into a spherical shape by the imbalance in cohesive forces of the surface layer. In the absence of other forces, drops of virtually all liquids would be approximately spherical. The spherical shape minimizes the necessary “wall tension” of the surface layer according to Laplace’s law.
Water droplet lying on a damask. Surface tension is high enough to prevent seeping through the textile
Another way to view surface tension is in terms of energy. A molecule in contact with a neighbor is in a lower state of energy than if it were alone. The interior molecules have as many neighbors as they can possibly have, but the boundary molecules are missing neighbors (compared to interior molecules) and therefore have a higher energy. For the liquid to minimize its energy state, the number of higher energy boundary molecules must be minimized. The minimized number of boundary molecules results in a minimal surface area.[5]
As a result of surface area minimization, a surface will assume the smoothest shape it can (mathematical proof that “smooth” shapes minimize surface area relies on use of the Euler–Lagrange equation). Since any curvature in the surface shape results in greater area, a higher energy will also result.
Physics[edit]
Physical units[edit]
Surface tension, represented by the symbol γ (alternatively σ or T), is measured in force per unit length. Its SI unit is newton per meter but the cgs unit of dyne per centimeter is also used. For example,[6]
Definition[edit]
This diagram illustrates the force necessary to increase the surface area. This force is proportional to the surface tension.
Surface tension can be defined in terms of force or energy.
In terms of force[edit]
Surface tension γ of a liquid is the force per unit length. In the illustration on the right, the rectangular frame, composed of three unmovable sides (black) that form a “U” shape, and a fourth movable side (blue) that can slide to the right. Surface tension will pull the blue bar to the left; the force F required to hold the movable side is proportional to the length L of the immobile side. Thus the ratio F/L depends only on the intrinsic properties of the liquid (composition, temperature, etc.), not on its geometry. For example, if the frame had a more complicated shape, the ratio F/L, with L the length of the movable side and F the force required to stop it from sliding, is found to be the same for all shapes. We therefore define the surface tension as
The reason for the
1/2 is that the film has two sides (two surfaces), each of which contributes equally to the force; so the force contributed by a single side is γL = F/2.
In terms of energy[edit]
Surface tension γ of a liquid is the ratio of the change in the energy of the liquid to the change in the surface area of the liquid (that led to the change in energy). This can be easily related to the previous definition in terms of force:[7] if F is the force required to stop the side from starting to slide, then this is also the force that would keep the side in the state of sliding at a constant speed (by Newton’s Second Law). But if the side is moving to the right (in the direction the force is applied), then the surface area of the stretched liquid is increasing while the applied force is doing work on the liquid. This means that increasing the surface area increases the energy of the film. The work done by the force F in moving the side by distance Δx is W = FΔx; at the same time the total area of the film increases by ΔA = 2LΔx (the factor of 2 is here because the liquid has two sides, two surfaces). Thus, multiplying both the numerator and the denominator of γ = 1/2F/L by Δx, we get
This work W is, by the usual arguments, interpreted as being stored as potential energy. Consequently, surface tension can be also measured in SI system as joules per square meter and in the cgs system as ergs per cm2. Since mechanical systems try to find a state of minimum potential energy, a free droplet of liquid naturally assumes a spherical shape, which has the minimum surface area for a given volume.
The equivalence of measurement of energy per unit area to force per unit length can be proven by dimensional analysis.[8]
Effects[edit]
Water[edit]
Several effects of surface tension can be seen with ordinary water:
- Beading of rain water on a waxy surface, such as a leaf. Water adheres weakly to wax and strongly to itself, so water clusters into drops. Surface tension gives them their near-spherical shape, because a sphere has the smallest possible surface area to volume ratio.
- Formation of drops occurs when a mass of liquid is stretched. The animation (below) shows water adhering to the faucet gaining mass until it is stretched to a point where the surface tension can no longer keep the drop linked to the faucet. It then separates and surface tension forms the drop into a sphere. If a stream of water were running from the faucet, the stream would break up into drops during its fall. Gravity stretches the stream, then surface tension pinches it into spheres.[9]
- Flotation of objects denser than water occurs when the object is nonwettable and its weight is small enough to be borne by the forces arising from surface tension.[5] For example, water striders use surface tension to walk on the surface of a pond in the following way. The nonwettability of the water strider’s leg means there is no attraction between molecules of the leg and molecules of the water, so when the leg pushes down on the water, the surface tension of the water only tries to recover its flatness from its deformation due to the leg. This behavior of the water pushes the water strider upward so it can stand on the surface of the water as long as its mass is small enough that the water can support it. The surface of the water behaves like an elastic film: the insect’s feet cause indentations in the water’s surface, increasing its surface area[10] and tendency of minimization of surface curvature (so area) of the water pushes the insect’s feet upward.
- Separation of oil and water (in this case, water and liquid wax) is caused by a tension in the surface between dissimilar liquids. This type of surface tension is called “interface tension”, but its chemistry is the same.
- Tears of wine is the formation of drops and rivulets on the side of a glass containing an alcoholic beverage. Its cause is a complex interaction between the differing surface tensions of water and ethanol; it is induced by a combination of surface tension modification of water by ethanol together with ethanol evaporating faster than water.
-
A. Water beading on a leaf
-
B. Water dripping from a tap
-
C. Water striders stay at the top of liquid because of surface tension
-
D. Lava lamp with interaction between dissimilar liquids: water and liquid wax
Surfactants[edit]
Surface tension is visible in other common phenomena, especially when surfactants are used to decrease it:
- Soap bubbles have very large surface areas with very little mass. Bubbles in pure water are unstable. The addition of surfactants, however, can have a stabilizing effect on the bubbles (see Marangoni effect). Note that surfactants actually reduce the surface tension of water by a factor of three or more.
- Emulsions are a type of colloid in which surface tension plays a role. Tiny fragments of oil suspended in pure water will spontaneously assemble themselves into much larger masses. But the presence of a surfactant provides a decrease in surface tension, which permits stability of minute droplets of oil in the bulk of water (or vice versa).
Surface curvature and pressure[edit]
Surface tension forces acting on a tiny (differential) patch of surface. δθx and δθy indicate the amount of bend over the dimensions of the patch. Balancing the tension forces with pressure leads to the Young–Laplace equation
If no force acts normal to a tensioned surface, the surface must remain flat. But if the pressure on one side of the surface differs from pressure on the other side, the pressure difference times surface area results in a normal force. In order for the surface tension forces to cancel the force due to pressure, the surface must be curved. The diagram shows how surface curvature of a tiny patch of surface leads to a net component of surface tension forces acting normal to the center of the patch. When all the forces are balanced, the resulting equation is known as the Young–Laplace equation:[11]
where:
- Δp is the pressure difference, known as the Laplace pressure.[12]
- γ is surface tension.
- Rx and Ry are radii of curvature in each of the axes that are parallel to the surface.
The quantity in parentheses on the right hand side is in fact (twice) the mean curvature of the surface (depending on normalisation).
Solutions to this equation determine the shape of water drops, puddles, menisci, soap bubbles, and all other shapes determined by surface tension (such as the shape of the impressions that a water strider’s feet make on the surface of a pond).
The table below shows how the internal pressure of a water droplet increases with decreasing radius. For not very small drops the effect is subtle, but the pressure difference becomes enormous when the drop sizes approach the molecular size. (In the limit of a single molecule the concept becomes meaningless.)
| Droplet radius | 1 mm | 0.1 mm | 1 μm | 10 nm |
|---|---|---|---|---|
| Δp (atm) | 0.0014 | 0.0144 | 1.436 | 143.6 |
Floating objects[edit]
Cross-section of a needle floating on the surface of water. Fw is the weight and Fs are surface tension resultant forces.
When an object is placed on a liquid, its weight Fw depresses the surface, and if surface tension and downward force become equal then it is balanced by the surface tension forces on either side Fs, which are each parallel to the water’s surface at the points where it contacts the object. Notice that small movement in the body may cause the object to sink. As the angle of contact decreases, surface tension decreases. The horizontal components of the two Fs arrows point in opposite directions, so they cancel each other, but the vertical components point in the same direction and therefore add up[5] to balance Fw. The object’s surface must not be wettable for this to happen, and its weight must be low enough for the surface tension to support it. If m denotes the mass of the needle and g acceleration due to gravity, we have
Liquid surface[edit]
To find the shape of the minimal surface bounded by some arbitrary shaped frame using strictly mathematical means can be a daunting task. Yet by fashioning the frame out of wire and dipping it in soap-solution, a locally minimal surface will appear in the resulting soap-film within seconds.[8][13]
The reason for this is that the pressure difference across a fluid interface is proportional to the mean curvature, as seen in the Young–Laplace equation. For an open soap film, the pressure difference is zero, hence the mean curvature is zero, and minimal surfaces have the property of zero mean curvature.
Contact angles[edit]
The surface of any liquid is an interface between that liquid and some other medium.[note 1] The top surface of a pond, for example, is an interface between the pond water and the air. Surface tension, then, is not a property of the liquid alone, but a property of the liquid’s interface with another medium. If a liquid is in a container, then besides the liquid/air interface at its top surface, there is also an interface between the liquid and the walls of the container. The surface tension between the liquid and air is usually different (greater) than its surface tension with the walls of a container. And where the two surfaces meet, their geometry must be such that all forces balance.[8][11]
Forces at contact point shown for contact angle greater than 90° (left) and less than 90° (right)
Where the two surfaces meet, they form a contact angle, θ, which is the angle the tangent to the surface makes with the solid surface. Note that the angle is measured through the liquid, as shown in the diagrams above. The diagram to the right shows two examples. Tension forces are shown for the liquid–air interface, the liquid–solid interface, and the solid–air interface. The example on the left is where the difference between the liquid–solid and solid–air surface tension, γls − γsa, is less than the liquid–air surface tension, γla, but is nevertheless positive, that is
In the diagram, both the vertical and horizontal forces must cancel exactly at the contact point, known as equilibrium. The horizontal component of fla is canceled by the adhesive force, fA.[8]
The more telling balance of forces, though, is in the vertical direction. The vertical component of fla must exactly cancel the difference of the forces along the solid surface, fls − fsa.[8]
| Liquid | Solid | Contact angle |
|||
|---|---|---|---|---|---|
| water |
|
0° | |||
| ethanol | |||||
| diethyl ether | |||||
| carbon tetrachloride | |||||
| glycerol | |||||
| acetic acid | |||||
| water | paraffin wax | 107° | |||
| silver | 90° | ||||
| methyl iodide | soda-lime glass | 29° | |||
| lead glass | 30° | ||||
| fused quartz | 33° | ||||
| mercury | soda-lime glass | 140° |
Since the forces are in direct proportion to their respective surface tensions, we also have:[11]
where
- γls is the liquid–solid surface tension,
- γla is the liquid–air surface tension,
- γsa is the solid–air surface tension,
- θ is the contact angle, where a concave meniscus has contact angle less than 90° and a convex meniscus has contact angle of greater than 90°.[8]
This means that although the difference between the liquid–solid and solid–air surface tension, γls − γsa, is difficult to measure directly, it can be inferred from the liquid–air surface tension, γla, and the equilibrium contact angle, θ, which is a function of the easily measurable advancing and receding contact angles (see main article contact angle).
This same relationship exists in the diagram on the right. But in this case we see that because the contact angle is less than 90°, the liquid–solid/solid–air surface tension difference must be negative:
Special contact angles[edit]
Observe that in the special case of a water–silver interface where the contact angle is equal to 90°, the liquid–solid/solid–air surface tension difference is exactly zero.
Another special case is where the contact angle is exactly 180°. Water with specially prepared Teflon approaches this.[11] Contact angle of 180° occurs when the liquid–solid surface tension is exactly equal to the liquid–air surface tension.
Liquid in a vertical tube[edit]
An old style mercury barometer consists of a vertical glass tube about 1 cm in diameter partially filled with mercury, and with a vacuum (called Torricelli’s vacuum) in the unfilled volume (see diagram to the right). Notice that the mercury level at the center of the tube is higher than at the edges, making the upper surface of the mercury dome-shaped. The center of mass of the entire column of mercury would be slightly lower if the top surface of the mercury were flat over the entire cross-section of the tube. But the dome-shaped top gives slightly less surface area to the entire mass of mercury. Again the two effects combine to minimize the total potential energy. Such a surface shape is known as a convex meniscus.
We consider the surface area of the entire mass of mercury, including the part of the surface that is in contact with the glass, because mercury does not adhere to glass at all. So the surface tension of the mercury acts over its entire surface area, including where it is in contact with the glass. If instead of glass, the tube was made out of copper, the situation would be very different. Mercury aggressively adheres to copper. So in a copper tube, the level of mercury at the center of the tube will be lower than at the edges (that is, it would be a concave meniscus). In a situation where the liquid adheres to the walls of its container, we consider the part of the fluid’s surface area that is in contact with the container to have negative surface tension. The fluid then works to maximize the contact surface area. So in this case increasing the area in contact with the container decreases rather than increases the potential energy. That decrease is enough to compensate for the increased potential energy associated with lifting the fluid near the walls of the container.
Illustration of capillary rise and fall. Red=contact angle less than 90°; blue=contact angle greater than 90°
If a tube is sufficiently narrow and the liquid adhesion to its walls is sufficiently strong, surface tension can draw liquid up the tube in a phenomenon known as capillary action. The height to which the column is lifted is given by Jurin’s law:[8]
where
- h is the height the liquid is lifted,
- γla is the liquid–air surface tension,
- ρ is the density of the liquid,
- r is the radius of the capillary,
- g is the acceleration due to gravity,
- θ is the angle of contact described above. If θ is greater than 90°, as with mercury in a glass container, the liquid will be depressed rather than lifted.
Puddles on a surface[edit]
Profile curve of the edge of a puddle where the contact angle is 180°. The curve is given by the formula:[11]
where
Small puddles of water on a smooth clean surface have perceptible thickness.
Pouring mercury onto a horizontal flat sheet of glass results in a puddle that has a perceptible thickness. The puddle will spread out only to the point where it is a little under half a centimetre thick, and no thinner. Again this is due to the action of mercury’s strong surface tension. The liquid mass flattens out because that brings as much of the mercury to as low a level as possible, but the surface tension, at the same time, is acting to reduce the total surface area. The result of the compromise is a puddle of a nearly fixed thickness.
The same surface tension demonstration can be done with water, lime water or even saline, but only on a surface made of a substance to which water does not adhere. Wax is such a substance. Water poured onto a smooth, flat, horizontal wax surface, say a waxed sheet of glass, will behave similarly to the mercury poured onto glass.
The thickness of a puddle of liquid on a surface whose contact angle is 180° is given by:[11]
where
- h is the depth of the puddle in centimeters or meters.
- γ is the surface tension of the liquid in dynes per centimeter or newtons per meter.
- g is the acceleration due to gravity and is equal to 980 cm/s2 or 9.8 m/s2
- ρ is the density of the liquid in grams per cubic centimeter or kilograms per cubic meter
Illustration of how lower contact angle leads to reduction of puddle depth
In reality, the thicknesses of the puddles will be slightly less than what is predicted by the above formula because very few surfaces have a contact angle of 180° with any liquid. When the contact angle is less than 180°, the thickness is given by:[11]
For mercury on glass, γHg = 487 dyn/cm, ρHg = 13.5 g/cm3 and θ = 140°, which gives hHg = 0.36 cm. For water on paraffin at 25 °C, γ = 72 dyn/cm, ρ = 1.0 g/cm3, and θ = 107° which gives hH2O = 0.44 cm.
The formula also predicts that when the contact angle is 0°, the liquid will spread out into a micro-thin layer over the surface. Such a surface is said to be fully wettable by the liquid.
The breakup of streams into drops[edit]
Breakup of an elongated stream of water into droplets due to surface tension.
In day-to-day life all of us observe that a stream of water emerging from a faucet will break up into droplets, no matter how smoothly the stream is emitted from the faucet. This is due to a phenomenon called the Plateau–Rayleigh instability,[11] which is entirely a consequence of the effects of surface tension.
The explanation of this instability begins with the existence of tiny perturbations in the stream. These are always present, no matter how smooth the stream is. If the perturbations are resolved into sinusoidal components, we find that some components grow with time while others decay with time. Among those that grow with time, some grow at faster rates than others. Whether a component decays or grows, and how fast it grows is entirely a function of its wave number (a measure of how many peaks and troughs per centimeter) and the radii of the original cylindrical stream.
Gallery[edit]
-
Breakup of a moving sheet of water bouncing off of a spoon.
-
Photo of flowing water adhering to a hand. Surface tension creates the sheet of water between the flow and the hand.
-
Surface tension prevents a coin from sinking: the coin is indisputably denser than water, so it must be displacing a volume greater than its own for buoyancy to balance mass.
-
An aluminium coin floats on the surface of the water at 10 °C. Any extra weight would drop the coin to the bottom.
-
A daisy. The entirety of the flower lies below the level of the (undisturbed) free surface. The water rises smoothly around its edge. Surface tension prevents water from displacing the air between the petals and possibly submerging the flower.
-
A metal paper clip floats on water. Several can usually be carefully added without overflow of water.
-
A metal paperclip floating on water. A grille in front of the light has created the ‘contour lines’ which show the deformation in the water surface caused by the metal paper clip.
Thermodynamics[edit]
Thermodynamic theories of surface tension[edit]
J.W. Gibbs developed the thermodynamic theory of capillarity based
on the idea of surfaces of discontinuity.[14] Gibbs considered the case of a sharp mathematical surface being placed somewhere within the microscopically fuzzy physical interface that exists between two homogeneous substances. Realizing that the exact choice of the surface’s location was somewhat arbitrary, he left it flexible. Since the interface exists in thermal and chemical equilibrium with the substances around it (having temperature T and chemical potentials μi), Gibbs considered the case where the surface may have excess energy, excess entropy, and excess particles, finding the natural free energy function in this case to be 

Gibbs’ placement of a precise mathematical surface in a fuzzy physical interface.
Considering a given subvolume 







For sufficiently macroscopic and gently curved surfaces, the surface free energy must simply be proportional to the surface area:[14][15]
for surface tension 

As stated above, this implies the mechanical work needed to increase a surface area A is dW = γ dA, assuming the volumes on each side do not change. Thermodynamics requires that for systems held at constant chemical potential and temperature, all spontaneous changes of state are accompanied by a decrease in this free energy 
Gibbs and other scientists have wrestled with the arbitrariness in the exact microscopic placement of the surface.[16] For microscopic surfaces with very tight curvatures, it is not correct to assume the surface tension is independent of size, and topics like the Tolman length come into play. For a macroscopic sized surface (and planar surfaces), the surface placement does not have a significant effect on γ however it does have a very strong effect on the values of the surface entropy, surface excess mass densities, and surface internal energy,[14]: 237 which are the partial derivatives of the surface tension function 
Gibbs emphasized that for solids, the surface free energy may be completely different from surface stress (what he called surface tension):[14]: 315 the surface free energy is the work required to form the surface, while surface stress is the work required to stretch the surface. In the case of a two-fluid interface, there is no distinction between forming and stretching because the fluids and the surface completely replenish their nature when the surface is stretched. For a solid, stretching the surface, even elastically, results in a fundamentally changed surface. Further, the surface stress on a solid is a directional quantity (a stress tensor) while surface energy is scalar.
Fifteen years after Gibbs, J.D. van der Waals developed the theory of capillarity effects based on the hypothesis of a continuous variation of density.[17] He added to the energy density the term 
Thermodynamics of bubbles[edit]
The pressure inside an ideal spherical bubble can be derived from thermodynamic free energy considerations.[15] The above free energy can be written as:
where 

For a spherical bubble, the volume and surface area are given simply by
and
Substituting these relations into the previous expression, we find
which is equivalent to the Young–Laplace equation when Rx = Ry.
Influence of temperature[edit]
Temperature dependence of the surface tension between the liquid and vapor phases of pure water
Temperature dependency of the surface tension of benzene
Surface tension is dependent on temperature. For that reason, when a value is given for the surface tension of an interface, temperature must be explicitly stated. The general trend is that surface tension decreases with the increase of temperature, reaching a value of 0 at the critical temperature. For further details see Eötvös rule. There are only empirical equations to relate surface tension and temperature:
- Eötvös:[21][22][23]
Here V is the molar volume of a substance, TC is the critical temperature and k is a constant valid for almost all substances.[21] A typical value is k = 2.1×10−7 J K−1 mol−2⁄3.[21][23] For water one can further use V = 18 ml/mol and TC = 647 K (374 °C).[24] A variant on Eötvös is described by Ramay and Shields:[25]
where the temperature offset of 6 K provides the formula with a better fit to reality at lower temperatures.
- Guggenheim–Katayama:[22]
γ° is a constant for each liquid and n is an empirical factor, whose value is 11/9 for organic liquids. This equation was also proposed by van der Waals, who further proposed that γ° could be given by the expression
where K2 is a universal constant for all liquids, and PC is the critical pressure of the liquid (although later experiments found K2 to vary to some degree from one liquid to another).[22]
Both Guggenheim–Katayama and Eötvös take into account the fact that surface tension reaches 0 at the critical temperature, whereas Ramay and Shields fails to match reality at this endpoint.
Influence of solute concentration[edit]
Solutes can have different effects on surface tension depending on the nature of the surface and the solute:
- Little or no effect, for example sugar at water|air, most organic compounds at oil/air
- Increase surface tension, most inorganic salts at water|air
- Non-monotonic change, most inorganic acids at water|air
- Decrease surface tension progressively, as with most amphiphiles, e.g., alcohols at water|air
- Decrease surface tension until certain critical concentration, and no effect afterwards: surfactants that form micelles
What complicates the effect is that a solute can exist in a different concentration at the surface of a solvent than in its bulk. This difference varies from one solute–solvent combination to another.
Gibbs isotherm states that:
- Γ is known as surface concentration, it represents excess of solute per unit area of the surface over what would be present if the bulk concentration prevailed all the way to the surface. It has units of mol/m2
- C is the concentration of the substance in the bulk solution.
- R is the gas constant and T the temperature
Certain assumptions are taken in its deduction, therefore Gibbs isotherm can only be applied to ideal (very dilute) solutions with two components.
Influence of particle size on vapor pressure[edit]
The Clausius–Clapeyron relation leads to another equation also attributed to Kelvin, as the Kelvin equation. It explains why, because of surface tension, the vapor pressure for small droplets of liquid in suspension is greater than standard vapor pressure of that same liquid when the interface is flat. That is to say that when a liquid is forming small droplets, the equilibrium concentration of its vapor in its surroundings is greater. This arises because the pressure inside the droplet is greater than outside.[25]
Molecules on the surface of a tiny droplet (left) have, on average, fewer neighbors than those on a flat surface (right). Hence they are bound more weakly to the droplet than are flat-surface molecules.
- Pv° is the standard vapor pressure for that liquid at that temperature and pressure.
- V is the molar volume.
- R is the gas constant
- rk is the Kelvin radius, the radius of the droplets.
The effect explains supersaturation of vapors. In the absence of nucleation sites, tiny droplets must form before they can evolve into larger droplets. This requires a vapor pressure many times the vapor pressure at the phase transition point.[25]
This equation is also used in catalyst chemistry to assess mesoporosity for solids.[26]
The effect can be viewed in terms of the average number of molecular neighbors of surface molecules (see diagram).
The table shows some calculated values of this effect for water at different drop sizes:
| P/P0 for water drops of different radii at STP[22] | ||||
|---|---|---|---|---|
| Droplet radius (nm) | 1000 | 100 | 10 | 1 |
| P/P0 | 1.001 | 1.011 | 1.114 | 2.95 |
The effect becomes clear for very small drop sizes, as a drop of 1 nm radius has about 100 molecules inside, which is a quantity small enough to require a quantum mechanics analysis.
Methods of measurement[edit]
Because surface tension manifests itself in various effects, it offers a number of paths to its measurement. Which method is optimal depends upon the nature of the liquid being measured, the conditions under which its tension is to be measured, and the stability of its surface when it is deformed. An instrument that measures surface tension is called tensiometer.
- Du Noüy ring method: The traditional method used to measure surface or interfacial tension. Wetting properties of the surface or interface have little influence on this measuring technique. Maximum pull exerted on the ring by the surface is measured.[21]
- Wilhelmy plate method: A universal method especially suited to check surface tension over long time intervals. A vertical plate of known perimeter is attached to a balance, and the force due to wetting is measured.
- Spinning drop method: This technique is ideal for measuring low interfacial tensions. The diameter of a drop within a heavy phase is measured while both are rotated.
- Pendant drop method: Surface and interfacial tension can be measured by this technique, even at elevated temperatures and pressures. Geometry of a drop is analyzed optically. For pendant drops the maximum diameter and the ratio between this parameter and the diameter at the distance of the maximum diameter from the drop apex has been used to evaluate the size and shape parameters in order to determine surface tension.
- Bubble pressure method (Jaeger’s method): A measurement technique for determining surface tension at short surface ages. Maximum pressure of each bubble is measured.
- Drop volume method: A method for determining interfacial tension as a function of interface age. Liquid of one density is pumped into a second liquid of a different density and time between drops produced is measured.
- Capillary rise method: The end of a capillary is immersed into the solution. The height at which the solution reaches inside the capillary is related to the surface tension by the equation discussed below.[27]
- Stalagmometric method: A method of weighting and reading a drop of liquid.
- Sessile drop method: A method for determining surface tension and density by placing a drop on a substrate and measuring the contact angle (see Sessile drop technique).[28]
- Du Noüy–Padday method: A minimized version of Du Noüy method uses a small diameter metal needle instead of a ring, in combination with a high sensitivity microbalance to record maximum pull. The advantage of this method is that very small sample volumes (down to few tens of microliters) can be measured with very high precision, without the need to correct for buoyancy (for a needle or rather, rod, with proper geometry). Further, the measurement can be performed very quickly, minimally in about 20 seconds.
- Vibrational frequency of levitated drops: The natural frequency of vibrational oscillations of magnetically levitated drops has been used to measure the surface tension of superfluid 4He. This value is estimated to be 0.375 dyn/cm at T = 0 K.[29]
- Resonant oscillations of spherical and hemispherical liquid drop: The technique is based on measuring the resonant frequency of spherical and hemispherical pendant droplets driven in oscillations by a modulated electric field. The surface tension and viscosity can be evaluated from the obtained resonant curves.[30][31][32]
- Drop-bounce method: This method is based on aerodynamic levitation with a split-able nozzle design. After dropping a stably levitated droplet onto a platform, the sample deforms and bounces back, oscillating in mid-air as it tries to minimize its surface area. Through this oscillation behavior, the liquid’s surface tension and viscosity can be measured.[33]
- By smartphone: Some smartphones can be used to measure the surface tension of a transparent liquid. The method is based on measuring the wavelength of capillary waves of known frequency. The smartphone is placed on top of a cup with the liquid. Then smartphone’s vibro-motor excites (through the cup) capillary ripples on the surface of the liquid, which are captured by smartphone’s camera.[34]
Values[edit]
Data table[edit]
| Liquid | Temperature (°C) | Surface tension, γ |
|---|---|---|
| Acetic acid | 20 | 27.60 |
| Acetic acid (45.1%) + Water | 30 | 40.68 |
| Acetic acid (10.0%) + Water | 30 | 54.56 |
| Acetone | 20 | 23.70 |
| Blood | 22 | 55.89 |
| Diethyl ether | 20 | 17.00 |
| Ethanol | 20 | 22.27 |
| Ethanol (40%) + Water | 25 | 29.63 |
| Ethanol (11.1%) + Water | 25 | 46.03 |
| Glycerol | 20 | 63.00 |
| n-Hexane | 20 | 18.40 |
| Hydrochloric acid 17.7 M aqueous solution | 20 | 65.95 |
| Isopropanol | 20 | 21.70 |
| Liquid helium II | −273 | 0.37[36] |
| Liquid nitrogen | −196 | 8.85 |
| Liquid oxygen | −182 | 13.2 |
| Mercury | 15 | 487.00 |
| Methanol | 20 | 22.60 |
| Molten Silver chloride | 650 | 163[37] |
| Molten Sodium chloride/Calcium chloride (47/53 mole %) | 650 | 139[38] |
| n-Octane | 20 | 21.80 |
| Sodium chloride 6.0 M aqueous solution | 20 | 82.55 |
| Sucrose (55%) + water | 20 | 76.45 |
| Water | 0 | 75.64 |
| Water | 25 | 71.97 |
| Water | 50 | 67.91 |
| Water | 100 | 58.85 |
| Toluene | 25 | 27.73 |
Surface tension of water[edit]
The surface tension of pure liquid water in contact with its vapor has been given by IAPWS[39] as
where both T and the critical temperature TC = 647.096 K are expressed in kelvins. The region of validity the entire vapor–liquid saturation curve, from the triple point (0.01 °C) to the critical point. It also provides reasonable results when extrapolated to metastable (supercooled) conditions, down to at least −25 °C. This formulation was originally adopted by IAPWS in 1976 and was adjusted in 1994 to conform to the International Temperature Scale of 1990.
The uncertainty of this formulation is given over the full range of temperature by IAPWS.[39] For temperatures below 100 °C, the uncertainty is ±0.5%.
Surface tension of seawater[edit]
Nayar et al.[40] published reference data for the surface tension of seawater over the salinity range of 20 ≤ S ≤ 131 g/kg and a temperature range of 1 ≤ t ≤ 92 °C at atmospheric pressure. The range of temperature and salinity encompasses both the oceanographic range and the range of conditions encountered in thermal desalination technologies. The uncertainty of the measurements varied from 0.18 to 0.37 mN/m with the average uncertainty being 0.22 mN/m.
Nayar et al. correlated the data with the following equation
where γsw is the surface tension of seawater in mN/m, γw is the surface tension of water in mN/m, S is the reference salinity[41] in g/kg, and t is temperature in degrees Celsius. The average absolute percentage deviation between measurements and the correlation was 0.19% while the maximum deviation is 0.60%.
The International Association for the Properties of Water and Steam (IAPWS) has adopted this correlation as an international standard guideline.[42]
See also[edit]
- Anti-fog
- Capillary wave — short waves on a water surface, governed by surface tension and inertia
- Cheerio effect — the tendency for small wettable floating objects to attract one another.
- Cohesion
- Dimensionless numbers
- Bond number or Eötvös number
- Capillary number
- Marangoni number
- Weber number
- Dortmund Data Bank — contains experimental temperature-dependent surface tensions
- Electrodipping force
- Electrowetting
- Electrocapillarity
- Eötvös rule — a rule for predicting surface tension dependent on temperature
- Fluid pipe
- Hydrostatic equilibrium—the effect of gravity pulling matter into a round shape
- Interface (chemistry)
- Meniscus — surface curvature formed by a liquid in a container
- Mercury beating heart — a consequence of inhomogeneous surface tension
- Microfluidics
- Sessile drop technique
- Sow-Hsin Chen
- Specific surface energy — same as surface tension in isotropic materials.
- Spinning drop method
- Stalagmometric method
- Surface pressure
- Surface science
- Surface tension biomimetics
- Surface tension values
- Surfactants — substances which reduce surface tension.
- Szyszkowski equation — calculating surface tension of aqueous solutions
- Tears of wine — the surface tension induced phenomenon seen on the sides of glasses containing alcoholic beverages.
- Tolman length — leading term in correcting the surface tension for curved surfaces.
- Wetting and dewetting
Explanatory notes[edit]
- ^ In a mercury barometer, the upper liquid surface is an interface between the liquid and a vacuum containing some molecules of evaporated liquid.
References[edit]
- ^ “Surface Tension – an overview | ScienceDirect Topics”. www.sciencedirect.com. Archived from the original on 2021-12-30. Retrieved 2021-12-30.
- ^ a b c “Surface Tension (Water Properties) – USGS Water Science School”. US Geological Survey. July 2015. Archived from the original on October 7, 2015. Retrieved November 6, 2015.
- ^ a b c Berry, M V (1971). “The molecular mechanism of surface tension” (PDF). Physics Education. 6 (2): 79–84. Bibcode:1971PhyEd…6…79B. doi:10.1088/0031-9120/6/2/001. S2CID 250797804. Archived (PDF) from the original on 31 December 2021. Retrieved 8 July 2020.
- ^ a b Halliday, David; Resnick, Robert; Krane, Kenneth S. (2010-04-20). Physics, Volume 2. John Wiley & Sons. p. 342. ISBN 978-0-471-40194-0. Archived from the original on 2022-09-22. Retrieved 2021-03-19.
- ^ a b c White, Harvey E. (1948). Modern College Physics. van Nostrand. ISBN 978-0-442-29401-4.
- ^ Bush, John W. M. (April 2004). “MIT Lecture Notes on Surface Tension, lecture 1” (PDF). Massachusetts Institute of Technology. Archived (PDF) from the original on March 8, 2022. Retrieved April 1, 2007.
- ^ “Mechanical definition of surface tension”. MIT. Archived from the original on April 12, 2013. Retrieved Dec 16, 2013.
- ^ a b c d e f g h Sears, Francis Weston; Zemanski, Mark W. (1955) University Physics 2nd ed. Addison Wesley
- ^ Bush, John W. M. (May 2004). “MIT Lecture Notes on Surface Tension, lecture 5” (PDF). Massachusetts Institute of Technology. Archived (PDF) from the original on February 26, 2007. Retrieved April 1, 2007.
- ^ Bush, John W. M. (May 2004). “MIT Lecture Notes on Surface Tension, lecture 3” (PDF). Massachusetts Institute of Technology. Archived (PDF) from the original on February 26, 2007. Retrieved April 1, 2007.
- ^ a b c d e f g h Pierre-Gilles de Gennes; Françoise Brochard-Wyart; David Quéré (2002). Capillarity and Wetting Phenomena—Drops, Bubbles, Pearls, Waves. Alex Reisinger. Springer. ISBN 978-0-387-00592-8.
- ^ Butt, Hans-Jürgen; Graf, Karlheinz; Kappl, Michael (2006). Physics and Chemistry of Interfaces. Wiley. p. 9. ISBN 978-3-527-60640-5.
- ^ Aaronson, Scott (March 2005) NP-complete Problems and Physical Reality Archived 2018-02-23 at the Wayback Machine. ACM SIGACT News
- ^ a b c d Gibbs, J.W. (2002) [1876–1878], “On the Equilibrium of Heterogeneous Substances”, in Bumstead, H.A.; Van Nameeds, R.G. (eds.), The Scientific Papers of J. Willard Gibbs, vol. 1, Woodbridge, CT: Ox Bow Press, pp. 55–354, ISBN 978-0918024770
- ^ a b Landau; Lifshitz (1980). Course of Theoretical Physics Volume 5: Statistical Physics I (3 ed.). Pergamon. pp. 517–537.
- ^ Rusanov, A (2005). “Surface thermodynamics revisited”. Surface Science Reports. 58 (5–8): 111–239. Bibcode:2005SurSR..58..111R. doi:10.1016/j.surfrep.2005.08.002. ISSN 0167-5729.
- ^ van der Waals, J.D. (1979) [1893], translated by Rowlinson, J.S., “The thermodynamic theory of capillarity under the hypothesis of a continuous variation of density”, J. Stat. Phys., 20 (2): 197–200, Bibcode:1979JSP….20..197R, doi:10.1007/BF01011513, S2CID 122954999
- ^ Cahn, J.W.; Hilliard, J.E. (1958), “Free energy of a nonuniform system. I. Interfacial free energy”, J. Chem. Phys., 28 (2): 258–266, Bibcode:1958JChPh..28..258C, doi:10.1063/1.1744102
- ^ Langer, J.S.; Bar-On, M.; Miller, H.D. (1975), “New computational method in the theory of spinodal decomposition”, Phys. Rev. A, 11 (4): 1417–1429, Bibcode:1975PhRvA..11.1417L, doi:10.1103/PhysRevA.11.1417
- ^
Gorban, A.N.; Karlin, I. V. (2016), “Beyond Navier–Stokes equations: capillarity of ideal gas”, Contemporary Physics (Review article), 58 (1): 70–90, arXiv:1702.00831, Bibcode:2017ConPh..58…70G, doi:10.1080/00107514.2016.1256123, S2CID 55317543 - ^ a b c d “Surface Tension by the Ring Method (Du Nouy Method)” (PDF). PHYWE. Archived (PDF) from the original on 2007-09-27. Retrieved 2007-09-08.
- ^ a b c d Adam, Neil Kensington (1941). The Physics and Chemistry of Surfaces, 3rd ed. Oxford University Press.
- ^ a b “Physical Properties Sources Index: Eötvös Constant”. Archived from the original on 2011-07-06. Retrieved 2008-11-16.
- ^ Vargaftik, N. B.; Volkov, B. N.; Voljak, L. D. (1983). “International Tables of the Surface Tension of Water” (PDF). Journal of Physical and Chemical Reference Data. 12 (3): 817. Bibcode:1983JPCRD..12..817V. doi:10.1063/1.555688. Archived from the original (PDF) on 2016-12-21. Retrieved 2017-07-13.
- ^ a b c Moore, Walter J. (1962). Physical Chemistry, 3rd ed. Prentice Hall.
- ^ Ertl, G.; Knözinger, H. and Weitkamp, J. (1997). Handbook of heterogeneous catalysis, Vol. 2, p. 430. Wiley-VCH, Weinheim. ISBN 3-527-31241-2
- ^ Calvert, James B. “Surface Tension (physics lecture notes)”. University of Denver. Archived from the original on 2007-09-15. Retrieved 2007-09-08.
- ^ “Sessile Drop Method”. Dataphysics. Archived from the original on August 8, 2007. Retrieved 2007-09-08.
- ^ Vicente, C.; Yao, W.; Maris, H.; Seidel, G. (2002). “Surface tension of liquid 4He as measured using the vibration modes of a levitated drop”. Physical Review B. 66 (21): 214504. Bibcode:2002PhRvB..66u4504V. doi:10.1103/PhysRevB.66.214504.
- ^ Zografov, Nikolay (2014). “Droplet oscillations driven by an electric field”. Colloids and Surfaces A: Physicochemical and Engineering Aspects. 460: 351–354. doi:10.1016/j.colsurfa.2013.12.013.
- ^ Tankovsky, N. (2013). “Electrically Driven Resonant Oscillations of Pendant Hemispherical Liquid Droplet and Possibility to Evaluate the Surface Tension in Real Time”. Zeitschrift für Physikalische Chemie. 227 (12): 1759–1766. doi:10.1524/zpch.2013.0420. S2CID 101722165.
- ^ Tankovsky, Nikolay (2011). “Oscillations of a Hanging Liquid Drop, Driven by Interfacial Dielectric Force”. Zeitschrift für Physikalische Chemie. 225 (4): 405–411. doi:10.1524/zpch.2011.0074. S2CID 101625925.
- ^ Sun, Yifan; Muta, Hiroaki; Ohishi, Yuji (June 2021). “Novel Method for Surface Tension Measurement: the Drop-Bounce Method”. Microgravity Science and Technology. 33 (3): 32. Bibcode:2021MicST..33…32S. doi:10.1007/s12217-021-09883-7.
- ^ Yue, Shichao; Katabi, Dina (2021). “Liquid testing with your smartphone”. Communications of the ACM. 64 (10): 75–83. doi:10.1145/3481038. ISSN 0001-0782.
- ^ Lange’s Handbook of Chemistry (1967) 10th ed. pp 1661–1665 ISBN 0-07-016190-9 (11th ed.)
- ^ Brouwer, W; Pathria, R. K (1967). “On the Surface Tension of Liquid Helium II”. Physical Review. 163 (1): 200–205. Bibcode:1967PhRv..163..200B. doi:10.1103/PhysRev.163.200.
- ^ Moser, Z; Gasior, W; Rzyman, K (1982). “Activities and Surface Tension of Liquid AgCl-KCl Solutions”. Journal of the Electrochemical Society. 129 (3): 502–506. doi:10.1149/1.2131529.
- ^ Addison, C; Coldrey, J (1960). “Influence of Surface Reactions on the Interface Between Liquid Sodium and Molten Sodium Chloride + Calcium Chloride Mixtures”. Transactions of the Faraday Society. 56: 840–845. doi:10.1039/tf9605600840.
- ^ a b International Association for the Properties of Water and Steam (June 2014). “Revised Release on Surface Tension of Ordinary Water Substance”. Archived from the original on 2016-04-04. Retrieved 2016-02-20.
- ^ Nayar, K. G; Panchanathan, D; McKinley, G. H; Lienhard, J. H (November 2014). “Surface tension of seawater” (PDF). J. Phys. Chem. Ref. Data. 43 (4): 43103. Bibcode:2014JPCRD..43d3103N. doi:10.1063/1.4899037. hdl:1721.1/96884. Archived from the original on 2022-09-22. Retrieved 2018-04-20.
- ^ Millero, Frank J; Feistel, Rainer; Wright, Daniel G; McDougall, Trevor J (January 2008). “The composition of Standard Seawater and the definition of the Reference-Composition Salinity Scale”. Deep-Sea Research Part I. 55 (1): 50. Bibcode:2008DSRI…55…50M. doi:10.1016/j.dsr.2007.10.001.
- ^ Guideline on the Surface Tension of Seawater (PDF) (Technical report). International Association for the Properties of Water and Steam. October 2019. IAPWS G14-19. Archived (PDF) from the original on 2020-03-26. Retrieved 2020-03-26.
External links[edit]
- “Why is surface tension parallel to the interface?”. Physics Stack Exchange. Retrieved 2021-03-19.
- Berry, M V (1971-03-01). “The molecular mechanism of surface tension”. Physics Education. 6 (2): 79–84. doi:10.1088/0031-9120/6/2/001. ISSN 0031-9120.
- Marchand, Antonin; Weijs, Joost H.; Snoeijer, Jacco H.; Andreotti, Bruno (2011-09-26). “Why is surface tension a force parallel to the interface?”. American Journal of Physics. 79 (10): 999–1008. doi:10.1119/1.3619866. ISSN 0002-9505. arXiv: https://arxiv.org/abs/1211.3854
- On surface tension and interesting real-world cases
- Surface Tensions of Various Liquids
- Calculation of temperature-dependent surface tensions for some common components
- Surface tension calculator for aqueous solutions containing the ions H+, NH+
4, Na+, K+, Mg2+, Ca2+, SO2−
4, NO−
3, Cl−, CO2−
3, Br− and OH−. - T. Proctor Hall (1893) New methods of measuring surface tension in liquids, Philosophical Magazine (series 5, 36: 385–415), link from Biodiversity Heritage Library.
- The Bubble Wall[permanent dead link] (Audio slideshow from the National High Magnetic Field Laboratory explaining cohesion, surface tension and hydrogen bonds)
- C. Pfister: Interface Free Energy. Scholarpedia 2010 (from first principles of statistical mechanics)
- Surface and Interfacial Tension
- “Molten salts mixture surface tension”. The Journal of Chemical Thermodynamics. 3 (2): 259–265. March 1971. doi:10.1016/S0021-9614(71)80111-8.
Поверхностное натяжение
(~W_p = sigma cdot S) ,
где Wp – поверхностная энергия (потенциальная энергия поверхностного слоя жидкости) (Дж); σ – удельная поверхностная энергия или коэффициент поверхностного натяжения (табличная величина) (Дж/м2 или Н/м); S – площадь поверхности жидкости (м2).
- Мыльные пузыри имеют две поверхности (наружную и внутреннюю оболочки), поэтому площадь их поверхности необходимо умножать на 2.
(~A = sigma cdot Delta S) ,
где A – работа внешних сил, совершаемая при изотермическом увеличении площади жидкости (Дж); σ – удельная поверхностная энергия или коэффициент поверхностного натяжения (табличная величина) (Дж/м2 или Н/м); ΔS – изменение площади поверхности жидкости (м2).
- Мыльные пузыри имеют две поверхности (наружную и внутреннюю оболочки), поэтому площадь их поверхности необходимо умножать на 2.
- ΔS > 0, если площадь поверхности увеличивается, ΔS < 0, если площадь поверхности уменьшается.
- А` = –А, где А` – работа сил поверхностного натяжения.
(~F = sigma cdot l_p) ,
где F – сила поверхностного натяжения жидкости (Н); σ – удельная поверхностная энергия или коэффициент поверхностного натяжения (табличная величина) (Дж/м2 или Н/м); lp – длина границы поверхности жидкости (м).
(~p_d = frac{2 sigma}{R}) ,
где pd – дополнительное (лапласовское) давление жидкости под мениском (Па); R – радиус мениска (м).
- В мыльной пленке две поверхности, поэтому дополнительное давление (~p_{pl} = frac{4 sigma}{R}) .
(~h = frac{2 sigma}{rho cdot g cdot R}) ,
где h – высота подъема (опускания) столба жидкости в капилляре (м); ρ – плотность жидкости в капилляре (кг/м3); R – радиус капилляра (м); g – ускорение свободного падения в данной местности (м/с2).
- Если жидкость смачивает капилляр, то жидкость будет подниматься; если жидкость не смачивает капилляр, то жидкость – опускаться.
В
§ 7.1 были
рассмотрены опыты, свидетельствующие
о стремлении поверхности жидкости к
сокращению. Это сокращение вызывается
силой поверхностного натяжения.
Силу, которая
действует вдоль поверхности жидкости
перпендикулярно линии, ограничивающей
эту поверхность, и стремится сократить
ее до минимума, называют силой
поверхностного натяжения.
Измерение силы поверхностного натяжения
Чтобы
измерить силу поверхностного натяжения,
проделаем следующий опыт. Возьмем
прямоугольную проволочную рамку, одна
сторона которой АВ
длиной
l
может
перемещаться с малым трением в вертикальной
плоскости. Погрузив рамку в сосуд с
мыльным раствором, получим на ней мыльную
пленку (рис. 7.11, а). Как только мы вытащим
рамку из мыльного раствора, проволочка
АВ
сразу
же придет в движение. Мыльная пленка
будет сокращать свою поверхность.
Следовательно, на проволочку АВ
действует
сила, направленная перпендикулярно
проволочке в сторону пленки. Это и есть
сила поверхностного натяжения.
Рис. 7.11
Чтобы
помешать проволочке двигаться, надо к
ней приложить некоторую силу. Для
создания этой силы можно прикрепить к
проволочке мягкую пружину, закрепленную
на основании штатива (см. рис. 7.11, о). Сила
упругости пружины вместе с силой тяжести,
действующей на проволочку, в сумме
составят результирующую силу
Для
равновесия проволочки необходимо, чтобы
выполнялось равенство
,
где
—
сила поверхностного натяжения, действующая
на проволочку со стороны одной из
поверхностей пленки (рис. 7.11, б).
Отсюда
.
От чего зависит сила поверхностного натяжения?
Если
проволочку переместить вниз на расстояние
h,
то
внешняя сила F1
=
2F
совершит
работу
(7.4.1)
Согласно
закону сохранения энергии эта работа
равна изменению энергии (в данном случае
поверхностной) пленки. Начальная
поверхностная энергия мыльной пленки
площадью S1
равна Uп1
= =2σS1,
так
как пленка имеет две поверхности
одинаковой площади. Конечная поверхностная
энергия
где
S2
—
площадь пленки после перемещения
проволочки на расстояние h.
Следовательно,
Так как
то
(7.4.2)
Приравнивая правые
части выражений (7.4.1) и (7.4.2), получим:
Отсюда
сила поверхностного натяжения, действующая
на границу поверхностного слоя длиной
l,
равна:
(7.4.3)
Направлена
сила поверхностного натяжения по
касательной к поверхности перпендикулярно
границе поверхностного слоя (перпендикулярно
проволочке АВ
в
данном случае, см. рис. 7.11, а).
Измерение коэффициента поверхностного натяжения
Существует много
способов измерения поверхностного
натяжения жидкостей. Например,
поверхностное натяжение а можно
определить, пользуясь установкой,
изображенной на рисунке 7.11. Мы рассмотрим
другой способ, не претендующий на большую
точность результата измерений.
Прикрепим
к чувствительному динамометру медную
проволочку, изогнутую так, как показано
на рисунке 7.12, a. Подставим под проволочку
сосуд с водой так, чтобы проволочка
коснулась поверхности воды (рис. 7.12, б)
и
«прилипла» к ней. Будем теперь медленно
опускать сосуд с водой (или, что то же,
поднимать динамометр с проволочкой).
Мы увидим, что вместе с проволочкой
поднимается обволакивающая ее водяная
пленка, а показание динамометра при
этом постепенно увеличивается. Оно
достигает максимального значения в
момент разрыва водяной пленки и «отрыва»
проволочки от воды. Если из показаний
динамометра в момент отрыва проволочки
вычесть ее вес, то получится сила F,
равная
удвоенной силе поверхностного натяжения
(у водяной пленки две поверхности):
где
l
—
длина проволочки.
Рис. 7.12
Отсюда
При
длине проволочки 1 = 5 см и температуре
20 °С сила оказывается равной 7,3 · 10-3
Н. Тогда
Результаты
измерений поверхностных натяжений
некоторых жидкостей приведены в таблице
4.
Таблица 4
|
Жидкость |
Температура, °С |
Поверхностное |
|
Вода(чистая) Раствор мыла Спирт Эфир Ртуть Золото(расплавленное) Жидкий водород Жидкий гелий |
20 20 20 20 20 1130 -253 -269 |
0,073 0,040 0,022 0,017 0,470 1,102 0,0021 0,00012 |
Из таблицы 4 видно,
что у легкоиспаряющихся жидкостей
(эфира, спирта) поверхностное натяжение
меньше, чем у нелетучих жидкостей,
например у ртути. Очень мало поверхностное
натяжение у жидкого водорода и особенно
у жидкого гелия. У жидких металлов
поверхностное натяжение, наоборот,
очень велико.
Различие в
поверхностном натяжении жидкостей
объясняется различием в силах
межмолекулярного взаимодействия.
Соседние файлы в предмете [НЕСОРТИРОВАННОЕ]
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #
- #






















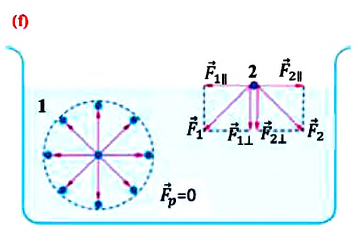
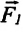


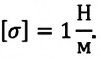
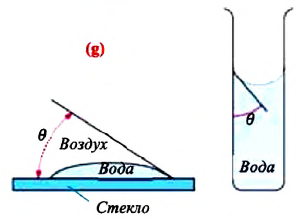
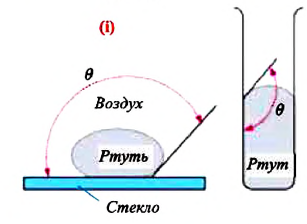

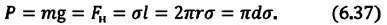
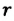 — радиус капилляра,
— радиус капилляра, 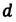 — диаметр капилляра.
— диаметр капилляра.
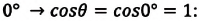

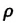 — плотность жидкости, поднимающейся в капилляре. Высота подъема жидкости в капилляре зависит от рода жидкости и обратно пропорциональна радиусу капилляра.
— плотность жидкости, поднимающейся в капилляре. Высота подъема жидкости в капилляре зависит от рода жидкости и обратно пропорциональна радиусу капилляра.
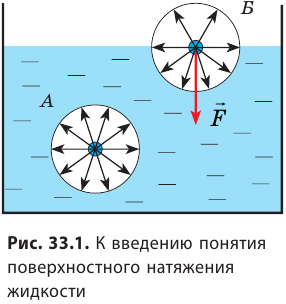
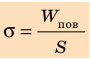
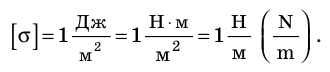
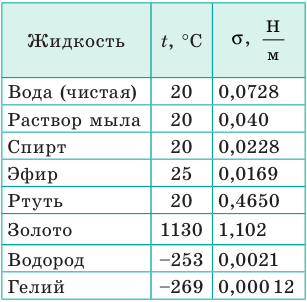
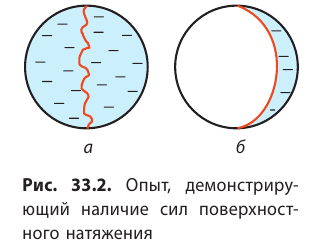
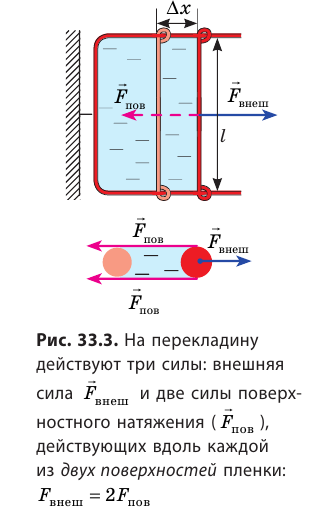

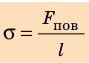



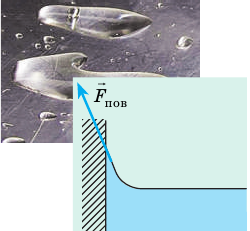
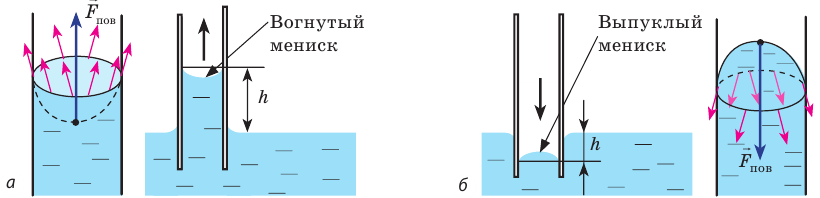
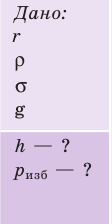
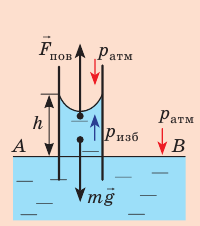
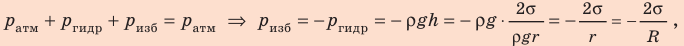
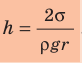 .
.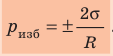 .
.














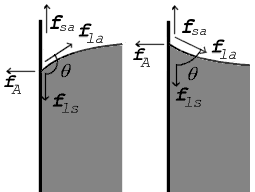
































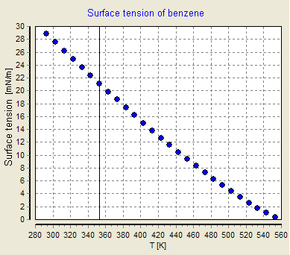






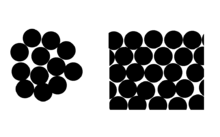
![{displaystyle gamma _{text{w}}=235.8left(1-{frac {T}{T_{text{C}}}}right)^{1.256}left[1-0.625left(1-{frac {T}{T_{text{C}}}}right)right]~{text{mN/m}},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0b65193820b2a29652f19d30a1adecdf0c13c8ea)