Эта статья — о научном эксперименте. О романе Умберто Эко см. Маятник Фуко (роман).
| Маятник Фуко | |
|---|---|
 Маятник Фуко в парижском Пантеоне |
|
 |
|
| Названо в честь | Жак Бернар Леон Фуко |
Ма́ятник Фуко́ — математический маятник, используемый для экспериментальной демонстрации суточного вращения Земли. Представляет собой тело массой до нескольких десятков килограммов на гибком подвесе длиной до нескольких десятков метров. Верхний конец нити укрепляется в кардановом подвесе или на упорном шарикоподшипнике для свободного движения маятника в любой вертикальной плоскости. Наличие суточного вращения нашей планеты ответственно за постепенный поворот плоскости колебаний маятника относительно связанных с Землёй ориентиров[1]. Впервые эффект продемонстрирован Л. Фуко (1851 год), в настоящее время в мире имеются действующие маятники Фуко, используемые в демонстрационных целях.
Наблюдение за маятником Фуко является одним из способов решения следующей занимательной задачи П. Л. Капицы[2]:
Астрономические наблюдения показывают, что на планете Венера полная облачность, так что «жители» Венеры лишены возможности наблюдать небесные светила. Опишите, каким методом они могли бы точно измерить длину своих суток.[3]
Самый большой в мире маятник Фуко (длиной 98 м, период 20 с) провисел в Исаакиевском соборе в Ленинграде с 1931 по 1986 год[4][1].
Эксперименты Фуко[править | править код]
Французский физик и астроном Лео́н Фуко́ впервые осуществил свой эксперимент в 2 часа ночи 8 января 1851 года в погребе своего дома на углу улиц Асса́ (фр.) (рус. и Вожира́р (фр.) (рус. в Париже. Для этого был использован маятник длиной 2 метра. В феврале с разрешения Араго он повторил опыт в Парижской обсерватории, на этот раз удлинив маятник до 11 метров. В подготовке эксперимента принимал также участие Фромент — ассистент Фуко[5].
Первая публичная демонстрация была осуществлена уже в марте 1851 года в парижском Пантеоне: под куполом Пантеона он подвесил на стальной проволоке длиной 67 м металлический шар массой 28 кг с закреплённым на нём остриём. Крепление маятника позволяло ему свободно колебаться во всех направлениях, под точкой крепления было сделано круговое ограждение диаметром 6 м, по краю ограждения была насыпана песчаная дорожка таким образом, чтобы маятник в своём движении мог при её пересечении прочерчивать на песке отметки. Чтобы избежать бокового толчка при пуске маятника, его отвели в сторону и привязали верёвкой, после чего верёвку пережгли. Период колебания маятника при такой длине подвеса составляет 16,4 секунды, при каждом колебании отклонение от предыдущего пересечения песчаной дорожки составляет около 3 мм, за час плоскость колебаний маятника поворачивается более чем на 11° по часовой стрелке, то есть примерно за 32 часа совершает полный оборот и возвращается в прежнее положение[6][4].
Опыты Фуко в Пантеоне проводились по просьбе президента Второй республики Луи Бонапарта (будущего Наполеона III)[7]. Широко распространена история о том, что Папа Пий IX благословил опыт Фуко, который показал бы всемогущество творца Вселенной. Документальных свидетельств благословения не известно[8][неавторитетный источник].
Объяснение опыта[править | править код]

Маятник Фуко на Северном полюсе. Ось вращения Земли лежит в плоскости колебаний маятника
Неинерциальная система отсчёта[править | править код]
В неинерциальной системе отсчёта, связанной с Землёй, поворот плоскости качаний маятника можно объяснить действием силы Кориолиса, которая максимальна на полюсе и отсутствует на экваторе[9][1].
Инерциальная система отсчёта[править | править код]
На тело маятника действуют только две силы — сила притяжения со стороны Земли и сила натяжения нити подвеса. Считая тело материальной точкой, получаем, что эти две силы, направленные вдоль пересекающихся в этой точке прямых, однозначно задают плоскость качаний маятника, которая не может изменяться. Следовательно, её вращение относительно поверхности Земли обусловлено вращением планеты вокруг собственной оси[1].
В простейшем случае — на полюсе, где ось вращения Земли лежит в плоскости качаний маятника — наблюдатель видит вращение этой плоскости в сторону, противоположную вращению Земли, на 360° за звёздные сутки (23 ч. 56 мин. 4 с, 15° за звёздный час)[1].
В точке с любой другой географической широтой плоскость качания маятника не может оставаться неподвижной относительно звёзд, а участвует во вращении Земли. Вектор 


В южном полушарии картина явления совершенно та же, за исключением того, что вращение плоскости качаний маятника происходит в противоположную сторону[1].
Расчётные формулы[править | править код]
В произвольной точке с географической широтой 

Строгое рассмотрение задачи приводит к двум уточнениям. Во-первых, маятник движется не в плоскости, а по конической поверхности. Маятник, запущенный классическим способом — оттягиванием в крайнее положение и пережиганием оттягивающей нити, в точку равновесия не попадает, промахиваясь мимо неё в северном полушарии вправо, а в южном — влево[1]. На показанной в статье анимации маятник запущен ударом в точке равновесия, поэтому он при каждом колебании возвращается в неё, описывая петли.
Во-вторых, скорость вращения плоскости колебаний маятника зависит и от длины подвеса[1]:
где 

Таким образом, увеличение длины нити уменьшает добавочный член, влияющий на скорость вращения маятника, в связи с чем целесообразно пользоваться маятниками возможно большей длины[1].
См. также[править | править код]
- Математический маятник
Примечания[править | править код]
- ↑ 1 2 3 4 5 6 7 8 9 10 11 Ерпылев Н. П. Фуко маятник // Физический энциклопедический словарь / гл. ред. Б. А. Введенский, Б. М. Вул. — Москва: Советская энциклопедия, 1966. — Т. 5 Спектр — Яркость. — С. 369. — 576 с. — 55 000 экз.
- ↑ Олимпиады по физике. Турнир им. Ломоносова 26.09.1999. Дата обращения: 4 марта 2010. Архивировано 30 января 2012 года.
- ↑ Капица П. Л. Физические задачи. — М.: «Знание», 1966. — 16 с. Архивировано 8 декабря 2009 года.
- ↑ 1 2 Фуко маятник // Большая российская энциклопедия : [в 35 т.] / гл. ред. Ю. С. Осипов. — М. : Большая российская энциклопедия, 2004—2017.
- ↑ G. Barenboim, J. A. Oteo. One pendulum to run them all (англ.). — 2013. — arXiv:1304.7922.
- ↑ Маятник Фуко. Дата обращения: 3 марта 2009. Архивировано из оригинала 31 октября 2008 года.
- ↑ Crease R. P. The prism and the pendulum. The ten most beautiful experiments in science Архивная копия от 27 сентября 2013 на Wayback Machine
- ↑ Благословлял ли Ватикан опыты с маятником Фуко? dictionnaire.narod.ru. Дата обращения: 15 июля 2022.
- ↑ Маятник Фуко в Исаакиевском соборе на YouTube // Киевнаучфильм, 1979 год
Ссылки[править | править код]
Впервые публичная демонстрация была осуществлена Фуко в 1851 г. в Парижском Пантеоне: под куполом Пантеона он подвесил металлический шар массой 28 кг с закреплённым на нём остриём на стальной проволоке длиной 67 м, крепление маятника позволяло ему свободно колебаться во всех направлениях, под точкой крепления было сделано круговое ограждение диаметром 6 метров, по краю ограждения была насыпана песчаная дорожка таким образом, чтобы маятник в своём движении мог при её пересечении прочерчивать на песке отметки. Чтобы избежать бокового толчка при пуске маятника, его отвели в сторону и привязали верёвкой, после чего верёвку пережгли.
Период колебания маятника при такой длине подвеса составлял 16,4 секунд, при каждом колебании отклонение от предыдущего пересечения песчаной дорожки составляло ~3 мм, за час плоскость колебаний маятника повернулась более чем на 11° по часовой стрелке, то есть примерно за 32 часа совершила полный оборот и вернулась в прежнее положение. —–>
Маятником Фуко называют сферический маятник длиной  движение которого рассматривается в системе координат, жестко связанной с вращающейся Землей. Кроме силы Кориолиса от добавочного ускорения на точку действует сила натяжения нити
движение которого рассматривается в системе координат, жестко связанной с вращающейся Землей. Кроме силы Кориолиса от добавочного ускорения на точку действует сила натяжения нити  (рис. 179). Уравнения движения принимают вид
(рис. 179). Уравнения движения принимают вид


Уравнения движения сферического маятника оказываются более сложными, чем уравнения движения свободной материальной точки, поскольку в эти уравнения входит сила реакции, являющаяся неизвестной функцией координат. Можно пытаться провести интегрирование уравнений методом последовательных приближений, предварительно исключив реакцию. Но и эта задача оказывается весьма сложной. Обычно при исследовании ограничиваются случаем малых колебаний (колебания с малой амплитудой), рассматривая движение приближенным методом. Отношения  рассматриваются как малые величины, квадратами которых в уравнениях движения можно пренебрегать. В таком случае
рассматриваются как малые величины, квадратами которых в уравнениях движения можно пренебрегать. В таком случае

Тогда для малых отклонений

В этом случае из третьего уравнения, отбрасывая бесконечно малые величины, получим

или

Применим теорему об изменении момента количества движения относительно оси  Для суммы моментов сил относительно оси
Для суммы моментов сил относительно оси  принимая во внимание, что изменением координаты
принимая во внимание, что изменением координаты  можно пренебречь, получим приближенное значение
можно пренебречь, получим приближенное значение

Обозначая через  угол, образованный вертикальной плоскостью качания маятника с плоскостью
угол, образованный вертикальной плоскостью качания маятника с плоскостью  получим
получим

где

тогда

Теорема об изменении момента количества движения дает

или

Если в начальный момент  то постоянная интегрирования обращается в нуль, и
то постоянная интегрирования обращается в нуль, и

Интегрируя это уравнение, получим

т. е. угол Ф изменяется с течением времени с постоянной скоростью.
Обозначая через Т время полного оборота плоскости качания маятника, т. е. время, за которое угол Ф изменится на величину  будем иметь
будем иметь

Здесь со — угловая скорость вращения Земли, так что

поэтому

На полюсе при  На экваторе
На экваторе  и время полного оборота
и время полного оборота  т. е. плоскость качания маятника практически не вращается. Вращение плоскости качания маятника впервые обнаружил в 1851 г. Леон Фуко (1819—1868) в своих знаменитых опытах в Пантеоне. Длина нити была равна
т. е. плоскость качания маятника практически не вращается. Вращение плоскости качания маятника впервые обнаружил в 1851 г. Леон Фуко (1819—1868) в своих знаменитых опытах в Пантеоне. Длина нити была равна  продолжительность простого колебания 16 сек, широта местности
продолжительность простого колебания 16 сек, широта местности  равна
равна  Время полного оборота плоскости качания, полученное на основании вычислений, оказалось равным 41 час 47 мин, что и было подтверждено опытами. Плоскость качания маятника Фуко, установленного в здании Исаакиевского собора в Ленинграде (длина маятника 98 м, вес 60 кг, амплитуда колебаний 5 м, период колебаний около 20 сек,
Время полного оборота плоскости качания, полученное на основании вычислений, оказалось равным 41 час 47 мин, что и было подтверждено опытами. Плоскость качания маятника Фуко, установленного в здании Исаакиевского собора в Ленинграде (длина маятника 98 м, вес 60 кг, амплитуда колебаний 5 м, период колебаний около 20 сек,  поворачивается за каждый час приблизительно на 13°.
поворачивается за каждый час приблизительно на 13°.
Все приведенные здесь рассуждения носят приближенный характер, так как предполагалось, что длина маятника достаточно
велика, а амплитуда колебаний мала. На практике маятник Фуко обычно отпускают из отклоненного начального положения, так что постоянная интегрирования в уравнении

отлична от нуля. Принимая во внимание, что в начальный момент  получим
получим

откуда

Полагая  преобразуем последнее уравнение к виду
преобразуем последнее уравнение к виду

Величины  являются относительными полярными координатами горизонтальной проекции точки по отношению к системе осей, вращающейся вокруг вертикали с угловой скоростью
являются относительными полярными координатами горизонтальной проекции точки по отношению к системе осей, вращающейся вокруг вертикали с угловой скоростью  Для полного исследования движения здесь необходимо принять во внимание еще интеграл живых сил. Тогда уравнения будут совпадать с уравнениями задачи о движении точки, притягиваемой неподвижным центром с силой, пропорциональной расстоянию точки до центра. Известно, что в таком движении точка описывает эллипс.
Для полного исследования движения здесь необходимо принять во внимание еще интеграл живых сил. Тогда уравнения будут совпадать с уравнениями задачи о движении точки, притягиваемой неподвижным центром с силой, пропорциональной расстоянию точки до центра. Известно, что в таком движении точка описывает эллипс.
From Wikipedia, the free encyclopedia
This article is about the physics experiment and instrument. For the novel by Umberto Eco, see Foucault’s Pendulum.
The Foucault pendulum or Foucault’s pendulum is a simple device named after French physicist Léon Foucault, conceived as an experiment to demonstrate the Earth’s rotation. A long and heavy pendulum suspended from the high roof above a circular area was monitored over an extended time period, showing that its plane of oscillation rotated.
The pendulum was introduced in 1851 and was the first experiment to give simple, direct evidence of the Earth’s rotation. Foucault pendulums today are popular displays in science museums and universities.[1]
Original Foucault pendulum[edit]

A print of the Foucault Pendulum, 1895
The first public exhibition of a Foucault pendulum took place in February 1851 in the Meridian of the Paris Observatory. A few weeks later, Foucault made his most famous pendulum when he suspended a 28-kilogram (62 lb) brass-coated lead bob with a 67-metre long (220 ft) wire from the dome of the Panthéon, Paris. The proper period of the pendulum was approximately 


Foucault explained his results in an 1851 paper entitled Physical demonstration of the Earth’s rotational movement by means of the pendulum, published in the Weekly reports of the sessions of the Academy of Sciences. He wrote that:[2]
…an oscillatory movement of the pendulum mass follows an arc of a circle whose plane is well known, and to which the inertia of matter ensures an unchanging position in space. If these oscillations continue for a certain time, the movement of the earth, which continues to rotate from west to east, will become sensitive in contrast to the immobility of the oscillation plane whose trace on the ground will seem animated by a movement consistent with the apparent movement of the celestial sphere; and if the oscillations could be perpetuated for twenty-four hours, the trace of their plane would then execute an entire revolution around the vertical projection of the point of suspension.
The original bob used in 1851 at the Panthéon was moved in 1855 to the Conservatoire des Arts et Métiers in Paris. A second temporary installation was made for the 50th anniversary in 1902.[3]
During museum reconstruction in the 1990s, the original pendulum was temporarily displayed at the Panthéon (1995), but was later returned to the Musée des Arts et Métiers before it reopened in 2000.[4] On April 6, 2010, the cable suspending the bob in the Musée des Arts et Métiers snapped, causing irreparable damage to the pendulum bob and to the marble flooring of the museum.[5][6] The original, now damaged pendulum bob is displayed in a separate case adjacent to the current pendulum display.
An exact copy of the original pendulum has been operating under the dome of the Panthéon, Paris since 1995.[7]
Explanation of mechanics[edit]

Animation of a Foucault pendulum on the northern hemisphere, with the Earth’s rotation rate and amplitude greatly exaggerated. The green trace shows the path of the pendulum bob over the ground (a rotating reference frame), while in any vertical plane. The actual plane of swing appears to rotate relative to the Earth: sitting astride the bob like a swing, Coriolis fictitious force disappears: observer is in a “free rotational” reference where, according to general relativity, non-Euclidean curved spacetime metrics must be used. The wire should be as long as possible—lengths of 12–30 m (40–100 ft) are common.[8]
At either the Geographic North Pole or Geographic South Pole, the plane of oscillation of a pendulum remains fixed relative to the distant masses of the universe while Earth rotates underneath it, taking one sidereal day to complete a rotation. So, relative to Earth, the plane of oscillation of a pendulum at the North Pole – viewed from above – undergoes a full clockwise rotation during one day; a pendulum at the South Pole rotates counterclockwise.
When a Foucault pendulum is suspended at the equator, the plane of oscillation remains fixed relative to Earth. At other latitudes, the plane of oscillation precesses relative to Earth, but more slowly than at the pole; the angular speed, ω (measured in clockwise degrees per sidereal day), is proportional to the sine of the latitude, φ:
where latitudes north and south of the equator are defined as positive and negative, respectively. A “pendulum day” is the time needed for the plane of a freely suspended Foucault pendulum to complete an apparent rotation about the local vertical. This is one sidereal day divided by the sine of the latitude.[9][10] For example, a Foucault pendulum at 30° south latitude, viewed from above by an earthbound observer, rotates counterclockwise 360° in two days.
Using enough wire length, the described circle can be wide enough that the tangential displacement along the measuring circle of between two oscillations can be visible by eye, rendering the Foucault pendulum a spectacular experiment: for example, the original Foucault pendulum in Panthéon moves circularly, with a 6-metre pendulum amplitude, by about 5 mm each period.

A Foucault pendulum at the North Pole: The pendulum swings in the same plane as the Earth rotates beneath it.

An excerpt from the illustrated supplement of the magazine Le Petit Parisien dated November 2, 1902, on the 50th anniversary of the experiment of Léon Foucault demonstrating the rotation of the earth.
A Foucault pendulum requires care to set up because imprecise construction can cause additional veering which masks the terrestrial effect. As observed by later Nobel laureate Heike Kamerlingh Onnes, who developed a fuller theory of the Foucault pendulum for his doctoral thesis (1879), geometrical imperfection of the system or elasticity of the support wire may cause an interference between two horizontal modes of oscillation, which caused Onnes’ pendulum to go over from linear to elliptic oscillation in an hour.[11] The initial launch of the pendulum is also critical; the traditional way to do this is to use a flame to burn through a thread which temporarily holds the bob in its starting position, thus avoiding unwanted sideways motion (see a detail of the launch at the 50th anniversary in 1902).
Notably, veering of a pendulum was observed already in 1661 by Vincenzo Viviani, a disciple of Galileo, but there is no evidence that he connected the effect with the Earth’s rotation; rather, he regarded it as a nuisance in his study that should be overcome with suspending the bob on two ropes instead of one.
Air resistance damps the oscillation, so some Foucault pendulums in museums incorporate an electromagnetic or other drive to keep the bob swinging; others are restarted regularly, sometimes with a launching ceremony as an added attraction. Besides air resistance (the use of a heavy symmetrical bob is to reduce friction forces, mainly air resistance by a symmetrical and aerodynamic bob) the other main engineering problem in creating a 1-meter Foucault pendulum nowadays is said to be ensuring there is no preferred direction of swing.[12]
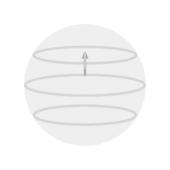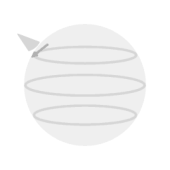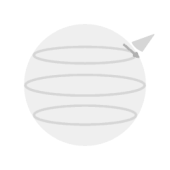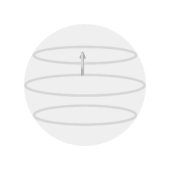
The animation describes the motion of a Foucault pendulum at a latitude of 30°N. The plane of oscillation rotates by an angle of −180° during one day, so after two days, the plane returns to its original orientation.
Precession as a form of parallel transport[edit]

Parallel transport of a vector around a closed loop on the sphere: The angle by which it twists, α, is proportional to the area inside the loop.
In a near-inertial frame moving in tandem with the Earth, but not sharing the rotation of the Earth about its own axis, the suspension point of the pendulum traces out a circular path during one sidereal day.
At the latitude of Paris, 48 degrees 51 minutes north, a full precession cycle takes just under 32 hours, so after one sidereal day, when the Earth is back in the same orientation as one sidereal day before, the oscillation plane has turned by just over 270 degrees. If the plane of swing was north–south at the outset, it is east–west one sidereal day later.
This also implies that there has been exchange of momentum; the Earth and the pendulum bob have exchanged momentum. The Earth is so much more massive than the pendulum bob that the Earth’s change of momentum is unnoticeable. Nonetheless, since the pendulum bob’s plane of swing has shifted, the conservation laws imply that an exchange must have occurred.
Rather than tracking the change of momentum, the precession of the oscillation plane can efficiently be described as a case of parallel transport. For that, it can be demonstrated, by composing the infinitesimal rotations, that the precession rate is proportional to the projection of the angular velocity of Earth onto the normal direction to Earth, which implies that the trace of the plane of oscillation will undergo parallel transport. After 24 hours, the difference between initial and final orientations of the trace in the Earth frame is α = −2π sin φ, which corresponds to the value given by the Gauss–Bonnet theorem. α is also called the holonomy or geometric phase of the pendulum. When analyzing earthbound motions, the Earth frame is not an inertial frame, but rotates about the local vertical at an effective rate of 2π sin φ radians per day. A simple method employing parallel transport within cones tangent to the Earth’s surface can be used to describe the rotation angle of the swing plane of Foucault’s pendulum.[13][14]
From the perspective of an Earth-bound coordinate system (the measuring circle and spectator are Earth-bounded, also if terrain reaction to Coriolis force is not perceived by spectator when he moves), using a rectangular coordinate system with its x-axis pointing east and its y-axis pointing north, the precession of the pendulum is due to the Coriolis force (other fictitious forces as gravity and centrifugal force have not direct precession component, Euler’s force is low because Earth’s rotation speed is nearly constant). Consider a planar pendulum with constant natural frequency ω in the small angle approximation. There are two forces acting on the pendulum bob: the restoring force provided by gravity and the wire, and the Coriolis force (the centrifugal force, opposed to the gravitational restoring force, can be neglected). The Coriolis force at latitude φ is horizontal in the small angle approximation and is given by
where Ω is the rotational frequency of Earth, Fc,x is the component of the Coriolis force in the x-direction and Fc,y is the component of the Coriolis force in the y-direction.
The restoring force, in the small-angle approximation and neglecting centrifugal force, is given by

Graphs of precession period and precession per sidereal day vs latitude. The sign changes as a Foucault pendulum rotates anticlockwise in the Southern Hemisphere and clockwise in the Northern Hemisphere. The example shows that one in Paris precesses 271° each sidereal day, taking 31.8 hours per rotation.
Using Newton’s laws of motion this leads to the system of equations
Switching to complex coordinates z = x + iy, the equations read
To first order in Ω/ω this equation has the solution
If time is measured in days, then Ω = 2π and the pendulum rotates by an angle of −2π sin φ during one day.
[edit]

The device described by Wheatstone.
Many physical systems precess in a similar manner to a Foucault pendulum. As early as 1836, the Scottish mathematician Edward Sang contrived and explained the precession of a spinning top. In 1851, Charles Wheatstone[15] described an apparatus that consists of a vibrating spring that is mounted on top of a disk so that it makes a fixed angle φ with the disk. The spring is struck so that it oscillates in a plane. When the disk is turned, the plane of oscillation changes just like the one of a Foucault pendulum at latitude φ.
Similarly, consider a nonspinning, perfectly balanced bicycle wheel mounted on a disk so that its axis of rotation makes an angle φ with the disk. When the disk undergoes a full clockwise revolution, the bicycle wheel will not return to its original position, but will have undergone a net rotation of 2π sin φ.
Foucault-like precession is observed in a virtual system wherein a massless particle is constrained to remain on a rotating plane that is inclined with respect to the axis of rotation.[16]
Spin of a relativistic particle moving in a circular orbit precesses similar to the swing plane of Foucault pendulum. The relativistic velocity space in Minkowski spacetime can be treated as a sphere S3 in 4-dimensional Euclidean space with imaginary radius and imaginary timelike coordinate. Parallel transport of polarization vectors along such sphere gives rise to Thomas precession, which is analogous to the rotation of the swing plane of Foucault pendulum due to parallel transport along a sphere S2 in 3-dimensional Euclidean space.[17]
In physics, the evolution of such systems is determined by geometric phases.[18][19] Mathematically they are understood through parallel transport.
Foucault pendulums around the world[edit]
There are numerous Foucault pendulums at universities, science museums, and the like throughout the world. The United Nations General Assembly Building at the United Nations headquarters in New York City has one. The Oregon Convention Center pendulum is claimed to be the largest, its length is approximately 27 m (89 ft),[20][21] however, there are larger ones listed in the article, such as the one in Gamow Tower at the University of Colorado (39.3 m). There used to be much longer pendulums, such as the 98 m (322 ft) pendulum in Saint Isaac’s Cathedral, Saint Petersburg, Russia.[22][23]
South Pole[edit]
The experiment has also been carried out at the South Pole, where it was assumed that the rotation of the Earth would have maximum effect.[24][25] A pendulum was installed in a six-story staircase of a new station under construction at the Amundsen-Scott South Pole Station. It had a length of 33 m (108 ft) and the bob weighed 25 kg (55 lb). The location was ideal: no moving air could disturb the pendulum. The researchers confirmed about 24 hours as the rotation period of the plane of oscillation.
See also[edit]
- Absolute rotation
- Coriolis effect
- Earth’s rotation
- Eötvös experiment
- Inertial frame
- Lariat chain
- Precession
References[edit]
- ^ Oprea, John (1995). “Geometry and the Foucault Pendulum”. Amer. Math. Monthly. 102 (6): 515–522. doi:10.2307/2974765. JSTOR 2974765. Archived from the original on 2015-04-02.
- ^ wikisource:Démonstration physique du mouvement de rotation de la Terre au moyen du pendule “Si donc ces oscillations se perpétuent pendant un certain temps, le mouvement de la terre, qui ne cesse de tourner d’occident en orient, deviendra sensible par le contraste de l’immobilité du plan d’oscillation dont la trace sur le sol semblera animée d’un mouvement conforme au mouvement apparent de la sphère céleste ; et si les oscillations pouvaient se perpétuer pendant vingt-quatre heures, la trace de leur plan exécuterait dans le même temps une révolution entière autour de la projection verticale du point de suspension.”
- ^ “The Pendulum of Foucault of the Panthéon. Ceremony of inauguration by M. Chaumié, minister of the state education, burnt the wire of balancing, to start the pendulum. 1902”. Paris en images. Archived from the original on 2014-08-21.
- ^ Kissell, Joe (November 8, 2004). “Foucault’s Pendulum: Low-tech proof of Earth’s rotation”. Interesting thing of the day. Archived from the original on March 12, 2012. Retrieved March 21, 2012.
- ^ Thiolay, Boris (April 28, 2010). “Le pendule de Foucault perd la boule”. L’Express (in French). Archived from the original on July 10, 2010.
- ^ “Foucault’s pendulum is sent crashing to Earth”. Times Higher Education. 13 May 2010. Archived from the original on 20 March 2012. Retrieved March 21, 2012.
- ^ “Foucault’s Pendulum and the Paris Pantheon”. Atlas Obscura. Archived from the original on January 12, 2018. Retrieved January 12, 2018.
- ^ “Foucault Pendulum”. Smithsonian Encyclopedia. Retrieved September 2, 2013.
- ^ “Pendulum day”. Glossary of Meteorology. American Meteorological Society. Archived from the original on 2007-08-17.
- ^ Daliga, K.; Przyborski, M.; Szulwic, J. “Foucault’s Pendulum. Uncomplicated Tool In The Study Of Geodesy And Cartography”. library.iated.org. Archived from the original on 2016-03-02. Retrieved 2015-11-02.
- ^ Sommeria, Joël (1 November 2017). “Foucault and the rotation of the Earth”. Comptes Rendus Physique. 18 (9): 520–525. Bibcode:2017CRPhy..18..520S. doi:10.1016/j.crhy.2017.11.003.
- ^ “A Short, Driven, Foucault Pendulum”. Archived from the original on 2009-03-31.
- ^ Somerville, W. B. (1972). “The Description of Foucault’s Pendulum”. Quarterly Journal of the Royal Astronomical Society. 13: 40. Bibcode:1972QJRAS..13…40S.
- ^ Hart, John B.; Miller, Raymond E.; Mills, Robert L. (1987). “A simple geometric model for visualizing the motion of a Foucault pendulum”. American Journal of Physics. 55 (1): 67–70. Bibcode:1987AmJPh..55…67H. doi:10.1119/1.14972.
- ^ Charles Wheatstone Wikisource: “Note relating to M. Foucault’s new mechanical proof of the Rotation of the Earth”, pp. 65–68.
- ^ Bharadhwaj, Praveen (2014). “Foucault precession manifested in a simple system”. arXiv:1408.3047 [physics.pop-ph].
- ^ Krivoruchenko, M. I. (2009). “Rotation of the swing plane of Foucault’s pendulum and Thomas spin precession: Two faces of one coin”. Phys. Usp. 52 (8): 821–829. arXiv:0805.1136. Bibcode:2009PhyU…52..821K. doi:10.3367/UFNe.0179.200908e.0873. S2CID 118449576.
- ^ “Geometric Phases in Physics”, eds. Frank Wilczek and Alfred Shapere (World Scientific, Singapore, 1989).
- ^ L. Mangiarotti, G. Sardanashvily, Gauge Mechanics (World Scientific, Singapore, 1998)
- ^ “Kristin Jones – Andrew Ginzel”. Retrieved 5 May 2018.
- ^ “LTW Automation Products”. ltwautomation.net. Archived from the original on 29 April 2016. Retrieved 5 May 2018.
- ^ “The first Foucault pendulum in Russia, beyond the Arctic Circle”. 2018-06-14. Archived from the original on 2019-03-21. Retrieved 2019-03-21.
- ^ Great Soviet Encyclopedia
- ^ Johnson, George (September 24, 2002). “Here They Are, Science’s 10 Most Beautiful Experiments”. The New York Times. Archived from the original on May 31, 2012. Retrieved September 20, 2012.
- ^ Baker, G. P. (2011). Seven Tales of the Pendulum. Oxford University Press. p. 388. ISBN 978-0-19-958951-7.
Further reading[edit]
- Arnold, V.I. (1989). Mathematical Methods of Classical Mechanics. Springer. p. 123. ISBN 978-0-387-96890-2.
- Marion, Jerry B.; Thornton, Stephen T. (1995). Classical dynamics of particles and systems (4th ed.). Brooks Cole. pp. 398–401. ISBN 978-0-03-097302-4.
External links[edit]
- Wolfe, Joe, “A derivation of the precession of the Foucault pendulum”.
- “The Foucault Pendulum”, derivation of the precession in polar coordinates.
- “The Foucault Pendulum” By Joe Wolfe, with film clip and animations.
- “Foucault’s Pendulum” by Jens-Peer Kuska with Jeff Bryant, Wolfram Demonstrations Project: a computer model of the pendulum allowing manipulation of pendulum frequency, Earth rotation frequency, latitude, and time.
- “Webcam Kirchhoff-Institut für Physik, Universität Heidelberg”.
- California academy of sciences, CA Archived 2016-05-25 at the Portuguese Web Archive Foucault pendulum explanation, in friendly format
- Foucault pendulum model Exposition including a tabletop device that shows the Foucault effect in seconds.
- Foucault, M. L., Physical demonstration of the rotation of the Earth by means of the pendulum, Franklin Institute, 2000, retrieved 2007-10-31. Translation of his paper on Foucault pendulum.
- Tobin, William. “The Life and Science of Léon Foucault”.
- Bowley, Roger (2010). “Foucault’s Pendulum”. Sixty Symbols. Brady Haran for University of Nottingham.
- Pendolo nel Salone The Foucault Pendulum inside Palazzo della Ragione in Padova, Italy
- Chessin, A. S. (1895). “On Foucault’s Pendulum”. Am. J. Math. 17 (1): 81–88. doi:10.2307/2369710. JSTOR 2369710.
- MacMillan, William Duncan (1915). “On Foucault’s Pendulum”. Am. J. Math. 37 (1): 95–106. doi:10.2307/2370259. JSTOR 2370259. S2CID 123717776.
- Somerville, W. B. (1972). “The description of Foucault’s pendulum”. Q. J. R. Astron. Soc. 13: 40–62. Bibcode:1972QJRAS..13…40S.
- Braginsky, Vladimir B.; Polnarev, Aleksander G.; Thorne, Kip S. (1984). “Foucault Pendulum at the South Pole: Proposal For an Experiment to Detect the Earth’s General Relativistic Gravitomagnetic Field” (PDF). Phys. Rev. Lett. 53 (9): 863. Bibcode:1984PhRvL..53..863B. doi:10.1103/PhysRevLett.53.863.
- Crane, H. Richard (1995). “Foucault pendulum “wall clock”“. Am. J. Phys. 63 (1): 33–39. Bibcode:1995AmJPh..63…33C. doi:10.1119/1.17765.
- Hard, John B.; Miller, Raymond E. (1987). “A simple geometric model for visualizing the motion of a Foucault pendulum”. Am. J. Phys. 55 (1): 67. Bibcode:1987AmJPh..55…67H. doi:10.1119/1.14972.
- Das, U.; Talukdar, B.; Shamanna, J. (2002). “Indirect Analytic Representation of Foucault’s Pendulum”. Czechoslov. J. Phys. 52 (12): 1321–1327. Bibcode:2002CzJPh..52.1321D. doi:10.1023/A:1021819627736. S2CID 118592240.
- Salva, Horacio R.; Benavides, Rubén E.; Perez, Julio C.; Cuscueta, Diego J. (2010). “A Foucault’s pendulum design”. Rev. Sci. Instrum. 81 (11): 115102–115102–4. Bibcode:2010RScI…81k5102S. doi:10.1063/1.3494611. PMID 21133496.
- Daliga, K.; Przyborski, M.; Szulwic, J. (2015). “Foucault’s Pendulum. Uncomplicated Tool in the Study of Geodesy and Cartography”. EDULEARN15 Proceedings – 7th International Conference on Education and New Learning Technologies, Barcelona, Spain. ISBN 978-84-606-8243-1.

ganst
+21
Решено
10 лет назад
Физика
1 – 4 классы
маятник фуко в исаакиевском соборе в петербурге совершил 3 колебания за 1 минуту. определите длину маятника
Смотреть ответ
1
Ответ проверен экспертом
3
(30 оценок)
70

wkw
10 лет назад
Светило науки – 474 ответа – 7292 помощи
дано n=3
t=1мин=60 с
найти l
решение
T=t/n
T=60c/3=20c
Т=2*пи*корень (lg)
g=9.8мс^2
выразим l
T^2=4*Пи^2*lg
отсюда l=T^2*g/4пи^2
l=20c^2*9.8м/с^2/4*3,14^2=99м, если g считать 10м/с^2, то длина равна 100м
(30 оценок)
https://vashotvet.com/task/123848

![{displaystyle Omega _{P}approx 15left[1-{frac {3}{8}}{Bigl (}{frac {a}{l}}{Bigr )}^{2}right]sin phi ,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/35caafb25301005cc1d84e21765abde1ef87975e)









