
{mu = dfrac{F_{тр}}{mg}}
Ускорение свободного падения g
Приводим 2 варианта нахождения коэффициента трения – зная силу трения и массу тела или зная угол наклона. Для обоих вариантов вы найдете удобные калькуляторы и формулы для расчета.
Коэффициент трения представляет собой безразмерную скалярную величину, которая равна отношению силы трения между двумя телами и силы, прижимающей их друг к другу, во время или в начале скольжения.
Коэффициент трения чаще всего обозначают греческой буквой µ («мю»).
Следует помнить, что коэффициент трения (μ) величина безразмерная, то есть не имеет единицы измерения.
Коэффициент трения зависит от качества обработки трущихся поверхностей, скорости движения тел относительно друг друга и материала соприкасающихся поверхностей. В большинстве случаев коэффициент трения находится в пределах от 0,1 до 0,5 (см. таблицу).
Содержание:
- калькулятор коэффициента трения
- формула коэффициента трения через силу трения и массу
- формула коэффициента трения через угол наклона
- таблица коэффициентов трения
- примеры задач
Формула коэффициента трения через силу трения и массу
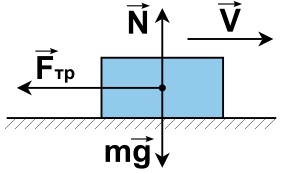
mu = dfrac{F_{тр}}{mg}
Fтр – сила трения
m – масса тела
g – ускорение свободного падения (в большинстве задач можно принять g=9.81 м/с²)
Формула коэффициента трения через угол наклона

mu = tg(alpha)
α – угол наклона
Таблица коэффициентов трения скольжения для разных пар материалов
| Трущиеся материалы (при сухих поверхностях) | Коэффициенты трения | |
|---|---|---|
| покоя | при движении | |
| Резина по сухому асфальту | 0,95-1,0 | 0,5-0,8 |
| Резина по влажному асфальту | 0,25-0,75 | |
| Алюминий по алюминию | 0,94 | |
| Бронза по бронзе | 0,20 | |
| Бронза по чугуну | 0,21 | |
| Дерево по дереву (в среднем) | 0,65 | 0,33 |
| Дерево по камню | 0,46-0,60 | |
| Дуб по дубу (вдоль волокон) | 0,62 | 0,48 |
| Дуб по дубу (перпендикулярно волокнам) | 0,54 | 0,34 |
| Железо по железу | 0,15 | 0,14 |
| Железо по чугуну | 0,19 | 0,18 |
| Железо по бронзе (слабая смазка) | 0,19 | 0,18 |
| Канат пеньковый по деревянному барабану | 0,40 | |
| Канат пеньковый по железному барабану | 0,25 | |
| Каучук по дереву | 0,80 | 0,55 |
| Каучук по металлу | 0,80 | 0,55 |
| Кирпич по кирпичу (гладко отшлифованные) | 0,5-0,7 | |
| Колесо со стальным бандажем по рельсу | 0,16 | |
| Лед по льду | 0,05-0,1 | 0,028 |
| Метал по аботекстолиту | 0,35-0,50 | |
| Метал по дереву (в среднем) | 0,60 | 0,40 |
| Метал по камню (в среднем) | 0,42-0,50 | |
| Метал по металу (в среднем) | 0,18-0,20 | |
| Медь по чугуну | 0,27 | |
| Олово по свинцу | 2,25 | |
| Полозья деревянные по льду | 0,035 | |
| Полозья обитые железом по льду | 0,02 | |
| Резина (шина) по твердому грунту | 0,40-0,60 | |
| Резина (шина) по чугуну | 0,83 | 0,8 |
| Ремень кожаный по деревянному шкиву | 0,50 | 0,30-0,50 |
| Ремень кожаный по чугунному шкиву | 0,30-0,50 | 0,56 |
| Сталь по железу | 0,19 | |
| Сталь(коньки) по льду | 0,02-0,03 | 0,015 |
| Сталь по райбесту | 0,25-0,45 | |
| Сталь по стали | 0,15-0,25 | 0,09 (ν = 3 м/с)
0,03 (ν = 27 м/с) |
| Сталь по феродо | 0,25-0,45 | |
| Точильный камень (мелкозернистый) по железу | 1 | |
| Точильный камень (мелкозернистый) по стали | 0,94 | |
| Точильный камень (мелкозернистый) по чугуну | 0,72 | |
| Чугун по дубу | 0,65 | 0,30-0,50 |
| Чугун по райбесту | 0,25-0,45 | |
| Чугун по стали | 0,33 | 0,13 (ν = 20 м/с) |
| Чугун по феродо | 0,25-0,45 | |
| Чугун по чугуну | 0,15 |
Примеры задач на нахождение коэффициента трения
Задача 1
Найдите коэффициент трения между полом и ящиком массой 20 кг, который равномерно двигают с силой 50 Н.
Решение
Для решения задачи воспользуемся формулой.
mu = dfrac{F_{тр}}{mg} = dfrac{50}{20 cdot 9.81} = dfrac{50}{196.2} approx 0.25484
Ответ: approx 0.25484
С помощью калькулятора удобно проверить ответ.
Задача 2
Найдите коэффициент трения если угол наклона 30°.
Решение
Для решения этой задачи воспользуемся второй формулой.
mu = tg(alpha) = tg(30°) approx 0.57735
Ответ: approx 0.57735
Проверим полученный ответ с помощью калькулятора .
Трение – это процесс взаимодействия тел между собой при движении этих тел относительно друг друга. А сила трения – это та сила, которая возникает в месте соприкосновения этих тел. Почему возникают силы трения?
- Шероховатость соприкасающихся поверхностей.
- Взаимное притяжение молекул, находящихся на поверхности этих тел.
Главной характеристикой трения является коэффициент трения мю (греческая буква мю). При движении одного тела по другому сила трения F равна произведению мю на N, F=мю* N. Коэффициент трения мю – основная характеристика трения. Мю определяется материалами, из которых сделаны тела. N – сила нормального давления одного тела на другое.
Но в школьной физике не проходят так называемого закона Амонтона — Кулона с учетом адгезии. Для большинства пар материалов коэффициент трения мю не превышает 1 (обычные значения от 0,1 до 0,5). Если же мю>1, то это означает, что появляется еще и сила адгезии. Адгезия в переводе с латинского языка adhaesio — прилипание. Это сцепление поверхностей разнородных тел. Она возникает из-за взаимодействия молекул или атомов этих тел. Это Вандер-Вальсовские и полярные силы, иногда даже образуются химические связи на поверхности между двумя телами и проникновение атомов этих двух веществ из одного тела во второе.
Это в определенной степени напоминает процессы склеивания, сварки, нанесения покрытий.
Если коэффициент трения мю>1, то это значит, что между контактирующими материалами имеется сила адгезии. Попробуйте бросить пластилин в стенку, он сразу прилипнет к стенке, то есть здесь мю>1. А почему прилипнет? Быстро возникают силы притяжения между атомами (или молекулами) пластилина и стенки. Можно и два одинаковых металлических бруска так хорошо и гладко отполировать, а потом положить один на другой, что у вас не хватит сил, чтобы не только двигать один кусок по другому, но и оторвать их друг от друга.
Физика, 10 класс
Урок 10. Силы трения
Перечень вопросов, рассматриваемых на уроке:
- Сухое и жидкое (вязкое) трение.
- Максимальная сила трения покоя.
- Формула для вычисления силы трения скольжения.
- Особенности сил сопротивления при движении твердых тел в жидкостях и газах.
- Формулы вычисления сил сопротивления при движении твердых тел в жидкостях и газах.
Глоссарий по теме:
Сухое трение – трение, возникающее при соприкосновении двух твёрдых тел при отсутствии между ними жидкой или газообразной прослойки.
Сила трения покоя – сила трения, действующая между двумя телами, неподвижными относительно друг друга.
Максимальная сила трения покоя – наибольшее значение силы трения, при котором скольжение еще не наступает.
Сила трения скольжения – сила, возникающая между соприкасающимися телами при их относительном движении.
Трение качения – сопротивление движению, возникающее при перекатывании тел друг по другу т.е. сопротивление качению одного тела (катка) по поверхности другого
Основная и дополнительная литература по теме урока:
- Мякишев Г.Я., Буховцев Б.Б., Сотский Н.Н. Физика.10 класс. Учебник для общеобразовательных организаций М.: Просвещение, 2017. – С. 113 – 122.
- Парфентьева Н. А. Сборник задач по физике. 10-11 классы. Базовый уровень.
Открытые электронные ресурсы:
http://kvant.mccme.ru/1978/05/kuda_napravlena_sila_treniya.htm
http://kvant.mccme.ru/1985/10/trenie_vrednoe_poleznoe_intere.htm
Теоретический материал для самостоятельного изучения
Трение – физическое явление, сопровождающее всякое движение на Земле. При любом механическом движении тела соприкасаются либо друг с другом, либо с окружающей их сплошной жидкой или газообразной средой. В результате соприкосновения возникает сила трения, которая препятствует движению. Трение может быть полезно, и тогда мы стремимся его увеличить. В случаях, когда трение вредно, принимаются меры для его уменьшения.
История открытия. Свой вклад в попытки объяснить природу трения внесли многие ученые, начиная с Аристотеля, Леонардо да Винчи, Амонтона, Леонарда Эйлера, Кулона. Дальнейший вклад в теорию трения сделали Майер, Джоуль, Гельмгольц, Кузнецов, Дерягин, Томлинсон, Рейнольдс, Штрибек, Боуден и другие.
Различают следующие виды трения:
- сухое;
- жидкое (вязкое).
Сухое трение бывает трех видов:
- трение покоя;
- трение скольжения;
- трение качения.
Причины возникновения силы трения:
- шероховатость поверхностей соприкасающихся тел.
- взаимное притяжение молекул соприкасающихся тел.
Сухое трение − трение, возникающее при соприкосновении двух твердых тел при отсутствии между ними жидкой или газообразной прослойки. Силы сухого трения всегда направлены по касательной к соприкасающимся поверхностям.
Сухое трение, возникающее при относительном покое тел, называют трением покоя.
Сухое трение, возникающее при относительном движении тел, называют трением скольжения.
Трение качения возникает, когда одно тело катится по поверхности другого тела.
Закон, выражающий зависимость максимального значения модуля силы трения покоя от модуля силы нормальной реакции опоры впервые экспериментально установил французский военный инженер и учёный-физик Шарль Огюстен де Кулон. Согласно этому закону, максимальное значение модуля силы трения покоя прямо пропорционально модулю силы нормальной реакции опоры
Fтр.макс = µN,
где Fтр.макс – модуль максимальной силы трения покоя, µ- коэффициент пропорциональности, называемый коэффициентом трения покоя.
Коэффициент трения µ характеризует обе трущиеся поверхности и зависит не только от материала этих поверхностей, но и от качества их обработки. Коэффициент трения определяется экспериментально.
Трение скольжения. Сила трения скольжения также направлена вдоль поверхности соприкосновения тел, но в отличие от силы трения покоя, которая противоположна внешней силе, стремящейся сдвинуть тело, сила трения скольжения всегда направлена противоположно относительной скорости. Модуль силы трения скольжения, как и максимальной силы трения покоя, тоже пропорционален прижимающей силе, а значит, нормальной силе реакции опоры:

При не слишком больших относительных скоростях движения сила трения скольжения мало отличается от максимальной силы трения покоя. Поэтому приближенно можно считать ее постоянной и равной максимальной силе трения покоя:
Fтр ≈ Fтр.макс = µN.
Важно! Сила трения зависит от относительной скорости движения тел. В этом ее главное отличие от сил тяготения и упругости, зависящих только от расстояний.
При движении твердого тела в жидкости или газе возникает силa жидкого (вязкого) трения. Сила жидкого трения значительно меньше силы сухого трения. Эта сила направлена против скорости тела относительно среды и тормозит движение.
Главная особенность силы сопротивления состоит в том, что она появляется только при наличии относительного движения тела и окружающей среды. Сила трения покоя в жидкостях и газах полностью отсутствует. Поэтому усилием рук можно сдвинуть тяжелую баржу в воде, а сдвинуть поезд усилием рук невозможно.
Модуль силы сопротивления Fc зависит от размеров, формы и состояния поверхности тела, свойств среды (жидкости или газа), в которой тело движется, и, наконец, от относительной скорости движения тела и среды.
Примерный характер зависимости модуля силы сопротивления от модуля относительной скорости тела показан на рисунке

При относительной скорости, равной нулю, сила сопротивления не действует на тело (Fc=0). С увеличением относительной скорости сила сопротивления сначала растет медленно, а затем все быстрее и быстрее. При малых скоростях движения силу сопротивления можно считать прямо пропорциональной скорости движения тела относительно среды:
Fc = k1v, (1)
где k1– коэффициент сопротивления, зависящий от формы, размеров, состояния поверхности тела и свойств среды – ее вязкости.
Вычислить коэффициент k1 теоретически для тел сколько-нибудь сложной формы не представляется возможным, его определяют опытным путем.
При больших скоростях относительного движения сила сопротивления пропорциональна квадрату скорости:
Fc = k2v2, (2)
где k2 – коэффициент сопротивления, отличный от k1.
Только опытным путём можно определить, какая из формул – (1) или (2) – подходит для использования в конкретной практической задаче.
Итак, основными особенностями силы сопротивления, действующей на тело, являются:
1) отсутствие силы трения покоя; 2) зависимость от относительной скорости движения.
Примеры и разбор решения заданий
1. Какая сила не позволяет человеку сдвинуть с места дом?
- Силы трения скольжения;
- сила трения покоя;
- сила тяжести.
Ответ: 2) Сила трения покоя.
2. Деревянный ящик равномерно движется по поверхности длинного стола. Сила давления ящика на поверхность равна 30 Н, сила трения 6 Н. Найдите коэффициент трения скольжения.
Решение.
Воспользуемся формулой, которая связывает силу давления на плоскость, силу трения и коэффициент трения Fтр = µP. Из этой формулы легко получить формулу для расчёта коэффициента трения µ = Fтр / P. Подставляя в неё численные значения, получаем:
µ = Fтр / P = 6Н/30Н = 0,2.
Ответ: 0,2.
3. Кубик из детского конструктора покоится на наклонной плоскости, образующей угол α = 40° с горизонтом. Сила трения покоя равна 0,32 Н. Определите значение силы тяжести, которая действует на кубик.

Решение.
По условию задачи кубик покоится. Следовательно, сумма всех действующих на него сил равна нулю. В проекции на ось, идущей вдоль склона плоскости, получаем соотношение: mg sin α – Fтр = 0. Из него выражаем формулу для расчета силы тяжести, действующей на кубик

Ответ: 0,5 Н.
Максимальный коэффициент – трение
Cтраница 1
Максимальный коэффициент трения у образцов из карбида титана TiC06 при температуре 400 С. Примерно при этой же температуре у карбида титана этого состава наблюдается переход в хрупко-вязкое состояние.
[2]
Валки трехвалковых станов обычно изготовляют из легированного чугуна, обладающего максимальным коэффициентом трения.
[4]
Ремни должны обладать высокой прочностью при переменных напряжениях, износостойкостью, максимальным коэффициентом трения на рабочих поверхностях, минимальной изгиб-ной жесткостью.
[5]
Согласно уравнению (4.34) и данным рис. 4.22, скорость скольжения, соответствующая максимальному коэффициенту трения, увеличивается с увеличением температуры.
[7]
В ряде случаев в муфтах сцепления для увеличения износостойкости элементов, повышения стабильности максимального коэффициента трения и плавности их включения применяют смазочные материалы. Шероховатость поверхности трения элементов муфт не должна быть высокой из соображений их износостойкости. Более подробные сведения о выборе шероховатости поверхностей трения муфт будут приведены ниже.
[8]
Оптимальный температурный режим в зоне сжатия должен обеспечить минимальный коэффициент трения полимера о червяк и максимальный коэффициент трения о внутреннюю поверхность цилиндра. В зоне сжатия из расплава полимера удаляется содержащийся в нем воздух. Последняя зона, назначением которой является подача расплава в экструзионную головку, действует как нагнетательный насос.
[9]
Если для соединения с открытым гнездом требуется резина с большим значением высокоэластического модуля, с высокой поверхностной активностью и максимальным коэффициентом трения, то для соединения с закрытым гнездом, наоборот, резину следует выбирать с учетом наименьших значений модуля, коэффициента трения и поверхностной активности. Если прокладка монтируется в закрытое гнездо с натягом по наружному диаметру, то выбор резины практически не зависит от указанных параметров.
[10]
Конструкция подшипников стана непосредственно влияет на величину удельного расхода энергии на чистую прокатку. Максимальный коэффициент трения имеют подшипники с металлическими вкладышами.
[12]
Между двумя одинаковыми брусками квадратного сечения, лежащими на горизонтальной плоскости, вставлен клин такой же массы, но с сечением в виде равностороннего треугольника. При каком максимальном коэффициенте трения брусков о плоскость они начнут разъезжаться.
[13]
Это можно объяснить тем, что при экспериментах коэффициент трения определяют как частное от деления среднего напряжения трения на удельное давление. СЛ, тогда как величина удельного давления определяется усредненными свойствами по всему объему тюб – Поэтому максимальный коэффициент трения / тахр0т – сл / 2 СГюб может быть больше 0 5 и при всестороннем сжатии.
[14]
При радиальных и наклонных канавках образуется клин из смазочного материала, поэтому коэффициент трения фрикционной пары невысок. Спиральные канавки способствуют повышению коэффициента трения при сравнительно малом износе сопряженной поверхности, но ухудшают условия подвода смазочного материала и охлаждения пары трения. Максимальный коэффициент трения при малом износе и высокой эффективности охлаждения обеспечивается при использовании комбинированных спиральных и радиальных канавок.
[15]
Страницы:
1

Friction between two objects. The blue one has more friction against the sloped surface than the green one.
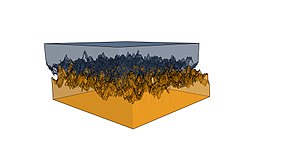
Figure 1: Simulated blocks with fractal rough surfaces, exhibiting static frictional interactions[1]
Friction is the force resisting the relative motion of solid surfaces, fluid layers, and material elements sliding against each other.[2] There are several types of friction:
- Dry friction is a force that opposes the relative lateral motion of two solid surfaces in contact. Dry friction is subdivided into static friction (“stiction”) between non-moving surfaces, and kinetic friction between moving surfaces. With the exception of atomic or molecular friction, dry friction generally arises from the interaction of surface features, known as asperities (see Figure 1).
- Fluid friction describes the friction between layers of a viscous fluid that are moving relative to each other.[3][4]
- Lubricated friction is a case of fluid friction where a lubricant fluid separates two solid surfaces.[5][6][7]
- Skin friction is a component of drag, the force resisting the motion of a fluid across the surface of a body.
- Internal friction is the force resisting motion between the elements making up a solid material while it undergoes deformation.[4]
When surfaces in contact move relative to each other, the friction between the two surfaces converts kinetic energy into thermal energy (that is, it converts work to heat). This property can have dramatic consequences, as illustrated by the use of friction created by rubbing pieces of wood together to start a fire. Kinetic energy is converted to thermal energy whenever motion with friction occurs, for example when a viscous fluid is stirred. Another important consequence of many types of friction can be wear, which may lead to performance degradation or damage to components. Friction is a component of the science of tribology.
Friction is desirable and important in supplying traction to facilitate motion on land. Most land vehicles rely on friction for acceleration, deceleration and changing direction. Sudden reductions in traction can cause loss of control and accidents.
Friction is not itself a fundamental force. Dry friction arises from a combination of inter-surface adhesion, surface roughness, surface deformation, and surface contamination. The complexity of these interactions makes the calculation of friction from first principles impractical and necessitates the use of empirical methods for analysis and the development of theory.
Friction is a non-conservative force – work done against friction is path dependent. In the presence of friction, some kinetic energy is always transformed to thermal energy, so mechanical energy is not conserved.
History
The Greeks, including Aristotle, Vitruvius, and Pliny the Elder, were interested in the cause and mitigation of friction.[8] They were aware of differences between static and kinetic friction with Themistius stating in 350 A.D. that “it is easier to further the motion of a moving body than to move a body at rest”.[8][9][10][11]
The classic laws of sliding friction were discovered by Leonardo da Vinci in 1493, a pioneer in tribology, but the laws documented in his notebooks were not published and remained unknown.[12][13][14][15][16][17] These laws were rediscovered by Guillaume Amontons in 1699[18] and became known as Amonton’s three laws of dry friction. Amontons presented the nature of friction in terms of surface irregularities and the force required to raise the weight pressing the surfaces together. This view was further elaborated by Bernard Forest de Bélidor[19] and Leonhard Euler (1750), who derived the angle of repose of a weight on an inclined plane and first distinguished between static and kinetic friction.[20]
John Theophilus Desaguliers (1734) first recognized the role of adhesion in friction.[21] Microscopic forces cause surfaces to stick together; he proposed that friction was the force necessary to tear the adhering surfaces apart.
The understanding of friction was further developed by Charles-Augustin de Coulomb (1785).[18] Coulomb investigated the influence of four main factors on friction: the nature of the materials in contact and their surface coatings; the extent of the surface area; the normal pressure (or load); and the length of time that the surfaces remained in contact (time of repose).[12] Coulomb further considered the influence of sliding velocity, temperature and humidity, in order to decide between the different explanations on the nature of friction that had been proposed. The distinction between static and dynamic friction is made in Coulomb’s friction law (see below), although this distinction was already drawn by Johann Andreas von Segner in 1758.[12]
The effect of the time of repose was explained by Pieter van Musschenbroek (1762) by considering the surfaces of fibrous materials, with fibers meshing together, which takes a finite time in which the friction increases.
John Leslie (1766–1832) noted a weakness in the views of Amontons and Coulomb: If friction arises from a weight being drawn up the inclined plane of successive asperities, why then isn’t it balanced through descending the opposite slope? Leslie was equally skeptical about the role of adhesion proposed by Desaguliers, which should on the whole have the same tendency to accelerate as to retard the motion.[12] In Leslie’s view, friction should be seen as a time-dependent process of flattening, pressing down asperities, which creates new obstacles in what were cavities before.
Arthur Jules Morin (1833) developed the concept of sliding versus rolling friction. Osborne Reynolds (1866) derived the equation of viscous flow. This completed the classic empirical model of friction (static, kinetic, and fluid) commonly used today in engineering.[13] In 1877, Fleeming Jenkin and J. A. Ewing investigated the continuity between static and kinetic friction.[22]
The focus of research during the 20th century has been to understand the physical mechanisms behind friction. Frank Philip Bowden and David Tabor (1950) showed that, at a microscopic level, the actual area of contact between surfaces is a very small fraction of the apparent area.[14] This actual area of contact, caused by asperities increases with pressure. The development of the atomic force microscope (ca. 1986) enabled scientists to study friction at the atomic scale,[13] showing that, on that scale, dry friction is the product of the inter-surface shear stress and the contact area. These two discoveries explain Amonton’s first law (below); the macroscopic proportionality between normal force and static frictional force between dry surfaces.
Laws of dry friction
The elementary property of sliding (kinetic) friction were discovered by experiment in the 15th to 18th centuries and were expressed as three empirical laws:
- Amontons’ First Law: The force of friction is directly proportional to the applied load.
- Amontons’ Second Law: The force of friction is independent of the apparent area of contact.
- Coulomb’s Law of Friction: Kinetic friction is independent of the sliding velocity.
Dry friction
Dry friction resists relative lateral motion of two solid surfaces in contact. The two regimes of dry friction are ‘static friction’ (“stiction”) between non-moving surfaces, and kinetic friction (sometimes called sliding friction or dynamic friction) between moving surfaces.
Coulomb friction, named after Charles-Augustin de Coulomb, is an approximate model used to calculate the force of dry friction. It is governed by the model:
where
The Coulomb friction 

The force of friction is always exerted in a direction that opposes movement (for kinetic friction) or potential movement (for static friction) between the two surfaces. For example, a curling stone sliding along the ice experiences a kinetic force slowing it down. For an example of potential movement, the drive wheels of an accelerating car experience a frictional force pointing forward; if they did not, the wheels would spin, and the rubber would slide backwards along the pavement. Note that it is not the direction of movement of the vehicle they oppose, it is the direction of (potential) sliding between tire and road.
Normal force
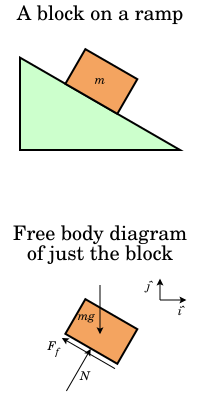
Free-body diagram for a block on a ramp. Arrows are vectors indicating directions and magnitudes of forces. N is the normal force, mg is the force of gravity, and Ff is the force of friction.
The normal force is defined as the net force compressing two parallel surfaces together, and its direction is perpendicular to the surfaces. In the simple case of a mass resting on a horizontal surface, the only component of the normal force is the force due to gravity, where 



The friction coefficient is an empirical (experimentally measured) structural property that depends only on various aspects of the contacting materials, such as surface roughness. The coefficient of friction is not a function of mass or volume. For instance, a large aluminum block has the same coefficient of friction as a small aluminum block. However, the magnitude of the friction force itself depends on the normal force, and hence on the mass of the block.
Depending on the situation, the calculation of the normal force 








If the object is on a tilted surface such as an inclined plane, the normal force from gravity is smaller than 
In general, process for solving any statics problem with friction is to treat contacting surfaces tentatively as immovable so that the corresponding tangential reaction force between them can be calculated. If this frictional reaction force satisfies 

Coefficient of friction
|
This section needs expansion with: explanation of why kinetic friction is always lower. You can help by adding to it. (August 2020) |
The coefficient of friction (COF), often symbolized by the Greek letter µ, is a dimensionless scalar value which equals the ratio of the force of friction between two bodies and the force pressing them together, either during or at the onset of slipping. The coefficient of friction depends on the materials used; for example, ice on steel has a low coefficient of friction, while rubber on pavement has a high coefficient of friction. Coefficients of friction range from near zero to greater than one. The coefficient of friction between two surfaces of similar metals is greater than that between two surfaces of different metals; for example, brass has a higher coefficient of friction when moved against brass, but less if moved against steel or aluminum.[23]
For surfaces at rest relative to each other, 

For surfaces in relative motion 


Arthur Morin introduced the term and demonstrated the utility of the coefficient of friction.[12] The coefficient of friction is an empirical measurement — it has to be measured experimentally, and cannot be found through calculations.[24] Rougher surfaces tend to have higher effective values. Both static and kinetic coefficients of friction depend on the pair of surfaces in contact; for a given pair of surfaces, the coefficient of static friction is usually larger than that of kinetic friction; in some sets the two coefficients are equal, such as teflon-on-teflon.
Most dry materials in combination have friction coefficient values between 0.3 and 0.6. Values outside this range are rarer, but teflon, for example, can have a coefficient as low as 0.04. A value of zero would mean no friction at all, an elusive property. Rubber in contact with other surfaces can yield friction coefficients from 1 to 2. Occasionally it is maintained that μ is always < 1, but this is not true. While in most relevant applications μ < 1, a value above 1 merely implies that the force required to slide an object along the surface is greater than the normal force of the surface on the object. For example, silicone rubber or acrylic rubber-coated surfaces have a coefficient of friction that can be substantially larger than 1.
While it is often stated that the COF is a “material property,” it is better categorized as a “system property.” Unlike true material properties (such as conductivity, dielectric constant, yield strength), the COF for any two materials depends on system variables like temperature, velocity, atmosphere and also what are now popularly described as aging and deaging times; as well as on geometric properties of the interface between the materials, namely surface structure.[1] For example, a copper pin sliding against a thick copper plate can have a COF that varies from 0.6 at low speeds (metal sliding against metal) to below 0.2 at high speeds when the copper surface begins to melt due to frictional heating. The latter speed, of course, does not determine the COF uniquely; if the pin diameter is increased so that the frictional heating is removed rapidly, the temperature drops, the pin remains solid and the COF rises to that of a ‘low speed’ test.[citation needed]
Approximate coefficients of friction
| Materials | Static Friction,  |
Kinetic/Sliding Friction, 
|
|||
|---|---|---|---|---|---|
| Dry and clean | Lubricated | Dry and clean | Lubricated | ||
| Aluminium | Steel | 0.61[25] | 0.47[25] | ||
| Aluminium | Aluminium | 1.05–1.35[25] | 0.3[25] | 1.4[25]–1.5[26] | |
| Gold | Gold | 2.5[26] | |||
| Platinum | Platinum | 1.2[25] | 0.25[25] | 3.0[26] | |
| Silver | Silver | 1.4[25] | 0.55[25] | 1.5[26] | |
| Alumina ceramic | Silicon nitride ceramic | 0.004 (wet)[27] | |||
| BAM (Ceramic alloy AlMgB14) | Titanium boride (TiB2) | 0.04–0.05[28] | 0.02[29][30] | ||
| Brass | Steel | 0.35–0.51[25] | 0.19[25] | 0.44[25] | |
| Cast iron | Copper | 1.05[25] | 0.29[25] | ||
| Cast iron | Zinc | 0.85[25] | 0.21[25] | ||
| Concrete | Rubber | 1.0 | 0.30 (wet) | 0.6–0.85[25] | 0.45–0.75 (wet)[25] |
| Concrete | Wood | 0.62[25][31] | |||
| Copper | Glass | 0.68[32] | 0.53[32] | ||
| Copper | Steel | 0.53[32] | 0.36[25][32] | 0.18[32] | |
| Glass | Glass | 0.9–1.0[25][32] | 0.005–0.01[32] | 0.4[25][32] | 0.09–0.116[32] |
| Human synovial fluid | Human cartilage | 0.01[33] | 0.003[33] | ||
| Ice | Ice | 0.02–0.09[34] | |||
| Polyethene | Steel | 0.2[25][34] | 0.2[25][34] | ||
| PTFE (Teflon) | PTFE (Teflon) | 0.04[25][34] | 0.04[25][34] | 0.04[25] | |
| Steel | Ice | 0.03[34] | |||
| Steel | PTFE (Teflon) | 0.04[25]−0.2[34] | 0.04[25] | 0.04[25] | |
| Steel | Steel | 0.74[25]−0.80[34] | 0.005–0.23[32][34] | 0.42–0.62[25][32] | 0.029–0.19[32] |
| Wood | Metal | 0.2–0.6[25][31] | 0.2 (wet)[25][31] | 0.49[32] | 0.075[32] |
| Wood | Wood | 0.25–0.62[25][31][32] | 0.2 (wet)[25][31] | 0.32–0.48[32] | 0.067–0.167[32] |
Under certain conditions some materials have very low friction coefficients. An example is (highly ordered pyrolytic) graphite which can have a friction coefficient below 0.01.[35]
This ultralow-friction regime is called superlubricity.
Static friction

When the mass is not moving, the object experiences static friction. The friction increases as the applied force increases until the block moves. After the block moves, it experiences kinetic friction, which is less than the maximum static friction.
Static friction is friction between two or more solid objects that are not moving relative to each other. For example, static friction can prevent an object from sliding down a sloped surface. The coefficient of static friction, typically denoted as μs, is usually higher than the coefficient of kinetic friction. Static friction is considered to arise as the result of surface roughness features across multiple length scales at solid surfaces. These features, known as asperities are present down to nano-scale dimensions and result in true solid to solid contact existing only at a limited number of points accounting for only a fraction of the apparent or nominal contact area.[36] The linearity between applied load and true contact area, arising from asperity deformation, gives rise to the linearity between static frictional force and normal force, found for typical Amonton–Coulomb type friction.[37]
The static friction force must be overcome by an applied force before an object can move. The maximum possible friction force between two surfaces before sliding begins is the product of the coefficient of static friction and the normal force: 



An example of static friction is the force that prevents a car wheel from slipping as it rolls on the ground. Even though the wheel is in motion, the patch of the tire in contact with the ground is stationary relative to the ground, so it is static rather than kinetic friction. Upon slipping, the wheel friction changes to kinetic friction. An anti-lock braking system operates on the principle of allowing a locked wheel to resume rotating so that the car maintains static friction.
The maximum value of static friction, when motion is impending, is sometimes referred to as limiting friction,[39]
although this term is not used universally.[3]
Kinetic friction
Kinetic friction, also known as dynamic friction or sliding friction, occurs when two objects are moving relative to each other and rub together (like a sled on the ground). The coefficient of kinetic friction is typically denoted as μk, and is usually less than the coefficient of static friction for the same materials.[40][41] However, Richard Feynman comments that “with dry metals it is very hard to show any difference.”[42]
The friction force between two surfaces after sliding begins is the product of the coefficient of kinetic friction and the normal force: 
New models are beginning to show how kinetic friction can be greater than static friction. Kinetic friction is now understood, in many cases, to be primarily caused by chemical bonding between the surfaces, rather than interlocking asperities;[44] however, in many other cases roughness effects are dominant, for example in rubber to road friction. Surface roughness and contact area affect kinetic friction for micro- and nano-scale objects where surface area forces dominate inertial forces.[45]
The origin of kinetic friction at nanoscale can be explained by thermodynamics.[46] Upon sliding, a new surface forms at the back of a sliding true contact, and existing surface disappears at the front of it. Since all surfaces involve the thermodynamic surface energy, work must be spent in creating the new surface, and energy is released as heat in removing the surface. Thus, a force is required to move the back of the contact, and frictional heat is released at the front.

Angle of friction, θ, when block just starts to slide.
Angle of friction
For the maximum angle of static friction between granular materials, see Angle of repose.
For certain applications, it is more useful to define static friction in terms of the maximum angle before which one of the items will begin sliding. This is called the angle of friction or friction angle. It is defined as:
and thus:
where 
Friction at the atomic level
Determining the forces required to move atoms past each other is a challenge in designing nanomachines. In 2008 scientists for the first time were able to move a single atom across a surface, and measure the forces required. Using ultrahigh vacuum and nearly zero temperature (5 K), a modified atomic force microscope was used to drag a cobalt atom, and a carbon monoxide molecule, across surfaces of copper and platinum.[48]
Limitations of the Coulomb model
The Coulomb approximation follows from the assumptions that: surfaces are in atomically close contact only over a small fraction of their overall area; that this contact area is proportional to the normal force (until saturation, which takes place when all area is in atomic contact); and that the frictional force is proportional to the applied normal force, independently of the contact area. The Coulomb approximation is fundamentally an empirical construct. It is a rule-of-thumb describing the approximate outcome of an extremely complicated physical interaction. The strength of the approximation is its simplicity and versatility. Though the relationship between normal force and frictional force is not exactly linear (and so the frictional force is not entirely independent of the contact area of the surfaces), the Coulomb approximation is an adequate representation of friction for the analysis of many physical systems.
When the surfaces are conjoined, Coulomb friction becomes a very poor approximation (for example, adhesive tape resists sliding even when there is no normal force, or a negative normal force). In this case, the frictional force may depend strongly on the area of contact. Some drag racing tires are adhesive for this reason. However, despite the complexity of the fundamental physics behind friction, the relationships are accurate enough to be useful in many applications.
“Negative” coefficient of friction
As of 2012, a single study has demonstrated the potential for an effectively negative coefficient of friction in the low-load regime, meaning that a decrease in normal force leads to an increase in friction. This contradicts everyday experience in which an increase in normal force leads to an increase in friction.[49] This was reported in the journal Nature in October 2012 and involved the friction encountered by an atomic force microscope stylus when dragged across a graphene sheet in the presence of graphene-adsorbed oxygen.[49]
Numerical simulation of the Coulomb model
Despite being a simplified model of friction, the Coulomb model is useful in many numerical simulation applications such as multibody systems and granular material. Even its most simple expression encapsulates the fundamental effects of sticking and sliding which are required in many applied cases, although specific algorithms have to be designed in order to efficiently numerically integrate mechanical systems with Coulomb friction and bilateral or unilateral contact.[50][51][52][53][54] Some quite nonlinear effects, such as the so-called Painlevé paradoxes, may be encountered with Coulomb friction.[55]
Dry friction and instabilities
Dry friction can induce several types of instabilities in mechanical systems which display a stable behaviour in the absence of friction.[56]
These instabilities may be caused by the decrease of the friction force with an increasing velocity of sliding, by material expansion due to heat generation during friction (the thermo-elastic instabilities), or by pure dynamic effects of sliding of two elastic materials (the Adams–Martins instabilities). The latter were originally discovered in 1995 by George G. Adams and João Arménio Correia Martins for smooth surfaces[57][58] and were later found in periodic rough surfaces.[59] In particular, friction-related dynamical instabilities are thought to be responsible for brake squeal and the ‘song’ of a glass harp,[60][61] phenomena which involve stick and slip, modelled as a drop of friction coefficient with velocity.[62]
A practically important case is the self-oscillation of the strings of bowed instruments such as the violin, cello, hurdy-gurdy, erhu, etc.
A connection between dry friction and flutter instability in a simple mechanical system has been discovered,[63] watch the movie Archived 2015-01-10 at the Wayback Machine for more details.
Frictional instabilities can lead to the formation of new self-organized patterns (or “secondary structures”) at the sliding interface, such as in-situ formed tribofilms which are utilized for the reduction of friction and wear in so-called self-lubricating materials.[64]
Fluid friction
Fluid friction occurs between fluid layers that are moving relative to each other. This internal resistance to flow is named viscosity. In everyday terms, the viscosity of a fluid is described as its “thickness”. Thus, water is “thin”, having a lower viscosity, while honey is “thick”, having a higher viscosity. The less viscous the fluid, the greater its ease of deformation or movement.
All real fluids (except superfluids) offer some resistance to shearing and therefore are viscous. For teaching and explanatory purposes it is helpful to use the concept of an inviscid fluid or an ideal fluid which offers no resistance to shearing and so is not viscous.
Lubricated friction
Lubricated friction is a case of fluid friction where a fluid separates two solid surfaces. Lubrication is a technique employed to reduce wear of one or both surfaces in close proximity moving relative to each another by interposing a substance called a lubricant between the surfaces.
In most cases the applied load is carried by pressure generated within the fluid due to the frictional viscous resistance to motion of the lubricating fluid between the surfaces. Adequate lubrication allows smooth continuous operation of equipment, with only mild wear, and without excessive stresses or seizures at bearings. When lubrication breaks down, metal or other components can rub destructively over each other, causing heat and possibly damage or failure.
Skin friction
Skin friction arises from the interaction between the fluid and the skin of the body, and is directly related to the area of the surface of the body that is in contact with the fluid. Skin friction follows the drag equation and rises with the square of the velocity.
Skin friction is caused by viscous drag in the boundary layer around the object. There are two ways to decrease skin friction: the first is to shape the moving body so that smooth flow is possible, like an airfoil. The second method is to decrease the length and cross-section of the moving object as much as is practicable.
Internal friction
Internal friction is the force resisting motion between the elements making up a solid material while it undergoes deformation.
Plastic deformation in solids is an irreversible change in the internal molecular structure of an object. This change may be due to either (or both) an applied force or a change in temperature. The change of an object’s shape is called strain. The force causing it is called stress.
Elastic deformation in solids is reversible change in the internal molecular structure of an object. Stress does not necessarily cause permanent change. As deformation occurs, internal forces oppose the applied force. If the applied stress is not too large these opposing forces may completely resist the applied force, allowing the object to assume a new equilibrium state and to return to its original shape when the force is removed. This is known as elastic deformation or elasticity.
Radiation friction
As a consequence of light pressure, Einstein[65] in 1909 predicted the existence of “radiation friction” which would oppose the movement of matter. He wrote, “radiation will exert pressure on both sides of the plate. The forces of pressure exerted on the two sides are equal if the plate is at rest. However, if it is in motion, more radiation will be reflected on the surface that is ahead during the motion (front surface) than on the back surface. The backward-acting force of pressure exerted on the front surface is thus larger than the force of pressure acting on the back. Hence, as the resultant of the two forces, there remains a force that counteracts the motion of the plate and that increases with the velocity of the plate. We will call this resultant ‘radiation friction’ in brief.”
Other types of friction
Rolling resistance
Rolling resistance is the force that resists the rolling of a wheel or other circular object along a surface caused by deformations in the object or surface. Generally the force of rolling resistance is less than that associated with kinetic friction.[66] Typical values for the coefficient of rolling resistance are 0.001.[67]
One of the most common examples of rolling resistance is the movement of motor vehicle tires on a road, a process which generates heat and sound as by-products.[68]
Braking friction
Any wheel equipped with a brake is capable of generating a large retarding force, usually for the purpose of slowing and stopping a vehicle or piece of rotating machinery. Braking friction differs from rolling friction because the coefficient of friction for rolling friction is small whereas the coefficient of friction for braking friction is designed to be large by choice of materials for brake pads.
Triboelectric effect
Rubbing dissimilar materials against one another can cause a build-up of electrostatic charge, which can be hazardous if flammable gases or vapours are present. When the static build-up discharges, explosions can be caused by ignition of the flammable mixture.
Belt friction
Belt friction is a physical property observed from the forces acting on a belt wrapped around a pulley, when one end is being pulled. The resulting tension, which acts on both ends of the belt, can be modeled by the belt friction equation.
In practice, the theoretical tension acting on the belt or rope calculated by the belt friction equation can be compared to the maximum tension the belt can support. This helps a designer of such a rig to know how many times the belt or rope must be wrapped around the pulley to prevent it from slipping. Mountain climbers and sailing crews demonstrate a standard knowledge of belt friction when accomplishing basic tasks.
Reducing friction
Devices
Devices such as wheels, ball bearings, roller bearings, and air cushion or other types of fluid bearings can change sliding friction into a much smaller type of rolling friction.
Many thermoplastic materials such as nylon, HDPE and PTFE are commonly used in low friction bearings. They are especially useful because the coefficient of friction falls with increasing imposed load.[69] For improved wear resistance, very high molecular weight grades are usually specified for heavy duty or critical bearings.
Lubricants
A common way to reduce friction is by using a lubricant, such as oil, water, or grease, which is placed between the two surfaces, often dramatically lessening the coefficient of friction. The science of friction and lubrication is called tribology. Lubricant technology is when lubricants are mixed with the application of science, especially to industrial or commercial objectives.
Superlubricity, a recently discovered effect, has been observed in graphite: it is the substantial decrease of friction between two sliding objects, approaching zero levels. A very small amount of frictional energy would still be dissipated.
Lubricants to overcome friction need not always be thin, turbulent fluids or powdery solids such as graphite and talc; acoustic lubrication actually uses sound as a lubricant.
Another way to reduce friction between two parts is to superimpose micro-scale vibration to one of the parts. This can be sinusoidal vibration as used in ultrasound-assisted cutting or vibration noise, known as dither.
Energy of friction
According to the law of conservation of energy, no energy is destroyed due to friction, though it may be lost to the system of concern. Energy is transformed from other forms into thermal energy. A sliding hockey puck comes to rest because friction converts its kinetic energy into heat which raises the thermal energy of the puck and the ice surface. Since heat quickly dissipates, many early philosophers, including Aristotle, wrongly concluded that moving objects lose energy without a driving force.
When an object is pushed along a surface along a path C, the energy converted to heat is given by a line integral, in accordance with the definition of work
where
Energy lost to a system as a result of friction is a classic example of thermodynamic irreversibility.
Work of friction
In the reference frame of the interface between two surfaces, static friction does no work, because there is never displacement between the surfaces. In the same reference frame, kinetic friction is always in the direction opposite the motion, and does negative work.[70] However, friction can do positive work in certain frames of reference. One can see this by placing a heavy box on a rug, then pulling on the rug quickly. In this case, the box slides backwards relative to the rug, but moves forward relative to the frame of reference in which the floor is stationary. Thus, the kinetic friction between the box and rug accelerates the box in the same direction that the box moves, doing positive work.[71]
The work done by friction can translate into deformation, wear, and heat that can affect the contact surface properties (even the coefficient of friction between the surfaces). This can be beneficial as in polishing. The work of friction is used to mix and join materials such as in the process of friction welding. Excessive erosion or wear of mating sliding surfaces occurs when work due to frictional forces rise to unacceptable levels. Harder corrosion particles caught between mating surfaces in relative motion (fretting) exacerbates wear of frictional forces. As surfaces are worn by work due to friction, fit and surface finish of an object may degrade until it no longer functions properly.[72] For example, bearing seizure or failure may result from excessive wear due to work of friction.
Applications
Friction is an important factor in many engineering disciplines.
Transportation
- Automobile brakes inherently rely on friction, slowing a vehicle by converting its kinetic energy into heat. Incidentally, dispersing this large amount of heat safely is one technical challenge in designing brake systems. Disk brakes rely on friction between a disc and brake pads that are squeezed transversely against the rotating disc. In drum brakes, brake shoes or pads are pressed outwards against a rotating cylinder (brake drum) to create friction. Since braking discs can be more efficiently cooled than drums, disc brakes have better stopping performance.[73]
- Rail adhesion refers to the grip wheels of a train have on the rails, see Frictional contact mechanics.
- Road slipperiness is an important design and safety factor for automobiles[74]
- Split friction is a particularly dangerous condition arising due to varying friction on either side of a car.
- Road texture affects the interaction of tires and the driving surface.
Measurement
- A tribometer is an instrument that measures friction on a surface.
- A profilograph is a device used to measure pavement surface roughness.
Household usage
- Friction is used to heat and ignite matchsticks (friction between the head of a matchstick and the rubbing surface of the match box).[75]
- Sticky pads are used to prevent object from slipping off smooth surfaces by effectively increasing the friction coefficient between the surface and the object.
See also
- Contact dynamics
- Contact mechanics
- Factor of adhesion
- Friction Acoustics
- Frictionless plane
- Galling
- Non-smooth mechanics
- Normal contact stiffness
- Stick-slip phenomenon
- Transient friction loading
- Triboelectric effect
- Unilateral contact
- Friction torque
References
- ^ a b c Hanaor, D.; Gan, Y.; Einav, I. (2016). “Static friction at fractal interfaces”. Tribology International. 93: 229–238. arXiv:2106.01473. doi:10.1016/j.triboint.2015.09.016. S2CID 51900923.
- ^ “friction”. Merriam-Webster Dictionary.
- ^ a b Beer, Ferdinand P.; Johnston, E. Russel, Jr. (1996). Vector Mechanics for Engineers (Sixth ed.). McGraw-Hill. p. 397. ISBN 978-0-07-297688-5.
- ^ a b Meriam, J. L.; Kraige, L. G. (2002). Engineering Mechanics (fifth ed.). John Wiley & Sons. p. 328. ISBN 978-0-471-60293-4.
- ^ Ruina, Andy; Pratap, Rudra (2002). Introduction to Statics and Dynamics (PDF). Oxford University Press. p. 713.
- ^ Hibbeler, R. C. (2007). Engineering Mechanics (Eleventh ed.). Pearson, Prentice Hall. p. 393. ISBN 978-0-13-127146-3.
- ^ Soutas-Little, Robert W.; Inman, Balint (2008). Engineering Mechanics. Thomson. p. 329. ISBN 978-0-495-29610-2.
- ^ a b Chatterjee, Sudipta (2008). Tribological Properties of Pseudo-elastic Nickel-titanium (Thesis). University of California. pp. 11–12. ISBN 9780549844372 – via ProQuest.
Classical Greek philosophers like Aristotle, Pliny the Elder and Vitruvius wrote about the existence of friction, the effect of lubricants and the advantages of metal bearings around 350 B.C.
- ^ Fishbane, Paul M.; Gasiorowicz, Stephen; Thornton, Stephen T. (1993). Physics for Scientists and Engineers. Vol. I (Extended ed.). Englewood Cliffs, New Jersey: Prentice Hall. p. 135. ISBN 978-0-13-663246-7.
Themistius first stated around 350 B.C. [sic] that kinetic friction is weaker than the maximum value of static friction.
- ^ Hecht, Eugene (2003). Physics: Algebra/Trig (3rd ed.). Cengage Learning. ISBN 9780534377298.
- ^ Sambursky, Samuel (2014). The Physical World of Late Antiquity. Princeton University Press. pp. 65–66. ISBN 9781400858989.
- ^ a b c d e Dowson, Duncan (1997). History of Tribology (2nd ed.). Professional Engineering Publishing. ISBN 978-1-86058-070-3.
- ^ a b c Armstrong-Hélouvry, Brian (1991). Control of machines with friction. USA: Springer. p. 10. ISBN 978-0-7923-9133-3.
- ^ a b van Beek, Anton. “History of Science Friction”. tribology-abc.com. Retrieved 2011-03-24.
- ^ Hutchings, Ian M. (2016). “Leonardo da Vinci’s studies of friction” (PDF). Wear. 360–361: 51–66. doi:10.1016/j.wear.2016.04.019. Archived (PDF) from the original on 2016-08-03.
- ^ Hutchings, Ian M. (2016-08-15). “Leonardo da Vinci’s studies of friction”. Wear. 360–361: 51–66. doi:10.1016/j.wear.2016.04.019.
- ^ Kirk, Tom (July 22, 2016). “Study reveals Leonardo da Vinci’s ‘irrelevant’ scribbles mark the spot where he first recorded the laws of friction”. phys.org. Retrieved 2016-07-26.
- ^ a b Popova, Elena; Popov, Valentin L. (2015-06-01). “The research works of Coulomb and Amontons and generalized laws of friction”. Friction. 3 (2): 183–190. doi:10.1007/s40544-015-0074-6.
- ^ Forest de Bélidor, Bernard. “Richtige Grund-Sätze der Friction-Berechnung” (“Correct Basics of Friction Calculation”), 1737, (in German)
- ^ “Leonhard Euler”. Friction Module. Nano World. 2002. Archived from the original on 2011-05-07. Retrieved 2011-03-25.
- ^ Goedecke, Andreas (2014). Transient Effects in Friction: Fractal Asperity Creep. Springer Science and Business Media. p. 3. ISBN 978-3709115060.
- ^ Fleeming Jenkin & James Alfred Ewing (1877) “On Friction between Surfaces moving at Low Speeds”, Philosophical Magazine Series 5, volume 4, pp 308–10; link from Biodiversity Heritage Library
- ^ Air Brake Association (1921). The Principles and Design of Foundation Brake Rigging. Air brake association. p. 5.
- ^ Valentin L. Popov (17 Jan 2014). “Generalized law of friction between elastomers and differently shaped rough bodies”. Sci. Rep. 4: 3750. Bibcode:2014NatSR…4E3750P. doi:10.1038/srep03750. PMC 3894559. PMID 24435002.
- ^ a b c d e f g h i j k l m n o p q r s t u v w x y z aa ab ac ad ae af ag ah ai aj “Friction Factors – Coefficients of Friction”. Archived from the original on 2019-02-01. Retrieved 2015-04-27.
- ^ a b c d “Mechanical Engineering Department: Tribology Introduction”. 2016-03-11.
- ^ Ferreira, Vanderlei; Yoshimura, Humberto Naoyuki; Sinatora, Amilton (2012-08-30). “Ultra-low friction coefficient in alumina–silicon nitride pair lubricated with water”. Wear. 296 (1–2): 656–659. doi:10.1016/j.wear.2012.07.030.
- ^ Tian, Y.; Bastawros, A. F.; Lo, C. C. H.; Constant, A. P.; Russell, A.M.; Cook, B. A. (2003). “Superhard self-lubricating AlMgB[sub 14] films for microelectromechanical devices”. Applied Physics Letters. 83 (14): 2781. Bibcode:2003ApPhL..83.2781T. doi:10.1063/1.1615677.
- ^ Kleiner, Kurt (2008-11-21). “Material slicker than Teflon discovered by accident”. Retrieved 2008-12-25.
- ^ Higdon, C.; Cook, B.; Harringa, J.; Russell, A.; Goldsmith, J.; Qu, J.; Blau, P. (2011). “Friction and wear mechanisms in AlMgB14-TiB2 nanocoatings”. Wear. 271 (9–10): 2111–2115. doi:10.1016/j.wear.2010.11.044.
- ^ a b c d e Coefficient of Friction Archived March 8, 2009, at the Wayback Machine. EngineersHandbook.com
- ^ a b c d e f g h i j k l m n o p q Barrett, Richard T. (1 March 1990). “(NASA-RP-1228) Fastener Design Manual”. NASA Technical Reports Server. NASA Lewis Research Center: 16. hdl:2060/19900009424. Retrieved 3 August 2020.
- ^ a b “Coefficients of Friction of Human Joints”. Retrieved 2015-04-27.
- ^ a b c d e f g h i “The Engineering Toolbox: Friction and Coefficients of Friction”. Retrieved 2008-11-23.
- ^ Dienwiebel, Martin; et al. (2004). “Superlubricity of Graphite” (PDF). Phys. Rev. Lett. 92 (12): 126101. Bibcode:2004PhRvL..92l6101D. doi:10.1103/PhysRevLett.92.126101. PMID 15089689. S2CID 26811802.
- ^ multi-scale origins of static friction 2016
- ^ Greenwood J.A. and JB Williamson (1966). “Contact of nominally flat surfaces”. Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences. 295 (1442).
- ^ Nakano, K.; Popov, V. L. (2020-12-10). “Dynamic stiction without static friction: The role of friction vector rotation”. Physical Review E. 102 (6): 063001. Bibcode:2020PhRvE.102f3001N. doi:10.1103/PhysRevE.102.063001. hdl:10131/00013921. PMID 33466084. S2CID 230599544.
- ^ Bhavikatti, S. S.; K. G. Rajashekarappa (1994). Engineering Mechanics. New Age International. p. 112. ISBN 978-81-224-0617-7. Retrieved 2007-10-21.
- ^ Sheppard, Sheri; Tongue, Benson H.; Anagnos, Thalia (2005). Statics: Analysis and Design of Systems in Equilibrium. Wiley and Sons. p. 618. ISBN 978-0-471-37299-8.
In general, for given contacting surfaces, μk < μs
- ^
Meriam, James L.; Kraige, L. Glenn; Palm, William John (2002). Engineering Mechanics: Statics. Wiley and Sons. p. 330. ISBN 978-0-471-40646-4.Kinetic friction force is usually somewhat less than the maximum static friction force.
- ^ Feynman, Richard P.; Leighton, Robert B.; Sands, Matthew (1964). “The Feynman Lectures on Physics, Vol. I, p. 12–5”. Addison-Wesley. Retrieved 2009-10-16.
- ^
Beatty, William J. “Recurring science misconceptions in K-6 textbooks”. Retrieved 2007-06-08. - ^
- ^ Makkonen, L (2012). “A thermodynamic model of sliding friction”. AIP Advances. 2 (1): 012179. Bibcode:2012AIPA….2a2179M. doi:10.1063/1.3699027.
- ^ Nichols, Edward Leamington; Franklin, William Suddards (1898). The Elements of Physics. Vol. 1. Macmillan. p. 101.
- ^ Ternes, Markus; Lutz, Christopher P.; Hirjibehedin, Cyrus F.; Giessibl, Franz J.; Heinrich, Andreas J. (2008-02-22). “The Force Needed to Move an Atom on a Surface” (PDF). Science. 319 (5866): 1066–1069. Bibcode:2008Sci…319.1066T. doi:10.1126/science.1150288. PMID 18292336. S2CID 451375. Archived (PDF) from the original on 2018-07-20.
- ^ a b Deng, Zhao; et al. (October 14, 2012). “Adhesion-dependent negative friction coefficient on chemically modified graphite at the nanoscale”. Nature. 11 (12): 1032–7. Bibcode:2012NatMa..11.1032D. doi:10.1038/nmat3452. PMID 23064494.
- “At the nanoscale, graphite can turn friction upside down”. R&D Magazine. 2012-10-17. Archived from the original on 2013-07-31.
- ^ Haslinger, J.; Nedlec, J.C. (1983). “Approximation of the Signorini problem with friction, obeying the Coulomb law” (PDF). Mathematical Methods in the Applied Sciences. 5 (1): 422–437. Bibcode:1983MMAS….5..422H. doi:10.1002/mma.1670050127. hdl:10338.dmlcz/104086.
- ^ Alart, P.; Curnier, A. (1991). “A mixed formulation for frictional contact problems prone to Newton like solution method”. Computer Methods in Applied Mechanics and Engineering. 92 (3): 353–375. Bibcode:1991CMAME..92..353A. doi:10.1016/0045-7825(91)90022-X.
- ^ Acary, V.; Cadoux, F.; Lemaréchal, C.; Malick, J. (2011). “A formulation of the linear discrete Coulomb friction problem via convex optimization”. Journal of Applied Mathematics and Mechanics. 91 (2): 155–175. Bibcode:2011ZaMM…91..155A. doi:10.1002/zamm.201000073. S2CID 17280625.
- ^ De Saxcé, G.; Feng, Z.-Q. (1998). “The bipotential method: A constructive approach to design the complete contact law with friction and improved numerical algorithms”. Mathematical and Computer Modelling. 28 (4): 225–245. doi:10.1016/S0895-7177(98)00119-8.
- ^ Simo, J.C.; Laursen, T.A. (1992). “An augmented lagrangian treatment of contact problems involving friction”. Computers and Structures. 42 (2): 97–116. doi:10.1016/0045-7949(92)90540-G.
- ^ Acary, V.; Brogliato, B. (2008). Numerical Methods for Nonsmooth Dynamical Systems. Applications in Mechanics and Electronics. Vol. 35. Springer Verlag Heidelberg.
- ^ Bigoni, D. (2012-07-30). Nonlinear Solid Mechanics: Bifurcation Theory and Material Instability. Cambridge University Press, 2012. ISBN 9781107025417.
- ^ Adams, G. G. (1995). “Self-excited oscillations of two elastic half-spaces sliding with a constant coefficient of friction”. Journal of Applied Mechanics. 62 (4): 867–872. Bibcode:1995JAM….62..867A. doi:10.1115/1.2896013.
- ^ Martins, J.A., Faria, L.O. & Guimarães, J. (1995). “Dynamic surface solutions in linear elasticity and viscoelasticity with frictional boundary conditions”. Journal of Vibration and Acoustics. 117 (4): 445–451. doi:10.1115/1.2874477.
- ^ M, Nosonovsky; G., Adams G. (2004). “Vibration and stability of frictional sliding of two elastic bodies with a wavy contact interface”. Journal of Applied Mechanics. 71 (2): 154–161. Bibcode:2004JAM….71..154N. doi:10.1115/1.1653684.
- ^ J., Flint; J., Hultén (2002). “Lining-deformation-induced modal coupling as squeal generator in a distributed parameter disk brake model”. Journal of Sound and Vibration. 254 (1): 1–21. Bibcode:2002JSV…254….1F. doi:10.1006/jsvi.2001.4052.
- ^ M., Kröger; M., Neubauer; K., Popp (2008). “Experimental investigation on the avoidance of self-excited vibrations”. Phil. Trans. R. Soc. A. 366 (1866): 785–810. Bibcode:2008RSPTA.366..785K. doi:10.1098/rsta.2007.2127. PMID 17947204. S2CID 16395796.
- ^ R., Rice, J.; L., Ruina, A. (1983). “Stability of Steady Frictional Slipping” (PDF). Journal of Applied Mechanics. 50 (2): 343–349. Bibcode:1983JAM….50..343R. CiteSeerX 10.1.1.161.5207. doi:10.1115/1.3167042. Archived (PDF) from the original on 2010-06-22.
- ^ Bigoni, D.; Noselli, G. (2011). “Experimental evidence of flutter and divergence instabilities induced by dry friction”. Journal of the Mechanics and Physics of Solids. 59 (10): 2208–2226. Bibcode:2011JMPSo..59.2208B. CiteSeerX 10.1.1.700.5291. doi:10.1016/j.jmps.2011.05.007. Archived from the original on 2020-08-18. Retrieved 2011-11-30.
- ^ Nosonovsky, Michael (2013). Friction-Induced Vibrations and Self-Organization: Mechanics and Non-Equilibrium Thermodynamics of Sliding Contact. CRC Press. p. 333. ISBN 978-1466504011.
- ^ Einstein, A. (1909). On the development of our views concerning the nature and constitution of radiation. Translated in: The Collected Papers of Albert Einstein, vol. 2 (Princeton University Press, Princeton, 1989). Princeton, NJ: Princeton University Press. p. 391.
- ^ Silliman, Benjamin (1871) Principles of Physics, Or Natural Philosophy, Ivison, Blakeman, Taylor & company publishers
- ^ Butt, Hans-Jürgen; Graf, Karlheinz and Kappl, Michael (2006) Physics and Chemistry of Interfaces, Wiley, ISBN 3-527-40413-9
- ^ Hogan, C. Michael (1973). “Analysis of highway noise”. Water, Air, & Soil Pollution. 2 (3): 387–392. Bibcode:1973WASP….2..387H. doi:10.1007/BF00159677. S2CID 109914430.
- ^ Valentin L. Popov; Lars Voll; Stephan Kusche; Qiang Li; Svetlana V. Rozhkova (2018). “Generalized master curve procedure for elastomer friction taking into account dependencies on velocity, temperature and normal force”. Tribology International. 120: 376–380. arXiv:1604.03407. doi:10.1016/j.triboint.2017.12.047. S2CID 119288819.
- ^ Den Hartog, J. P. (1961). Mechanics. Courier Dover Publications. p. 142. ISBN 978-0-486-60754-2.
- ^ Leonard, William J (2000). Minds-on Physics. Kendall/Hunt. p. 603. ISBN 978-0-7872-3932-9.
- ^ Bayer, Raymond George (2004). Mechanical wear. CRC Press. pp. 1, 2. ISBN 978-0-8247-4620-9. Retrieved 2008-07-07.
- ^ “How Do Car Brakes Work?”. Wonderopolis. Retrieved November 4, 2018.
- ^ Iskander, R; Stevens, A. “Effectiveness of the Application of High Friction Surfacing-Crash-Reduction.pdf” (PDF). Archived (PDF) from the original on 2017-09-03. Retrieved 2017-09-03.
- ^ “How Does Lighting A Match Work?”. curiosity.com. Curiosity. November 11, 2015. Archived from the original on November 5, 2018. Retrieved November 4, 2018.
External links
- “Friction” . Encyclopædia Britannica. Vol. 11 (11th ed.). 1911.
- Coefficients of Friction – tables of coefficients, plus many links
- Measurement of friction power
- Physclips: Mechanics with animations and video clips from the University of New South Wales
- Values for Coefficient of Friction – CRC Handbook of Chemistry and Physics
- Characteristic Phenomena in Conveyor Chain
- Atomic-scale Friction Research and Education Synergy Hub (AFRESH) an Engineering Virtual Organization for the atomic-scale friction community to share, archive, link, and discuss data, knowledge and tools related to atomic-scale friction.
- Coefficients of friction of various material pairs in atmosphere and vacuum.




