Как найти минимальную энергию атома

Водородоподобный
атом — атом,
содержащий в электронной оболочке один
и только один электрон.Таким атомом,
кроме водорода,
и его изотопов,может быть любой ион,
если число потерянных им электронов
равно заряду
атома.
Поэтому, поскольку у такого иона остаётся
только один электрон,
его и называют водородоподобным атомом.(Электронов
столько же ,сколько и у водорода, но
заряд другой)

Вводя
в качестве универсальной константы
теории боровский радиус

как
радиус первой стационарной орбиты
электрона в атоме водорода, запишем
формулу в
виде

Важно
отметить, что оценка размера атома
водорода (),
полученная, совпадает с соответствующей
оценкой из газокинетической теории.

Оценим
энергию основного состояния, в котором
энергия минимальна.

.

Подставим
найденное значение r в
выражение для полной энергии:

и
получим

.

Для
водорода (Z =
1) r =
0.53·10^-10м, E =
13.6 эВ. Это радиус первой боровской орбиты
и |E|
– точное значение энергии связи
электрона. Решение довольно грубое, но
дает правильную зависимость энергии
от m, Z, e и h.

30.Оценка минимальной энергии частицы в одномерной бесконечно глубокой потенциальной яме.

Потенциальная
яма –
область пространства, где присутствует
локальный минимум потенциальной
энергии частицы.

Бесконечная
глубина ямы означает, что потенциальная
энергия частицы внутри ямы равна нулю,
а вне ямы — бесконечности.

Для
бесконечной одномерной потенциальной
ямы имеем следующее:Энергия частицы
принимает определенные дискретные
значения. Обычно говорят, что частица
находится в определенных энергетических
состояниях. где
n = 1, 2, 3…

E=T+U
E=p²/2m
∆x≤L
∆p≥ħ⁄2L

Емин~p²/2m~ħ²/mL²
Емин≥π²/mL²

Соседние файлы в папке Семестр 01

• #
• #
• #
• #
• #

Ionization energy can be defined as the minimum amount of energy required to remove the valence electron i.e. the most loosely bound electron from an isolated gaseous atom or ion. It is important to note the term isolated gaseous atom as if the atom is not isolated, then the energy required may be more due to the interatomic forces. 

Let’s learn about Ionization Energy in detail in this article.

What is Ionization Energy?

Ionization Energy is defined as the difficulty in removing the electron from an atom or ion.

X + Δ ⟶  X⁺ + e⁻

Ionization is an endothermic process as the energy needs to be supplied in order to remove the electron. The ionization energy increases as the removal of electrons takes place due to the fact that the atom now gains a +ve charge and the electrons are held more tightly.  Thus it is difficult to remove an electron from a cation.

Ionization energy or ionization potential is measured in electronvolts (eV) or KJ/mol. eV is a preferred unit in physics while kJ/mol is a preferred unit in chemistry as chemistry generally deals with 1 mole of an atom.

Factors Affecting Ionization Energy

Factors that affect the Ionization Energy of any atom and molecule are:

• If an atom or ion is positively charged then the electrons are more tightly held by the nucleus, so the ionization energy is high.
• If the number of shells is less i.e. the electrons are near to the nucleus then the IE is high and vice versa.
• It is difficult to remove the inner electrons as compared to the outer electrons due to this reason.
• The IE is less when there is more than 1 electron in a shell due to the interelectronic repulsions.
• An atom with lower IE acts as a good reducing agent while those with high IE act as a good oxidizing agent.

Ionization Energy using Bohr’s Atomic Model

According to Bohr’s model of the atom, electrons can exist only in fixed paths called orbits. The electrons revolve around the nucleus in these fixed orbits due to the attraction of the nucleus. Each orbit has its own fixed energy. The electron in a particular orbit is said to possess the energy of the same orbit. If an electron gains energy equal to the energy difference between two orbits then it can jump to the higher orbit whose energy it possesses. This is called the excitation of the electron. If an electron gains enough energy, then it can overcome the attractive force of the nucleus and finally come out of the atom. Such an atom is said to be ionized.

The image given below shows the Ionization energy of the electron.

Ionization Energy Formula

Thus the ionization energy is the energy difference between the energy of the electron in the initial orbit and the energy of the electron outside the atom (infinite orbit in this case).

According to Bohr, the energy of an electron in the nth orbit is given by:

where,
m is mass of one electron,
e is charge on one electron,
h is Planck’s constant,
Z is atomic number,
n is the orbit from which electron is removed

This formula can be rewritten as,

E_n ​=−R × n² / Z²​

E_n = −13.6 × n² / Z² eV / atom

This is the energy of the atom in the nth orbit. Now we said that the ionization energy is the energy difference between the energy of the electron in the initial orbit and the energy of the electron in the infinite orbit. Thus we can consider the initial orbit as n₁​ = n and the final orbit as n₂ = ∞.

IE = E_n2​​ − E_n1​​ = ΔE

As 

This formula is known as the ionization energy formula. Note that the IE is the same as the energy of the electron in the nth orbit but positive in sign. This is due to the fact that the energy is supplied to remove the electron and thus it has a positive sign.

How to Determine the Ionization Energy of an Element?

The Ionization Energy of an Element of an element is calculated using the steps given below,

Step 1: First write the atomic number of the atom for which the Ionization energy has to be found.

Step 2: Determine ‘n’ the value of the orbit for which ionization energy is to be found.

Step 3: Use the formula for finding the Ionization Energy. E_n = −13.6 × n² / Z² eV / atom

Step 4: Substitute all the values in the above formula and simplify to get Ionization Energy in electron volts.

Successive Ionization Energies

An atom can have any number of ionization energies depending on the number of electrons it has.

First Ionization Energy

Energy required to remove the first outermost electron from a neutral atom is known as the first IE. The first IE is equal to the energy of the electron in that particular orbit.

Second Ionization Energy

The energy required to remove the second electron from an atom with +1 charge is called second IE and so on. The second Ionization energy is always higher than the first IE as it is difficult to remove the electron from a positively charged ion.

X + ΔH₁ ⟶ X⁺ + e⁻

where ΔH₁ = First IE

X⁺ + ΔH₂ ⟶ X⁺² + e⁻

where ΔH_2​ = Second IE

The IE keeps on increasing as the charge on the atom increases. Thus the third IE is higher than the second and the second IE is higher than the first IE.

ΔH₁ < ΔH₂ < ΔH₃ …… < ΔH_n

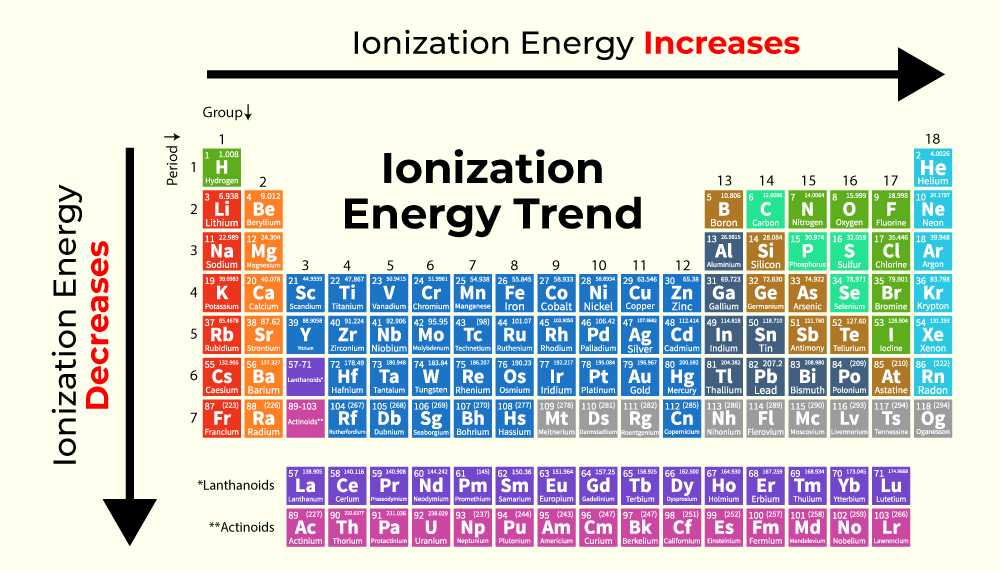
Ionization Energy Trends in Periodic Table

Ionization Energy follows a specific trend while moving from Left to Right and Top to Bottom in the periodic table.

The image given below shows the trend of variation of Ionization Energy while moving left to right and top to bottom in the periodic table. 

Left to Right in a Period

As we move from left to right in a period in the periodic table the atomic radius decreases. It is due to the reason that the elements start gaining near noble gas configuration as we move across the period. Due to this interelectronic repulsion is reduced and the atomic radius decreases. Hence the electrons are more tightly held by the nucleus. Hence a higher amount of energy is required to remove the electrons. Thus IE increases as we move across a period.

Top to Bottom in a Group

As we move from top to bottom in a group, new shells are added up and the electrons start to occupy higher orbitals and shells. Thus the atomic radius also increases. The electrons are now far away from the nucleus. So lesser amount of energy is required to remove the electron. Thus IE decreases as moves down a group.

Read More,

• Nuclear Binding Energy
• Molecules and Compounds
• Gram Atomic and Gram Molecular Mass

Solved Example on Ionization Energy

Example 1: Calculate the ionization energy of the Hydrogen atom if the electron is in the second orbit.

Solution:

For hydrogen given, Z = 1, n = 2

Thus, 

ΔE = 13.6 × n² / Z²

     = 13.6 × 1/4

     = 3.4 eV

Example 2: Find the IE required to remove the outermost electron from the Sodium atom.

Solution:

For Sodium, Z=11 and n=3 for outermost electron

Thus, 

ΔE = 13.6 × n² / Z²

     = 13.6 × 121/9

     = 182.844 eV

Example 3: Calculate the wavelength of radiation required to ionize the Na atom if it is known that the ionization energy of Na is 496 kJ/mol.

Solution:

Given, 

ΔE = 496 kJ/mol, 

λ = ?

We know that E = hc / λ

496 = (6.6 × 10⁻³⁴ × 3 × 10⁸) / λ

λ = 3.99 ×10⁻²⁸ ms⁻¹

Example 4: The first, second, and third ionization energies of Aluminium are 578, 1817, and 2745 kJ/mol. Calculate the ionization energy required to convert Al ion to Al³⁺.

Given,

ΔH₁ = 578, ΔH₂ = 1817, ΔH₃ = 2745

Al + ΔE ⟶  Al³⁺

ΔE = ΔH₁ + ΔH₂ + ΔH₃

ΔE = 578+1817+2745

     = 5140 kJ/mol

FAQs on Ionization Energy

Q1: What is Ionization Energy?

Answer:

Ionization energy can be defined as the minimum amount of energy required to remove the valence electron i.e. the most loosely bound electron from an isolated gaseous atom or ion.

Q2: What factors govern Ionization Energy?

Answer:

Factors governing ionization energy are,

• If an atom or ion is positively charged then the electrons are more tightly held by the nucleus, so the ionization energy is high.
• If the number of shells are less i.e. the electrons are near to the nucleus then the IE is high and vice versa.
• The IE is less when there are more than 1 electrons in a shell due to the inter electronic repulsions.

Q3: Why does the ionization energy increase with the increase in charge?

Answer:

As the charge on the atom increases, the inter electronic repulsions become less. Thus the electrons are more closer to the nucleus and held more tightly. Thus it becomes difficult to remove the electron and hence the ionization energy increases with the increase in charge of the atom.

Q4: Which has the highest ionization energy between nitrogen and oxygen?

Answer:

Nitrogen has the highest ionization energy between nitrogen and oxygen as nitrogen has half-filled electronic configuration so it requires more energy to extract electrons from the Nitrogen’s orbit.

Q5: Which of the following element has the highest first ionization energy He⁺, Li⁺², Be⁺³

Answer: 

As we can see that all these ions have one electron each. So we will compare their nuclear charge because higher the nuclear charge, higher is the IE because electrons are more tightly held by the nucleus. 

Thus, Be⁺³ has ionization Energy.

Популярное на сайте:

Как быстро выучить стихотворение наизусть? Запоминание стихов является стандартным заданием во многих школах. 

Как научится читать по диагонали? Скорость чтения зависит от скорости восприятия каждого отдельного слова в тексте. 

Как быстро и эффективно исправить почерк?  Люди часто предполагают, что каллиграфия и почерк являются синонимами, но это не так.

Как научится говорить грамотно и правильно? Общение на хорошем, уверенном и естественном русском языке является достижимой целью. 

2018-01-11   

Найти минимальную кинетическую энергию а-частиц, способных сблизиться с -первоначально покоившимся ядром азота до расстояния $R = 5 cdot 10^{-13} см$. Ответ выразить в электрон-вольтах. Массовые числа атомов гелия и азота: $A_{He} = 4, A_{N} = 14$.

Решение:

Задача решается с помощью законов сохранения импульса и энергии. В начальном состоянии, когда $alpha$-частица находится на большом удалении от ядра азота, импульс системы равен импульсу налетающей частицы $m_{ alpha} v_{ alpha}$. В момент наибольшего сближения ядра и частицы они движутся с одинаковой скоростью $v$. Закон сохранения импульса запишется в виде

$m_{ alpha}v_{ alpha} = (m_{ alpha} + m_{N})v$. (1)

Начальная механическая энергия системы равна кинетической энергии $alpha$-частицы, а конечная — кинетической энергии двигающихся со скоростью $v$ ядра азота и $alpha$ – частицы и энергии их электростатического взаимодействия:

$frac{m_{ alpha}v_{ alpha}^{2}}{2} = frac{(m_{ alpha} + m_{N})v^{2}}{2} + frac{q_{ alpha}q_{N}}{4 pi epsilon_{0}R}$. (2)

Подставляя сюда $q_{ alpha} = Z_{ alpha} e, q_{N} = Z_{N}e$, где $Z_{ alpha} = 2, Z_{N} = 7$ — атомные номера частиц, из соотношений (1) и (2) найдем

$v_{ alpha}^{2} = frac{2Z_{ alpha}Z_{N}e^{2}}{4 pi epsilon_{0}R} frac{m_{ alpha} + m_{N}}{m_{ alpha}m_{N}}$.

Энергия $alpha$-частицы

$W_{ alpha} = frac{m_{ alpha}v_{ alpha}^{2}}{2} = frac{Z_{ alpha}Z_{N}e^{2}}{4 pi epsilon_{0}R} frac{m_{ alpha} + m_{N}}{m_{N}}$.

Введем массовые числа атомов гелия и азота $A_{He} = 4, A_{N} = 14$ и выразим через них массы частиц, учитывая, что $alpha$-частица является ядром атома гелия:

$m_{ alpha} = m_{He} = A_{He}m_{0}; m_{N} = A_{N}m_{0}$,

где $m_{0}$ — атомная единица массы. В результате получим

$W_{ alpha} = frac{Z_{ alpha }Z_{N}e^{2}}{4 pi epsilon_{0}R} frac{A_{He} + A_{N}}{A_{N}} = 8,3 cdot 10^{-13} Дж = 5,2 МэВ$.