Как найти миноры матрицы калькулятор

Как найти миноры матрицы калькулятор

Этот онлайн калькулятор позволит вам очень просто найти произведение двух матриц.
Для того чтобы найти произведение матриц онлайн (умножить матрицы онлайн).

Заполните матрицу:

– порядок матрицы

r = – строка
c = – столбец

Количество знаков после разделителя дроби в числах:

Mi,j
 = =

Скачать калькулятор

Рейтинг: 2.6 (Голосов 269)

×

Пожалуйста напишите с чем связна такая низкая оценка:

×

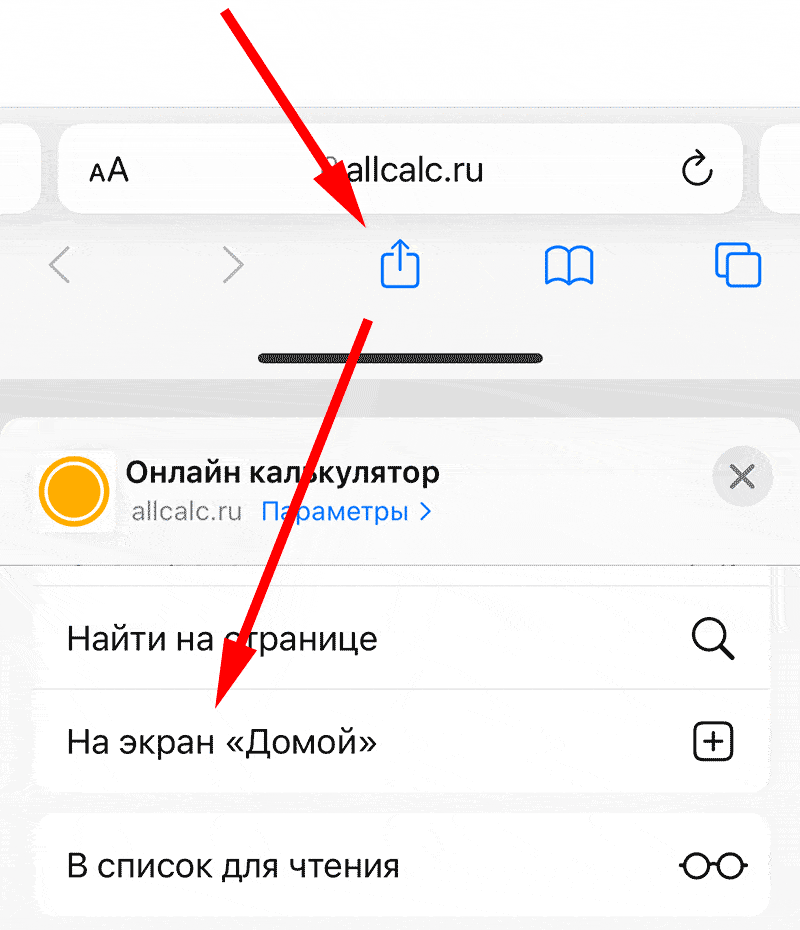
Для установки калькулятора на iPhone – просто добавьте страницу
«На главный экран»

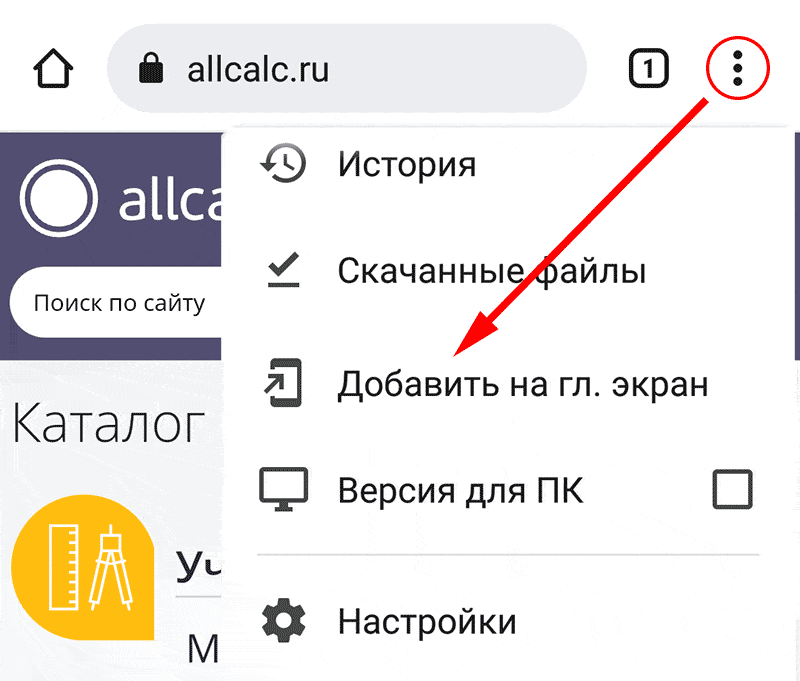
Для установки калькулятора на Android – просто добавьте страницу
«На главный экран»

Сообщить об ошибке

Смотрите также

Операции с матрицами Действия с векторами Решение СЛУ Ранг матрицы Решение матриц
Определитель матрицы Геометрия Математический анализ Аналитическая геометрия Арифметика
Источник

bold{mathrm{Basic}} bold{alphabetagamma} bold{mathrm{ABGamma}} bold{sincos} bold{gedivrightarrow} bold{overline{x}spacemathbb{C}forall} bold{sumspaceintspaceproduct} bold{begin{pmatrix}square&square\square&squareend{pmatrix}} bold{H_{2}O}
square^{2} x^{square} sqrt{square} nthroot[msquare]{square} frac{msquare}{msquare} log_{msquare} pi theta infty int frac{d}{dx}
ge le cdot div x^{circ} (square) |square| (f:circ:g) f(x) ln e^{square}
left(squareright)^{‘} frac{partial}{partial x} int_{msquare}^{msquare} lim sum sin cos tan cot csc sec
alpha beta gamma delta zeta eta theta iota kappa lambda mu
nu xi pi rho sigma tau upsilon phi chi psi omega
A B Gamma Delta E Z H Theta K Lambda M
N Xi Pi P Sigma T Upsilon Phi X Psi Omega
sin cos tan cot sec csc sinh cosh tanh coth sech
arcsin arccos arctan arccot arcsec arccsc arcsinh arccosh arctanh arccoth arcsech
begin{cases}square\squareend{cases} begin{cases}square\square\squareend{cases} = ne div cdot times < > le ge
(square) [square] ▭:longdivision{▭} times twostack{▭}{▭} + twostack{▭}{▭} – twostack{▭}{▭} square! x^{circ} rightarrow lfloorsquarerfloor lceilsquarerceil
overline{square} vec{square} in forall notin exist mathbb{R} mathbb{C} mathbb{N} mathbb{Z} emptyset
vee wedge neg oplus cap cup square^{c} subset subsete superset supersete
int intint intintint int_{square}^{square} int_{square}^{square}int_{square}^{square} int_{square}^{square}int_{square}^{square}int_{square}^{square} sum prod
lim lim _{xto infty } lim _{xto 0+} lim _{xto 0-} frac{d}{dx} frac{d^2}{dx^2} left(squareright)^{‘} left(squareright)^{”} frac{partial}{partial x}
(2times2) (2times3) (3times3) (3times2) (4times2) (4times3) (4times4) (3times4) (2times4) (5times5)
(1times2) (1times3) (1times4) (1times5) (1times6) (2times1) (3times1) (4times1) (5times1) (6times1) (7times1)
mathrm{Радианы} mathrm{Степени} square! ( ) % mathrm{очистить}
arcsin sin sqrt{square} 7 8 9 div
arccos cos ln 4 5 6 times
arctan tan log 1 2 3 –
pi e x^{square} 0 . bold{=} +

Подпишитесь, чтобы подтвердить свой ответ

Подписаться

Войдите, чтобы сохранять заметки

Войти

Номер Строки

Примеры

  • кофакторы:begin{pmatrix}1&-4\4&-7end{pmatrix}

  • мелкие:begin{pmatrix}1&2&1\6&-1&0\-1&-2&-1end{pmatrix}

  • кофакторы:begin{pmatrix}0&9&3\2&0&4\3&7&0end{pmatrix}

  • мелкие:begin{pmatrix}a&1\0&2aend{pmatrix}

  • Показать больше

Описание

Пошаговый поиск миноров и кофакторов матрицы

matrix-minors-cofactors-calculator

мелкие begin{pmatrix}1&2&1\6&-1&0\-1&-2&-1end{pmatrix}

ru

Блог-сообщения, имеющие отношение к Symbolab

  • The Matrix… Symbolab Version

    Matrix, the one with numbers, arranged with rows and columns, is extremely useful in most scientific fields. There…

    Read More

    • Введите Задачу

    Сохранить в блокнот!

    Войти

    Источник

    Минор матрицы

    Операции с матрицами

    Минором Δij элемента аij квадратной матрицы Аn×n (матрицы n-го порядка) будет определитель матрицы А (n-1) -го порядка, который находим способом вычеркивания i-й строки и j-го столбца из матрицы А (вычеркиваем строку и столбец, на пересечении которых расположен элемент аij).

    Рассмотрим квадратную матрицу А

    Пусть Минор матрицы

    Для любого элемента аij матрицы можно найти дополнительный минор Δij посредством вычеркивания строки i и столбца j.

    Алгебраическим дополнением Аij элемента аij матрицы n-го порядка является его минор, знак которого состоит из номеров строки и столбца:

    Aij = (-1)i+j х Δij,

    Mij — обозначение минора элемента аij.

    Представим матрицу Минор матрицы
    Тогда каждому ее элементу будет соответствовать алгебраическое дополнение
    (-1)i+j × Δij

    Алгебраическое дополнение и минор будут одинаковыми при четной сумме номеров строки и столбца. Если сумма номеров является нечетным числом, то они будут различаться знаком.

    В прямоугольных матрицах количество строк не равно количеству столбцов. В этом случае минором k-го порядка матрицы A, состоящей из m строк и n столбцов, является определитель с элементами, находящимися на пересечении k строк и k столбцов матрицы. При этом, k ≤ m и k ≤ n

    С помощью онлайн калькулятора вы сможете быстро рассчитать значение минора.

     — порядок матрицы

    r = – строка
    c = – столбец

    Источник

    dCode

    Search for a tool

    Minors of a Matrix

    Tool for calculating the minors of a matrix, i.e. the values of the determinants of its square sub-matrices (removing one row and one column of the starting matrix).

    Results

    Minors of a Matrix –

    Tag(s) : Matrix

    Share

    Share

    dCode and more

    dCode is free and its tools are a valuable help in games, maths, geocaching, puzzles and problems to solve every day!
    A suggestion ? a feedback ? a bug ? an idea ? Write to dCode!

    1. Mathematics
    2. Matrix
    3. Minors of a Matrix

    Minors of NxN Matrix Calculator

    Answers to Questions (FAQ)

    What is a matrix minor? (Definition)

    The minors of a square matrix $ M = m_{i, j} $ of size $ n $ are the determinants of the square sub-matrices obtained by removing the row $ i $ and the column $ j $ from $ M $.

    Sometimes minors are defined by removing opposing rows and columns (ie. row $ n-i $ and column $ n-j $).

    How to calculate a matrix minors?

    For a square matrix of order 2, finding the minors is calculating the matrix of cofactors without the coefficients.

    For larger matrices like 3×3, calculate the determinants of each sub-matrix.

    Example: $$ M = begin{bmatrix} a & b & c \ d & e & f \ g & h & i end{bmatrix} $$

    The determinant of the sub-matrix obtained by removing the first row and the first column is: $ ei-fh $$, do the same for all combinations of rows and columns.

    What is the difference between a minor and a cofactor?

    For a square matrix, the minor is identical to the cofactor except for the sign (indeed, the cofactors can have a – sign depending on their position in the matrix). Minors do not take this minus sign.

    Source code

    dCode retains ownership of the “Minors of a Matrix” source code. Except explicit open source licence (indicated Creative Commons / free), the “Minors of a Matrix” algorithm, the applet or snippet (converter, solver, encryption / decryption, encoding / decoding, ciphering / deciphering, breaker, translator), or the “Minors of a Matrix” functions (calculate, convert, solve, decrypt / encrypt, decipher / cipher, decode / encode, translate) written in any informatic language (Python, Java, PHP, C#, Javascript, Matlab, etc.) and all data download, script, or API access for “Minors of a Matrix” are not public, same for offline use on PC, mobile, tablet, iPhone or Android app!
    Reminder : dCode is free to use.

    Cite dCode

    The copy-paste of the page “Minors of a Matrix” or any of its results, is allowed (even for commercial purposes) as long as you cite dCode!
    Exporting results as a .csv or .txt file is free by clicking on the export icon
    Cite as source (bibliography):
    Minors of a Matrix on dCode.fr [online website], retrieved on 2023-05-20, https://www.dcode.fr/matrix-minors

    French (Français)

    Summary

    https://www.dcode.fr/matrix-minors

    © 2023 dCode — The ultimate ‘toolkit’ to solve every games / riddles / geocaching / CTF.

    ▲

     

    Источник

    Этот онлайн калькулятор позволит вам очень просто найти произведение двух матриц.
    Для того чтобы найти произведение матриц онлайн (умножить матрицы онлайн).

    Заполните матрицу:

    – порядок матрицы

    r = – строка
    c = – столбец

    Количество знаков после разделителя дроби в числах:

    Вам помог этот калькулятор?

    Предложения и пожелания пишите на [email protected]

    Поделитесь этим калькулятором на форуме или в сети!

    Это помогает делать новые калькуляторы.

    НЕТ

    Источник

    Добавить комментарий