Как найти объем шести угольной призмы

Как найти объем шести угольной призмы

Объем правильной шестиугольной призмы

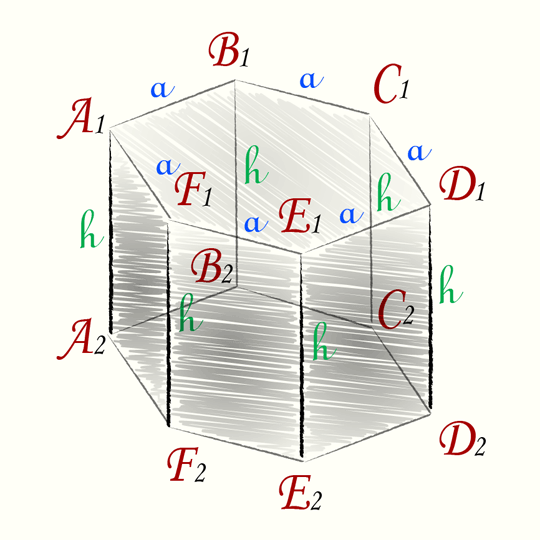
У правильной шестиугольной призмы в основании лежит правильный шестиугольник.

Объем правильной шестиугольной призмы

Объем правильной шестиугольной призмы

Объем правильной шестиугольной призмы равен произведению площади правильного шестиугольника лежащего в основании на высоту призмы.

[ V = frac{3sqrt{3}}{2}a^{2} h ]

Вычислить, найти объем правильной шестиугольной призмы

a (сторона правильного шестиугольника) 
h (высота призмы) 

Вычислить

нажмите кнопку для расчета

Объем правильной шестиугольной призмы

 стр. 362
Источник

При необходимости вычислить объем правильной шестиугольной призмы достаточно знать сторону ее грани
(ребро) a и высоту h. Формула вычисления объема следующая (объем любой призмы равен площади ее
основания, умноженной на высоту, в данном случае берется площадь основания правильного
шестиугольника):

V = (3 * a² / 2) * h * √3

где a — сторона его граней (ребро), h — высота.

Цифр после
запятой:

Результат в:

Пример. Вычислим объем шестигранного простого карандаша типа 7 длиной 177 мм (это
высота призмы h) со стороной грани a = 4,5 мм. V = (3 * 4,5² / 2) * 177 * √3 =9312 (мм³) = 9,312 см³.

Призма – это многогранник, две грани которого являются равными многоугольниками, лежащими в
параллельных плоскостях (эти грани называются основаниями), а остальные грани (называемые боковыми
гранями) являются параллелограммами, у которых стороны общие со сторонами многоугольников. Если у
призмы боковые ребра перпендикулярны плоскости основания, она называется прямой призмой. Все боковые
ребра призмы параллельны и равны по длине. Длина бокового ребра прямой призмы – это ее высота. Если
при этом у прямой призмы основания представляют собой правильные многоугольники, призма называется
правильной. У правильной призмы боковые грани представляют собой равные прямоугольники.

У правильной шестиугольной призмы (другое название – правильная шестигранная призма) основания –
правильные шестиугольники. Примерами правильной шестиугольной призмы являются новый незаточенный
шестигранный карандаш, шестигранная головка болта, башни некоторых средневековых замков, смотревшие
в поле 3 или 4 сторонами.

Источник

A hexagonal prism is a three-dimensional geometric structure with two hexagonal bases connected by six rectangular faces. It is a polyhedron with eight faces, twelve vertices, and eighteen edges. It is also known as an octahedron as it has eight faces; two of the eight faces are hexagons, which are the bases of the prism, and the other six faces are rectangles, which are the lateral (or) side faces of the prism. The top and bottom faces of the hexagonal prism are in the shape of a hexagon and are congruent with each other. 

There are two kinds of hexagonal prisms, namely, a regular hexagonal prism and an irregular hexagonal prism.

  • A regular hexagonal prism is a prism that has two hexagonal bases whose all sides are of the same length. In a regular hexagonal prism, the angles also measure the same.
  • An irregular hexagonal prism is a prism that has two irregular hexagonal bases. All the sides of the base do not have the same length, and the measures of each angle are different.

Surface Area of a Hexagonal Prism

The total area that is covered by the surfaces of a hexagonal prism is referred to as its surface area. The surface area of a prism is measured in terms of square units such as sq. m, sq. cm, sq. in, etc. A hexagonal prism has two types of areas just like other three-dimensional shapes: lateral surface area (LSA) and total surface area (TSA).

Let us consider a hexagonal prism that has an apothem length “a”, a base length “s”, and a height “h”. We know that the general formula to calculate the lateral surface area of a prism is the product of its base and height. So, the lateral surface area of the prism of a hexagonal prism is determined by calculating the product of the perimeter of the base of the hexagonal prism and its height.

The formula to determine the lateral surface area of the hexagonal prism is equal to the sum of the areas of its six rectangular faces. Thus, 

Lateral surface area of hexagonal prism (LSA) = 6sh sq. units.

Where “s” is the length of the base edge, and
“h” is the height of the prism.

The formula to determine the surface area of a hexagonal prism is given as follows:

Total Surface Area, TSA = 2×(Area of hexagonal base) + 6×(Area of rectangular faces) = 6s(a + h).

Total surface area of the hexagonal prism (TSA) = 6s(a + h) sq. units.

Where “a” is the apothem length,
“s” is the length of the base edge, and
“h” is the height of the prism.

The formula to determine the surface area of a hexagonal prism in the case of a regular hexagonal prism, TSA = 6sh + 3√3s2.

Total surface area of the hexagonal prism (TSA) = 6sh + 3√3s2 sq. units.

Where “s” is the length of the base edge, and
“h” is the height of the prism.

Volume of a Hexagonal Prism

The volume of a hexagonal prism is the amount of space enclosed by it in three-dimensional space. It is also referred to as the amount of substance that it can hold, which is the capacity of a hexagonal prism. The formula for the volume of a hexagonal prism is equivalent to the product of its base area and height, which is measured in terms of cubic units such as cm3, m3, in3, etc.

The formula for finding the volume of a hexagonal prism is given as follows,

Volume of the Hexagonal Prism (V) = Base area × height

The formula for calculating the volume of a hexagonal prism when the length of the edge of the base and height of the prism is known is given as follows.

Volume of the Hexagonal Prism (V) = [(3√3)/2]s2h

Where “s” is the length of the base edge, and
“h” is the height of the prism.

The formula for calculating the volume of a hexagonal prism when the apothem length, length of the edge of the base, and height of the prism are known is given as follows.

Volume of the Hexagonal Prism (V) = 3ash

Where “a” is the apothem length,
“s” is the length of the base edge, and
“h” is the height of the prism.

Solved Example on Volume of a Hexagonal Prism

Example 1: Calculate the volume of a hexagonal prism with a base edge length of 15 cm and a height of 12 cm.

Solution:

Given data, 

Length of the base edge (s) = 15 cm

The height of the prism (h) = 12 cm

We know that,

The volume of a hexagonal prism = [(3√3)/2]s2h

= (3/2) × (1.732) × (15)2 × 12

= 7,014.805 cu. cm

Hence, the volume of the hexagonal prism is 7,014.805 cu. cm.

Example 2: Determine the volume of the hexagonal prism if its height is 10 inches and its base area is given as 60 sq. in.

Solution:

Given data, 

Base area = 60 sq. in

The height of the prism (h) = 10 inches

We know that

The volume of the hexagonal prism (V) = Base area × height

= 60 × 10 = 600 cu. in

Hence, the volume of the hexagonal prism is 600 cu. in.

Example 3: Calculate the volume of a hexagonal prism if its height is 13 cm, the length of each side of the base is 10 cm, and the apothem length is 8 cm.

Solution:

Given data,

Length of the base edge length (s) = 10 cm

Apothem length (a) = 8 cm

The height of the prism (h) = 13 cm

We know that

The volume of the hexagonal prism (V) = 3ash cubic units

= 3 × 8 × 10 × 13 = 3,120 cu. cm

Hence, the volume of the hexagonal prism is 3,120 cu. cm.

Example 4: Find the total surface area of a hexagonal prism if the length of each side of the base is 8 cm and the height is 10 cm.

Solution:

Given data,

Length of the base edge (s) = 8 cm

The height of the prism (h) = 10 cm

We know that,

The total surface area of the hexagonal prism = 6sh + 3√3s2

= 6 × 8 × 10 + 3√3 × (8)2

= 480 + 3√3 × 64

= 480 + 332.554 = 812.554 sq. cm

Thus, the total surface area of the hexagonal prism is 812.554 sq. cm.

Example 5: Determine the lateral surface area of a hexagonal prism with a base edge length of 12 cm and a height of 9 cm.

Solution:

Given data,

Length of the base edge length (s) = 12 cm

The height of the prism (h) = 9 cm

We know that,

The lateral surface area of a hexagonal prism = 6sh sq. units

LSA = 6 × 12 × 9

LSA = 648 sq. cm

Hence, the volume of the hexagonal prism is 648 sq. cm.

FAQs on Hexagonal Prism

Question 1: What is the Volume of a Hexagonal Prism?

Answer:

The volume of a hexagonal prism is the amount of space enclosed by it in three-dimensional space. It is also referred to as the amount of substance that it can hold, which is the capacity of a hexagonal prism. The formula for the volume of a hexagonal prism is equivalent to the product of its base area and height, which is measured in terms of cubic units such as cm3, m3, in3, etc.

The formula for finding the volume of a rectangular prism is given as follows,

The volume of the hexagonal prism (V) = Base area × height

Question 2: How to determine the Volume of a Hexagonal Prism?

Answer:

Follow the steps given below to determine the volume of a hexagonal prism:

Step 1: Calculate the base area of the prism using the appropriate formula.
Step 2: Note down the value of the height of the given hexagonal prism.
Step 3: Substitute the values of the given dimensions in the formula, V = [(3√3)/2]s2h
Step 4: Finally, write the final value in appropriate cubic units.

Question 3: How can we determine the Volume of a Hexagonal Prism when its Base Area and Height are given?

Answer:

We know that the general formula for calculating the volume of any prism is the product of its base area and its height. A hexagonal prism is a prism that has a hexagon as its base. So, we can also use the same formula for determining the volume of a hexagonal prism. As both the base area and the height of the hexagonal prism are given, the formula to determine the volume of a hexagonal prism is given as follows:

Volume of a hexagonal prism = Base Area × height.

Question 4: What will happen to the Volume of the Hexagonal Prism if its Height is reduced to Half?

Answer:

We know that the formula for calculating the volume of the hexagonal prism is V = 3abh. Where “a” is the apothem length, “s” is the length of the base edge, and “h” is the height of the prism. So, the volume of the prism is directly proportional to its height. So, if the height is reduced to half, the new height will become (h/2). So, the volume of the new hexagonal prism will be 3ab (h/2) = (1/2) (3abh) = V/2. Therefore, we can conclude the volume will also be reduced by half.

Question 5: What is meant by the Surface Area of a Hexagonal Prism?

Answer:

The total area that is covered by the surfaces of a hexagonal prism is referred to as its surface area. The surface area of a prism is measured in terms of square units such as sq. m, sq. cm, sq. in, etc. The formula to determine the surface area of a hexagonal prism is given as follows:

Total Surface Area, TSA = 2(Area of hexagon base) + 6(Area of rectangle faces) = 6s(a + h), where the “a” is apothem length of the prism, “s” is the length of the base edge, and “h” is the height of the prism. 

The formula to determine the surface area of a hexagonal prism in the case of a regular hexagonal prism, TSA = 6sh + 3√3s2.

Question 6: How to determine the Lateral Surface Area of a Hexagonal Prism?

Answer:

Follow the steps mentioned below to determine the lateral surface area of the hexagonal prism:

  • Step 1: Note down the values of the apothem length, base length, and height of the given hexagonal prism.
  • Step 2: Substitute the given values in the formula of the lateral surface area of a hexagonal prism, LSA = 6sh. 
  • Step 3: Write the resulting value in square units.

Источник

Текущая версия страницы пока не проверялась опытными участниками и может значительно отличаться от версии, проверенной 18 апреля 2020 года; проверки требует 1 правка.

Шестиугольная призма — призма с шестиугольным основанием. У этого многогранника 8 граней, 18 рёбер и 12 вершин[1].

До заточки многие карандаши имеют форму длинной шестиугольной призмы[2].

Полуправильный (или однородный) многогранник[править | править код]

Если все боковые грани одинаковые, шестиугольная призма является полуправильным многогранником, более обще, однородным многогранником и четвёртой призмой в бесконечном множестве призм, образованных прямоугольными боковыми сторонами и двумя правильными основаниями. Призму можно рассматривать как усечённый[en] шестигранный осоэдр, представленный символом Шлефли t{2,6}. С другой стороны, его можно рассматривать как прямое произведение правильного шестиугольника на отрезок, которое представляется как {6}×{}. Двойственным многогранником шестиугольной призмы является шестиугольная бипирамида[en].

Группой симметрии прямой шестиугольной призмы является D6h с порядком 24, а группой вращений является D6 с порядком 12.

Объём[править | править код]

Как и у большинства призм, объём правильной шестигранной призмы можно найти умножением площади основания (с длиной стороны a) на высоту h, что даёт формулу[3]:

{displaystyle V={frac {3{sqrt {3}}}{2}}a^{2}times h}

Симметрия[править | править код]

Топология однородной шестиугольной призмы могут иметь геометрические вариации с низкой симметрией:

Симметрия D6h, [2,6], (*622) C6v, [6], (*66) D3h, [2,3], (*322) D3d, [2+,6], (2*3)
Конструкция {6}×{}, CDel node 1.pngCDel 2.pngCDel node 1.pngCDel 6.pngCDel node.png t{3}×{}, CDel node 1.pngCDel 2.pngCDel node 1.pngCDel 3.pngCDel node 1.png CDel node 1.pngCDel 2.pngCDel node f1.pngCDel 3.pngCDel node f1.png s2{2,6}, CDel node h.pngCDel 2x.pngCDel node h.pngCDel 6.pngCDel node 1.png
Рисунок Hexagonal Prism.svg Hexagonal frustum.png Truncated triangle prism.png Cantic snub hexagonal hosohedron.png
Нарушение Hexagonal frustum2.png Truncated triangle prism2.png Isohedral hexagon prism.png
Isohedral hexagon prism2.png 		Cantic snub hexagonal hosohedron2.png

Как часть пространственных мозаик[править | править код]

Шестигранная призма присутствует как ячейка в четырёх призматических однородных выпуклых сотах[en] в трёхмерном пространстве:

Шестигранные призмы существуют также в качестве трёхмерных граней четырёхмерных однородных многогранников[en]:

Усечённая тетраэдральная призма[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 2.pngCDel node 1.png 		Усечённая октаэдральная призма[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 4.pngCDel node.pngCDel 2.pngCDel node 1.png 		Усечённая кубоктаэдрическая призма[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 4.pngCDel node 1.pngCDel 2.pngCDel node 1.png 		Усечённая икосаэдрическая призма[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 5.pngCDel node.pngCDel 2.pngCDel node 1.png 		Усечённая икосододекаэдрическая призма[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 5.pngCDel node 1.pngCDel 2.pngCDel node 1.png
Truncated tetrahedral prism.png Truncated octahedral prism.png Truncated cuboctahedral prism.png Truncated icosahedral prism.png Truncated icosidodecahedral prism.png
Усечённая внутрь 5-ячейка[en]
CDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.png 		Рёберно усечённая 5-ячейка[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.png 		Усечённая внутрь 16-ячейка[en]
CDel node 1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.png 		Рёберно усечённый гиперкуб[en]
CDel node 1.pngCDel 4.pngCDel node 1.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.png
4-simplex t013.svg 4-simplex t0123.svg 4-cube t023.svg 4-cube t0123.svg
Усечённая внутрь 24-ячейка[en]
CDel node 1.pngCDel 3.pngCDel node.pngCDel 4.pngCDel node 1.pngCDel 3.pngCDel node 1.png 		Рёберно усечённая 24-ячейка[en]
CDel node 1.pngCDel 3.pngCDel node 1.pngCDel 4.pngCDel node 1.pngCDel 3.pngCDel node 1.png 		Усечённая внутрь 600-ячейка[en]
CDel node 1.pngCDel 5.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.png 		Рёберно усечённая 120-ячейка[en]
CDel node 1.pngCDel 5.pngCDel node 1.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node 1.png
24-cell t0123 F4.svg 24-cell t013 F4.svg 120-cell t023 H3.png 120-cell t0123 H3.png

Связанные многогранники и мозаики[править | править код]

Однородные шестиугольные диэдральные сферические многогранники

Симметрия: [6,2], (*622) [6,2]+, (622) [6,2+], (2*3)
Hexagonal dihedron.png Dodecagonal dihedron.png Hexagonal dihedron.png Spherical hexagonal prism.png Spherical hexagonal hosohedron.png Spherical truncated trigonal prism.png Spherical dodecagonal prism2.png Spherical hexagonal antiprism.png Spherical trigonal antiprism.png
CDel node 1.pngCDel 6.pngCDel node.pngCDel 2.pngCDel node.png CDel node 1.pngCDel 6.pngCDel node 1.pngCDel 2.pngCDel node.png CDel node.pngCDel 6.pngCDel node 1.pngCDel 2.pngCDel node.png CDel node.pngCDel 6.pngCDel node 1.pngCDel 2.pngCDel node 1.png CDel node.pngCDel 6.pngCDel node.pngCDel 2.pngCDel node 1.png CDel node 1.pngCDel 6.pngCDel node.pngCDel 2.pngCDel node 1.png CDel node 1.pngCDel 6.pngCDel node 1.pngCDel 2.pngCDel node 1.png CDel node h.pngCDel 6.pngCDel node h.pngCDel 2x.pngCDel node h.png CDel node.pngCDel 6.pngCDel node h.pngCDel 2x.pngCDel node h.png
{6,2} t{6,2} r{6,2} t{2,6} {2,6} rr{2,6} tr{6,2}[en] sr{6,2} s{2,6}
Двойственные им многогранники
Spherical hexagonal hosohedron.png Spherical dodecagonal hosohedron.png Spherical hexagonal hosohedron.png Spherical hexagonal bipyramid.png Hexagonal dihedron.png Spherical hexagonal bipyramid.png Spherical dodecagonal bipyramid.png Spherical hexagonal trapezohedron.png Spherical trigonal trapezohedron.png
V62 V122 V62 V4.4.6[en] V26 V4.4.6[en] V4.4.12 V3.3.3.6[en] V3.3.3.3

Этот многогранник можно считать членом последовательности однородных многогранников с угловой фигурой (4.6.2p) и диаграммой Коксетера — Дынкина CDel node 1.pngCDel p.pngCDel node 1.pngCDel 3.pngCDel node 1.png. Для p < 6 членами последовательности являются усечённые во всех углах многогранники (зоноэдры), и они показаны ниже как сферические мозаики. Для p > 6 они являются мозаиками гиперболической плоскости начиная с усечённой трисемиугольной мозаики[en].

*n32 мутации по симметрии полностью усечённых мозаик: 4.6.2n

Симметрия
*n32[en]
n,3[en] 		Сферическая Евклидова Компактная гиперболическая Паракомп. Некомпактная гиперболическая
*232
[2,3] 		*332
[3,3] 		*432
[4,3] 		*532
[5,3] 		*632
[6,3] 		*732
[7,3] 		*832
[8,3] 		*∞32
[∞,3] 		 
[12i,3] 		 
[9i,3] 		 
[6i,3] 		 
[3i,3]
Фигуры Spherical truncated trigonal prism.png Uniform tiling 332-t012.png Uniform tiling 432-t012.png Uniform tiling 532-t012.png Uniform polyhedron-63-t012.png H2 tiling 237-7.png H2 tiling 238-7.png H2 tiling 23i-7.png H2 tiling 23j12-7.png H2 tiling 23j9-7.png H2 tiling 23j6-7.png H2 tiling 23j3-7.png
Конфигурация 4.6.4 4.6.6 4.6.8 4.6.10 4.6.12[en] 4.6.14[en] 4.6.16[en] 4.6.∞[en] 4.6.24i 4.6.18i 4.6.12i 4.6.6i
Двойственная Spherical hexagonal bipyramid.png Spherical tetrakis hexahedron.png Spherical disdyakis dodecahedron.png Spherical disdyakis triacontahedron.png Tiling Dual Semiregular V4-6-12 Bisected Hexagonal.svg H2checkers 237.png H2checkers 238.png H2checkers 23i.png H2 checkers 23j12.png H2 checkers 23j9.png H2 checkers 23j6.png H2 checkers 23j3.png
Конфигурация грани V4.6.4[en] V4.6.6 V4.6.8[en] V4.6.10 V4.6.12[en] V4.6.14[en] V4.6.16[en] V4.6.∞ V4.6.24i V4.6.18i V4.6.12i V4.6.6i

См. также[править | править код]

Семейство правильных призм

Многоугольник Triangular prism.png Tetragonal prism.png Pentagonal prism.png Hexagonal prism.png Prism 7.png Octagonal prism.png Prism 9.png Decagonal prism.png Hendecagonal prism.png Dodecagonal prism.png Heptadecagonal Prism.svg Circular cylinder rh.svg
Мозаика Spherical triangular prism.png Spherical square prism.png Spherical pentagonal prism.png Spherical hexagonal prism.png Spherical heptagonal prism.png Spherical octagonal prism.png Spherical decagonal prism.png Infinite prism tiling.png
Конфигурация 3.4.4 4.4.4 5.4.4 6.4.4 7.4.4 8.4.4 9.4.4 10.4.4 11.4.4 12.4.4 17.4.4 ∞.4.4

Примечания[править | править код]

  1. ↑ 1 2 Anthony Pugh. Polyhedra: A Visual Approach. — University of California Press, 1976. — С. 21, 27, 62. — ISBN 9780520030565.
  2. ↑ Audrey Simpson. Core Mathematics for Cambridge IGCSE. — Cambridge University Press, 2011. — С. 266–267. — ISBN 9780521727921.
  3. ↑ Carolyn C. Wheater. Geometry. — Career Press, 2007. — С. 236–237. — ISBN 9781564149367.

Ссылки[править | править код]

  • Uniform Honeycombs in 3-Space Модели в формате VRML
  • The Uniform Polyhedra
  • Virtual Reality Polyhedra The Encyclopedia of Polyhedra
  • Prisms and antiprisms
  • Weisstein, Eric W. Hexagonal prism (англ.) на сайте Wolfram MathWorld.
  • Hexagonal Prism Interactive Model — Просмотр призм в браузере
Источник

Рассмотрением свойств и особенностей правильных фигур в трехмерном пространстве занимается стереометрия. Одним из многочисленных классов объемных фигур являются призмы. В данной статье приведем формулу объема призмы шестиугольной и покажем, как ее следует использовать при решении задачи.

Что такое шестиугольная призма?

Коротко отвечая на поставленный вопрос, следует сказать, что любая призма, имеющая в основании плоский многоугольник с шестью углами и шестью сторонами, называется шестиугольной. Этот многоугольник называется основанием фигуры. Рисунок ниже показывает, как выглядит такая призма.

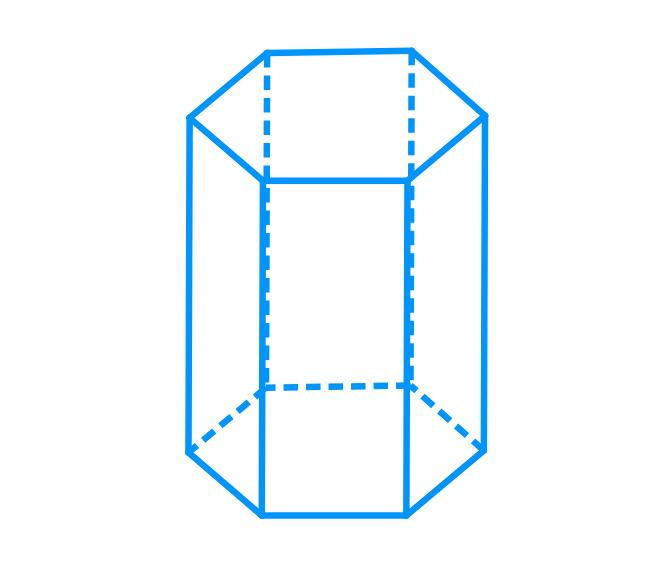
Шестиугольная призма

Видно, что фигура образована двумя одинаковыми шестиугольными основаниями, которые расположены в параллельных плоскостях. Соединены они с помощью шести параллелограммов. Призма имеет 8 граней, 18 ребер и 12 вершин.

Если все параллелограммы, которые образуют боковую поверхность, представляют собой прямоугольники или квадраты, то фигура будет прямой. У прямой призмы расстояние между основаниями (высота) совпадает с длиной бокового ребра. Если основания прямой фигуры являются равносторонними и равноугольными, то можно говорить о правильной шестиугольной призме.

Определение объема

Теперь ответим на вопрос, как найти объем призмы шестиугольной. Формула для объем произвольной призмы имеет следующий вид:

V = So*h

Где So – площадь основания, h – высота фигуры. Если мы имеем правильную призму с шестиугольником в основании, то его площадь рассчитать можно, пользуясь следующим выражением:

So = 3*√3/2*a2

Где латинской буквой a обозначена длина ребра основания. Это выражение можно получить самостоятельно, если разделить правильный шестиугольник на шесть одинаковых равносторонних треугольников, а затем сложить их площади. Длины сторон треугольников при таком делении будут равны a.

Учитывая выражение для So, можно привести формулу объема призмы шестиугольной правильной. Она будет иметь такой вид:

V = 3*√3/2*a2*h

Выражение показывает, что для определения величины V правильной призмы достаточно знать всего два ее линейных размера.

Если в основании прямой фигуры будет находиться неправильный шестиугольник, тогда следует применить геометрический анализ, чтобы определить его площадь So.

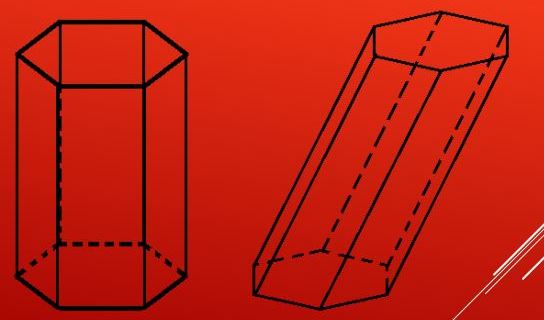
Прямая и наклонная призмы

Самым сложным случаем определения объема шестиугольной призмы является ситуация с наклонной фигурой. Для нее высота уже не равна длине бокового ребра. Чтобы ее вычислить, следует знать какой-либо вертикальный угол (либо между основанием и боковой гранью, либо между ребрами боковым и основания).

Пример задачи

Дана правильная призма шестиугольная. Известно, что ее самая длинная объемная диагональ составляет 25 см. Угол между ней и плоскостью основания составляет 30o. Чему равен объем геометрической фигуры?

Шестиугольная призма из бумаги

Чтобы воспользоваться полученной выше формулой объема призмы шестиугольной, следует вычислить параметры a и h. Самая длинная объемная диагональ соединяет противоположные вершины разных оснований. Знание ее длины и угла между ней и основанием позволяет вычислить высоту и длину ребра шестиугольника с помощью следующих формул:

a = 25*cos(30o)/2 = 10,825 см;

h = 25*sin(30o) = 12,5 см

Теперь можно вычислить V:

V = 3*√3/2*a2*h = 3*√3/2*10,8252*12,5 = 3805,55 см3

Таким образом, искомый объем равен приблизительно 3,8 литра.

Источник

Добавить комментарий