В данной публикации мы рассмотрим, как можно найти объем куба и разберем примеры решения задач для закрепления материала.
- Формула вычисления объема куба
- Примеры задач
Формула вычисления объема куба
1. Через длину ребра
Объем (V) куба равняется произведению его длины на ширину на высоту. Т.к. данные величины у куба равны, следовательно, его объем равен кубу любого ребра.
V = a ⋅ a ⋅ a = a3

2. Через длину диагонали грани
Как мы знаем, грани куба равны между собой и являются квадратом, сторона которого может быть найдена через длину диагонали по формуле: a=d/√2.

Следовательно, вычислить объем куба можно так:
![]()
Примеры задач
Задание 1
Вычислите объем куба, если его ребро равняется 5 см.
Решение:
Подставляем в формулу заданное значение и получаем:
V = 5 см ⋅ 5 см ⋅ 5 см = 125 см3.
Задание 2
Известно, что объем куба равен 512 см3. Найдите длину его ребра.
Решение:
Пусть ребро куба – это a. Выведем его длину из формулы расчета объема:![]()
Задание 3
Длина диагонали грани куба составляет 12 см. Найдите объем фигуры.
Решение:
Применим формулу, в которой используется диагональ грани:
![]()
Куб – это трехмерная фигура, представляющая собой правильный многогранник, все грани которого квадраты. Чтобы найти объем куба достаточно знать только длину его стороны (они у куба равны).
Чтобы найти объем куба можно воспользоваться калькулятором, либо одной из подходящих формул, которые мы приводим ниже.
Содержание:
- калькулятор объема куба
- формула объема куба через ребро
- формула объема куба через диагональ грани
- формула объема куба через периметр грани
- формула объема куба через диагональ куба
- формула объема куба через площадь полной поверхности
- примеры задач
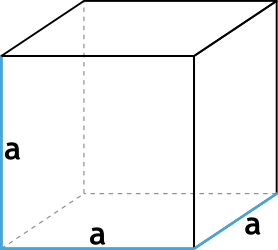
Формула объёма куба через ребро
Формула объёма куба через диагональ грани
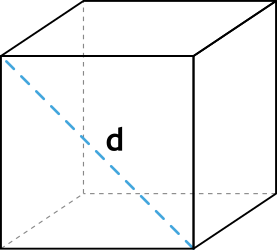
{V = Big( dfrac{d}{sqrt{2}} Big) ^3}
d – диагональ грани куба
Формула объёма куба через периметр грани

{V= Big( dfrac{P}{4} Big) ^3}
P – периметр грани куба
Формула объёма куба через диагональ куба

{V= dfrac{D^3}{3sqrt{3}}}
D – диагональ куба
Формула объёма куба через площадь полной поверхности
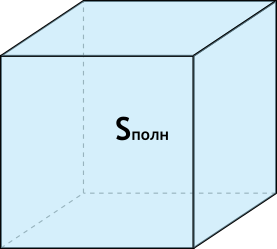
{V= dfrac{sqrt{{S_{полн}}^3}}{6sqrt{6}}}
Sполн – диагональ куба
Примеры задач на нахождение объема куба
Задача 1
Чему равен объём куба с ребром 5 см?
Решение
Для нахождения объема куба, когда известа длина ребра, воспользуемся первой формулой:
V=a ^ 3 = 5 ^ 3 = 125 : см^3
Ответ: 125 см³
Воспользуемся калькулятором для проверки полученного результата.
Задача 2
Найти объем куба, если площадь его поверхности равна 96 см².
Решение
В данном примере нам подойдет эта формула:
V= dfrac{sqrt{{S_{полн}}^3}}{6sqrt{6}} = dfrac{sqrt{{96}^3}}{6sqrt{6}} = dfrac{sqrt{96 cdot 96 cdot 96}}{6sqrt{6}} = dfrac{96 sqrt{96}}{6sqrt{6}} = dfrac{96 sqrt{16 cdot 6}}{6sqrt{6}} = dfrac{96 cdot 4 sqrt{6}}{6sqrt{6}} = dfrac{384 sqrt{6}}{6sqrt{6}} = 64 : см^3
Ответ: 64 см³
Проверить ответ поможет калькулятор .
Также на нашем сайте вы можете найти объем конуса.
Загрузить PDF
Загрузить PDF
Куб — трехмерная геометрическая фигура, у которой все ребра равны (длина равна ширине и равна высоте). У куба шесть квадратных граней, которые пересекаются под прямым углом и стороны которых равны. Вычислить объем куба легко — нужно перемножить длину, ширину и высоту. Так как у куба длина равна ширине и равна высоте, то объем куба равен s3, где s — длина одного (любого) ребра куба.
-

1
Найдите длину одного ребра куба. Как правило, длина ребра куба дана в условии задачи. Если вы вычисляете объем реального объекта кубической формы, измерьте его ребро линейкой или рулеткой.
- Рассмотрим пример. Ребро куба равно 5 см. Найдите объем куба.
-

2
Возведите в куб длину ребра куба. Другими словами, умножьте длину ребра куба саму на себя три раза. Если s — длина ребра куба, то s * s *s = s3 и, таким образом, вы вычислите объем куба.
- Этот процесс аналогичен процессу нахождения площади основания куба (равна произведению длины на ширину квадрата в основании) и последующему умножению площади основания на высоту куба (то есть, другими словами, вы умножаете длину на ширину и на высоту). Так как в кубе длина ребра равна ширине и равна высоте, то этот процесс можно заменить возведением ребра куба в третью степень.
- В нашем примере объем куба равен 5 * 5 *5 = 53 = 125.
-

3
К ответу припишите единицы измерения объема (если вы этого не сделаете, ваша оценка может быть снижена). Так как объем — это количественная характеристика пространства, занимаемого телом, то единицами измерения объема являются кубические единицы (кубические сантиметры, кубические метры и так далее).
- В нашем примере размер ребра куба давался в сантиметрах, поэтому объем будет измеряться в кубических сантиметрах (или в см3). Итак, объем куба равен 125 см3.
- Если размер ребра куба дается в других единицах, то и объем куба измеряется в соответствующих кубических единицах. Например, если ребро куба равно 5 м (а не 5 см), то его объем равен 125 м3.
Реклама
-

1
В некоторых задачах длина ребра куба не дана, но даны другие величины, с помощью которых можно найти ребро куба и его объем. Например, если вам дана площадь поверхности куба, то разделите ее на 6, из полученного значения извлеките квадратный корень и вы найдете длину ребра куба. Затем возведите длину ребра куба в третью степень и вычислите объем куба.
- Площадь поверхности куба равна 6s2, где s — длина ребра куба (то есть вы находите площадь одной грани куба, а затем умножаете ее на 6, так как у куба 6 равных граней).
- Рассмотрим пример. Площадь поверхности куба равна 50 см2. Найдите объем куба.
-

2
Разделите площадь поверхности куба на 6 (так как у куба 6 равных граней, вы получите площадь одной грани куба). В свою очередь площадь одной грани куба равна s2, где s — длина ребра куба.
- В нашем примере: 50/6 = 8,33 см2 (не забывайте, что площадь измеряется в квадратных единицах — см2, м2 и так далее).
-

3
Так как площадь одной грани куба равна s2, то извлеките квадратный корень из значения площади одной грани и получите длину ребра куба.
- В нашем примере, √8,33 = 2,89 см.
-

4
Возведите в куб полученное значение, чтобы найти объем куба (как описано в предыдущем разделе).
- В нашем примере: 2,89 * 2,89 * 2,89 = 2,893 = 24,14 см3. К ответу не забудьте приписать кубические единицы.
Реклама
-

1
Разделите диагональ одной из граней куба на √2, чтобы найти длину ребра куба. Таким образом, если в задаче дана диагональ грани (любой) куба, то вы можете найти длину ребра куба, разделив диагональ на √2.
- Рассмотрим пример. Диагональ грани куба равна 7 см. Найдите объем куба. В этом случае длина ребра куба равна 7/√2 = 4,96 см. Объем куба равен 4,963 = 122,36 см3.
- Запомните: d2 = 2s2, где d — диагональ грани куба, s — ребро куба. Эта формула вытекает из теоремы Пифагора, согласно которой квадрат гипотенузы (в нашем случае диагональ грани куба) прямоугольного треугольника равен сумме квадратов катетов (в нашем случае ребер), то есть d2 = s2 + s2 = 2s2.
-

2
Разделите диагональ куба на √3, чтобы найти длину ребра куба. Таким образом, если в задаче дана диагональ куба, то вы можете найти длину ребра куба, разделив диагональ на √3. Диагональ куба — отрезок, соединяющий две вершины, симметричные относительно центра куба, равный D2 = 3s2 (где D — диагональ куба, s — ребро куба).
- Эта формула вытекает из теоремы Пифагора, согласно которой квадрат гипотенузы (в нашем случае диагональ куба) прямоугольного треугольника равен сумме квадратов катетов (в нашем случае один катет — это ребро, а второй катет — это диагональ грани куба, равная 2s2), то есть D2 = s2 + 2s2 = 3s2.
- Рассмотрим пример. Диагональ куба равна 10 м. Найдем объем куба:
- D2 = 3s2
- 102 = 3s2
- 100 = 3s2
- 33,33 = s2
- 5,77 м = s
- Объем куба равен 5,773 = 192,45 м3
Реклама
Об этой статье
Эту страницу просматривали 605 392 раза.
Была ли эта статья полезной?
Куб (или гексаэдр) — это правильный многогранник, который состоит из многоугольников, являющихся квадратами.
У куба 12 ребер – отрезков, которые являются сторонами квадратов (граней куба).
Также он имеет 8 вершин и 6 граней.
Онлайн-калькулятор объема куба
Формула объема куба
Для нахождения объема куба нужно перемножить его измерения – длину, ширину и высоту. Исходя из того, что куб состоит из квадратов, все его измерения одинаковы и численно равны длине ребра.
Формула для вычисления объема куба такова:
V=a3V=a^3
где aa — длина ребра куба.
Рассмотрим несколько примеров.
Найти объем куба, если периметр PP его грани aa равен 16 cм.16text{ cм.}
Решение
P=16P=16
Периметр PP грани куба связан с длиной его ребра aa по формуле:
P=a+a+a+a=4⋅aP=a+a+a+a=4cdot a
16=4⋅a16=4cdot a
a=164=4a=frac{16}{4}=4
Найдем объем нашего тела:
V=a3=43=64 см3V=a^3=4^3=64text{ см}^3
Ответ: 64 см3.64text{ см}^3.
Одна четвертая часть диагонали квадрата равна 3 см.3text{ см.} Найти объем куба, образованного данным четырехугольником.
Решение
Пусть dd — диагональ фигуры, тогда по условию:
d4=3frac{d}{4}=3
d=4⋅3=12d=4cdot 3=12
Найдем сторону этого квадрата. Обратимся за помощью к теореме Пифагора:
a2+a2=12a^2+a^2=12,
где aa — сторона квадрата.
2⋅a2=122cdot a^2=12
a=6a=sqrt{6}
Приходим к окончательным расчетам для объема:
V=a3=(6)3=66 см3V=a^3=(sqrt{6})^3=6sqrt{6}text{ см}^3
Ответ: 66 см3.6sqrt{6}text{ см}^3.
Чуть более сложный пример.
В куб вписан шар, площадь SS которого равна 64π64pi. Найти объем куба.
Решение
S=64πS=64pi
Первый шагом является нахождение радиуса RR данного шара. Формула его площади такова:
S=4⋅π⋅R2S=4cdotpicdot R^2
64π=4⋅π⋅R264pi=4cdotpicdot R^2
64=4⋅R264=4cdot R^2
644=R2frac{64}{4}=R^2
16=R216=R^2
R=4R=4
Для куба радиус вписанного шара является половиной его стороны aa:
a=2⋅R=2⋅4=8a=2cdot R=2cdot4=8
Объем вычисляется следующим образом:
V=a3=83=512 см3V=a^3=8^3=512text{ см}^3
Ответ: 512 см3.512text{ см}^3.
На Студворке вы можете оформить заказ контрольных работ для студентов по самым низким ценам!
Тест по теме «Объем куба»
Download Article
Download Article
A cube is a three-dimensional shape that has equal width, height, and length measurements. A cube has six square faces, all of which have sides of equal length and all of which meet at right angles.[1]
Finding the volume of a cube is a snap – generally, all that’s needed is to multiply the cube’s length × width × height. Since a cube’s sides are all equal in length, another way of thinking of a cube’s volume is s3, where s is the length of one of the cube’s sides. See Step 1 below for a detailed breakdown of these processes.
Help Finding Volume of a Cube
-

1
Find the length of one side of the cube.[2]
Often, in problems asking you to find the volume of a cube, you’ll be given the length of one of a cube’s sides. If you have this information, you have all you need to solve for the cube’s volume. If you’re not solving an abstract math problem but are instead attempting to find the volume of a real-life object shaped like a cube, use a ruler or measuring tape to measure the side of the cube.[3]
- To better understand the process of finding the volume of a cube, let’s follow along with an example problem as we go through the steps in this section. Let’s say the side of the cube is 2 inches (5.08 cm) long. We’ll use this information to find the volume of the cube in the next step.
-

2
Cube the length of the side. When you’ve found the length of one of the cube’s sides, cube this number. In other words, multiply it by itself twice. If s is the length of the side, you would multiply s × s × s (or, in simplified form, s3). This will give you the volume of your cube![4]
[5]
- This process is essentially the same as finding the area of the base and then multiplying it by the cube’s height (or, in other words, length × width × height), since the area of the base is found by multiplying its length and its width. Since the length, width, and height of a cube are equal, we can shorten this process by simply cubing any of these measurements.
- Let’s proceed with our example. Since the length of the side of our cube is 2 inches, we can find the volume by multiplying 2 x 2 x 2 (or 23) = 8.
Advertisement
-

3
Label your answer with cubic units.[6]
Since volume is the measure of three-dimensional space, your answer should be in cubic units by definition. Often, on math schoolwork, neglecting to label your answer with the right units can cause you to lose points on a problem, so don’t forget to use the correct label!- In our example, since our original measurement was in inches, our final answer will be labelled with the units “cubic inches” (or in3). So, our answer of 8 becomes 8 in3.
- If we had used a different initial unit of measurement, our final cubic units would differ. For instance, if our cube had sides with lengths of 2 meters, rather than 2 inches, we would label it with cubic meters (m3).
Advertisement
-

1
Find your cube’s surface area. While the easiest way to find a cube’s volume is to cube the length of one of its sides, it’s not the only way. The length of a cube’s side or the area of one of its faces can be derived from several other of the cube’s properties, which means that if you start with one of these pieces of information, you can find the volume of the cube in a roundabout manner. For instance, if you know a cube’s surface area, all you need to do to find its volume is to divide the surface area by 6, then take the square root of this value to find the length of the cube’s sides. From here, all you’ll need to do is cube the length of the side to find the volume as normal. In this section, we’ll walk through this process step-by-step.
- The surface area of a cube is given via the formula 6s2, where s is the length of one of the cube’s sides. This formula is essentially the same as finding the 2-dimensional area of the cube’s six faces and adding these values together. We’ll use this formula to find the volume of the cube from its surface area.[7]
- As a running example, let’s say that we have a cube whose surface we know to be 50 cm2, but we don’t know its side lengths. In the next few steps, we’ll use this information to find the cube’s volume.
- The surface area of a cube is given via the formula 6s2, where s is the length of one of the cube’s sides. This formula is essentially the same as finding the 2-dimensional area of the cube’s six faces and adding these values together. We’ll use this formula to find the volume of the cube from its surface area.[7]
-

2
Divide the cube’s surface area by 6. Since the cube has 6 faces with equal area, dividing the cube’s surface area by 6 will give you the area of one of its faces. This area is equal to the lengths of two of its sides multiplied (l × w, w × h, or h × l).[8]
- In our example, dividing 50/6 = 8.33 cm2. Don’t forget that two-dimensional answers have square units (cm2, in2, and so on).
-

3
Take the square root of this value. Since the area of one of the cube’s faces is equal to s2 (s × s), taking the square root of this value will find you the length of one of the cube’s sides. Once you have this, you have enough information to solve for the volume of the cube as you normally would.[9]
- In our example, √8.33 is roughly 2.89 cm.
-

4
Cube this value to find the cube’s volume. Now that you’ve obtained a value for the length of the cube’s side, simply cube this value (multiply it by itself twice) to find the volume of the cube as detailed in the section above. Congratulations – you’ve found the volume of a cube from its surface area.[10]
- In our example, 2.89 × 2.89 × 2.89 = 24.14 cm3. Don’t forget to label your answer with cubic units.
Advertisement
-

1
Divide the diagonal across one of the cube’s faces by √2 to find the cube’s side length. By definition, the diagonal of a perfect square is √2 × the length of one of its sides. Thus, if the only information you’re given about a cube is regarding the diagonal length of one of its faces, you can find the side length for the cube by dividing this value by √2. From here, it’s relatively simple to cube your answer and find the volume of the cube as described above.[11]
- For instance, let’s say that one of a cube’s faces has a diagonal that is 7 feet long. We would find the side length of the cube by dividing 7/√2 = 4.96 feet. Now that we know the side length, we can find the volume of the cube by multiplying 4.963 = 122.36 feet3.
- Note that, in general terms, d2 = 2s2 where d is the length of the diagonal of one of the cube’s faces and s is the length of one of the sides of the cube. This is because, according to the Pythagorean theorem, the square of the hypotenuse of a right triangle is equal to the sums of the squares of the other two sides. Thus, because the diagonal of a cube’s face and two of the sides on that face form a right triangle, d2 = s2 + s2 = 2s2.
-

2
Square the diagonal of two opposite corners of the cube, then divide by 3 and take the square root to find the side length. If the only piece of information you’re given about a cube is the length of a 3-dimensional line segment stretching diagonally from one corner of the cube to the corner opposite it, it’s still possible to find the volume of the cube. Because d forms one of the sides of a right triangle that has the diagonal between the two opposite corners of the cube as a hypotenuse, we can say that D2 = 3s2, where D = the 3-dimensional diagonal between opposite corners of the cube.[12]
- This is because of the Pythagorean Theorem. D, d, and s form a right triangle with D as the hypotenuse, so we can say that D2 = d2 + s2. Since we calculated above that d2 = 2s2, we can say that D2 = 2s2 + s2 = 3s2.
- As an example, let’s say that we know that the diagonal from one of the corners in the base of the cube to the opposite corner in the “top” of the cube is 10 m. If we want to find the volume, we would insert 10 for each “D” in the equation above as follows:
- D2 = 3s2.
- 102 = 3s2.
- 100 = 3s2
- 33.33 = s2
- 5.77 m = s. From here, all we need to do to find the volume of the cube is to cube the side length.
- 5.773 = 192.45 m3
Advertisement
Add New Question
-
Question
If the volume of a cube is 512 cubic meters, what are its side measurements?

The side length is the cube root of the volume.
-
Question
A cube-shaped structure has sides 3 feet long. If I plan to fill it with gravel to a depth of 2.5 feet, how much gravel will I need?

Multiply the area of the bottom of the cube by 2.5.
-
Question
If the length is 2 meters, the height is 1 meter and the width is 2 meters of a water tank, how many gallons of water will the cube contain?

First, you need to find the volume of the tank. This can be found by multiplying the length, height, and width altogether. When you do that, you get 4 cubic meters. Doing some research, you will find that 1 cubic meter is approximately equal to about 220 imperial gallons or 264.2 US liquid gallons. If you use the imperial gallons, then the tank would hold a little less than 880 imperial gallons. But if you use the US liquid gallons, it would hold slightly less than 1,057 gallons.
See more answers
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
Advertisement
References
About This Article
Article SummaryX
To calculate the volume of a cube, find the length of one of the sides of the cube. When you have this measurement, multiply it by itself 2 times to get the volume, which is called “cubing” the number. For example, if your cube has a length of 2, you would multiply 2 × 2 × 2 to get a volume of 8. Be sure to include the units cubed with your answer. To learn more, like how to find the volume if you have the cube’s surface area, keep reading!
Did this summary help you?
Thanks to all authors for creating a page that has been read 1,657,157 times.
Reader Success Stories
-

Carla Trollope-Davis
Mar 1, 2017
“I have the tendency to get confused when reading math problems and explanations. I found that your combination of…” more
