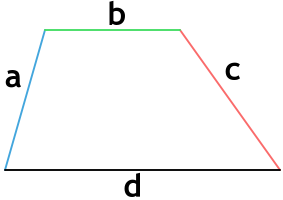
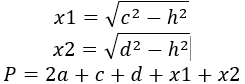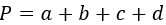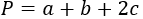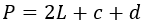Как найти периметр трапеции если даны основания

Как найти периметр трапеции если даны основания

Как найти периметр трапеции

Содержание:

  • Основные свойства трапеции
  • Способы нахождений периметра

    • По всем сторонам
    • По сторонам равнобедренной трапеции
    • Через среднюю линию
  • Примеры решения задач

Определения

​Трапеция — это четырехугольник, у которого лишь одна пара противолежащих сторон параллельна.

Периметр трапеции — это сумма длин всех его сторон.

Основные свойства трапеции

  • средняя линия трапеции параллельна ее основаниям, а также равна половине их суммы;

Свойство 1

 
  • биссектриса любого угла данного четырехугольника отсекает на его основании отрезок, равный боковой стороне;

Свойство 2

 
  • треугольники ABO и DCO (на картинке), образованные диагоналями фигуры и ее основаниями, подобны;

Свойство 3

 
  • треугольники OAB и OCD, образованные диагоналями трапеции и ее боковыми сторонами, имеют одинаковую площадь;

Свойство 4

 
  • если сумма длин оснований четырехугольника равна сумме его боковых ребер, то в фигуру можно вписать окружность;

Свойство 5

 
  • точки M и N середины диагоналей лежат на одной прямой со средней линией фигуры. Также отрезок MN равен полуразность оснований четырехугольника;

Свойство 6

 
  • середины оснований фигуры, точка пересечения ее диагоналей, а также точка пересечения продолжений ее боковых сторон лежат на одной прямой;

Свойство 7

 

Свойства равнобедренной трапеции

Осторожно! Если преподаватель обнаружит плагиат в работе, не избежать крупных проблем (вплоть до отчисления). Если нет возможности написать самому, закажите тут.

  • в равнобедренной трапеции углы при обоих ее основаниях одинаковы;
  • диагонали равны;
  • равнобедренную трапецию всегда можно вписать в окружность или описать окружность вокруг;
  • если диагонали перпендикулярны, то высота фигуры равна полусумме ее оснований.

Способы нахождений периметра

Рассмотрим способы, с помощью которых можно найти сумму длин всех сторон данного четырехугольника.

По всем сторонам

Периметр по всем сторон

 

Формула для нахождения периметра выглядит так:

P=a+b+c+d

где a, b, c, d — стороны трапеции.

По сторонам равнобедренной трапеции

Периметр по сторон 2

 

Если нам известны ребра этого четырехугольника с одинаковыми боковыми сторонами, то находить ее P можно по следующей формуле:

(P=2times a+b+c)

или

(P=2times c+a+b)

Через среднюю линию

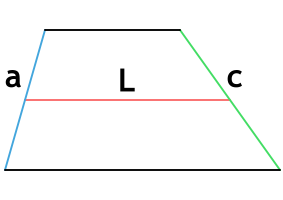
Через среднюю линию

 

Так как средняя линия трапеции равна полусумме ее оснований, то формулу P можно выразить так:

(P=2times l+AB+CD)

где l — средняя линия фигуры.

Примеры решения задач

Давайте рассмотрим наглядные примеры решения задач на нахождение суммы длин всех ребер этой фигуры.

Задача 1

Дана трапеция с боковыми сторонами 4 см и 5 см, а ее основания равны 7 см и 10 см. Найти периметр данного многоугольника.

Решение:

Нам пригодится самая первая формула для расчета:

P=a+b+c+d.

Подставляем значения и получаем:

P=4+7+5+10=26;см.

Ответ: 26 см.

Задача 2

Известно, что у трапеции две боковые стороны равны 7 см, а ее основания равны 5 см и 8 см. Нужно найти P четырехугольника.

Решение:

Так как трапеция равнобедренная, удобнее всего будет использовать формулу:

(P=2times a+b+c)

Таким образом, получается:

(P=2times 7+5+8=27) см.

Ответ: 27 см.

Задача 3

Средняя линия l трапеции равна 6 см, а боковые стороны 5 см и 9 см. Вычислить P фигуры.

Решение:

Считать будем по формуле

(P=2times l+a+c)

(P=2times 6+5+9=26) см.

Ответ: 26 см.

Насколько полезной была для вас статья?

Рейтинг: 3.82 (Голосов: 11)

Выделите текст и нажмите одновременно клавиши «Ctrl» и «Enter»

Текст с ошибкой:

Расскажите, что не так

Поиск по содержимому

Источник

Периметр трапеции калькулятор онлайн умеет вычислять периметр четырьмя способами:

  1. По четырем сторонам.
  2. По основанию и боковой стороне равнобедренной трапеции.
  3. По средней линии и боковым сторонам.
  4. По высоте и верхнему основанию и боковым сторонам.

Сделав расчет периметра на этом онлайн калькуляторе Вы получите не только ответ, но и детальное, пошаговое решение с выводом формул и промежуточных действий.

Трапеция

Трапеция – это четырехугольник, у которого две стороны параллельны (основания), а две другие не параллельны (боковые стороны).
Формула периметра трапеции: Формула периметра трапеции по верхнему основанию, высоте и боковым сторонам
где a – верхнее основание, h – высота, с и d – боковые стороны.

Решение:

x1 = √c2 – h2

= √62 – 52

= √36 – 25

= 3.317

x2 = √d2 – h2

= √42 – 52

= √16 – 25

= NAN

P = 2a + c + d + x1 + x2

= 2·2 + 6 + 4 + 3.317 + NAN

=

NAN

Ответ: Периметр трапеции с верхним основанием a = 2 высотой h = 5 и боковыми сторонами с = 6, d = 4 равен NAN

Периметр трапеции – это сумма четырех сторон. 
Так как, довольно часто, не все стороны известны, то периметр может быть найден и по другим формулам.  Вывод этих формул основан на правилах геометрии и тригонометрии.

Как найти периметр трапеции?

Найти периметр трапеции очень просто на нашем онлайн калькуляторе. Так же периметр может быть найден самостоятельно по формулам. Выбор нужной формулы зависит от того какие данные известны.

1) По четырем сторонам


где a,b,c,d – стороны трапеции.

2) По основанию и боковой стороне равнобедренной трапеции


где a,b,c – стороны трапеции.

3) По средней линии и боковым сторонам


где c,d – боковые стороны и L – длина средней линии.

4) По высоте и верхнему основанию и боковым сторонам


ггде a – верхнее основание, h – высота, с и d – боковые стороны.

Скачать все формулы в формате Word

Источник

Рассчитать периметр трапеции с помощью калькулятора или самостоятельно по формуле поможет материалы этой страницы. Кроме того вы можете рассчитать периметр равнобедренной трапеции.

Содержание:
  1. калькулятор периметра трапеции
  2. формула периметра трапеции через 4 стороны
  3. формула периметра трапеции через среднюю линию и боковые стороны
  4. примеры задач

Трапеция – выпуклый четырехугольник у которого две стороны параллельны (их называют основаниями), а две другие стороны, которые называют боковые стороны, непараллельны.

Периметр других четырехугольников также можно рассчитать на сайте: квадрат, прямоугольник, параллелограмм, ромб.

Формула периметра трапеции через все стороны

Периметр трапеции через стороны

{P = a+b+c+d}

a, b, c и d – стороны трапеции

Формула периметра трапеции через среднюю линию и боковые стороны

Периметр трапеции через среднюю линию и боковые стороны

{P = a+c+2L}

a и c – боковые стороны трапеции

L – средняя линия трапеции

Пример задачи на нахождение периметра трапеции

Задача 1

Найдите периметр трапеции если ее основания равны 7см и 10см а боковые стороны 4см и 5см.

Решение

Применим первую формулу. Подставим в нее значения длин сторон трапеции и рассчитаем ее периметр:

P = a+b+c+d = 4+7+5+10 = 26 : см

Ответ: 26 см

Осталось проверить ответ с помощью калькулятора .

Источник
Определение трапеции

Трапеция — это геометрическая фигура, четырехугольник, у которого две противоположные стороны параллельны.

Эти параллельные стороны называют основаниями трапеции. Две другие стороны – это боковые стороны трапеции.

Онлайн-калькулятор периметра трапеции

Трапеции бывают равнобедренные, разносторонние и прямоугольные.
У трапеции есть так называемая средняя линия, которая показана на рисунке ниже. Она параллельна двум основаниям, а также равна их полусумме.

площадь трапеции

Формула периметра трапеции

Для нахождения периметра трапеции нужно сложить длины всех ее сторон.

Периметр трапеции

P=a+b+c+dP = a + b + c + d,

где a,b,c,da, b, c, d — стороны трапеции.

Рассмотрим задачи на нахождение периметра трапеции.

Задача 1

Найти периметр трапеции, стороны которой равны:

a=c=2a = c = 2 см,
d=5d = 5 см,
b=3b = 3 см.

Решение

Нам дана равнобедренная трапеция, так как две ее стороны одинаковой длины. Также известны все стороны фигуры. Воспользуемся формулой и подставим вместо aa, bb, cc, dd их численные значения:

P=2+3+2+5=12P = 2 + 3 + 2 + 5 = 12 (см).

Ответ: P=12P = 12 см.

Рассмотрим еще одну задачу на вычисление стороны равнобедренной трапеции.

Задача 2

У равнобедренной трапеции основания равны 5 см и 3 см, а ее периметр равен 16 см. Чему равны боковые стороны?

Решение

В данной задаче нам известен периметр равнобедренной трапеции и ее основания: P=16P = 16 см, d=5d = 5 см, b=3b = 3 см, но неизвестны боковые стороны.

Воспользуемся формулой нахождения периметра трапеции, обозначив боковую сторону буквой aa:

P=a+a+b+d=2a+b+dP = a + a + b + d = 2a + b + d

Далее выразим 2a2a и найдем длину боковой стороны:

2a=P−b−d2a = P – b – d

2a=16−3−5=16−8=82a = 16 – 3 – 5 = 16 – 8 = 8

a=82=4a = frac{8}{2} = 4 см.

Боковая сторона равнобедренной трапеции равна 4 см.

Ответ: 4 см.

Не знаете, где заказать контрольную работу онлайн? Наши эксперты помогут вам в выполнении работы!

Тест по теме “Периметр трапеции”

Источник


Download Article


Download Article

A trapezoid is defined as a quadrilateral with two parallel sides. As with any polygon, to find the perimeter of a trapezoid you need to add all four of its sides together. However, often you will be missing side lengths but have other information, such as the height of the trapezoid, or the angle measurements. Using this information, you can use rules of geometry and trigonometry to find the unknown lengths of sides.

  1. Image titled Find the Perimeter of a Trapezoid Step 1

    1

    Set up the formula for perimeter of a trapezoid. The formula is P=T+B+L+R, where P equals the perimeter of the trapezoid, and the variables T equals the length of the top base of the trapezoid, B equals the length of the bottom base, L equals the length of the left side, and R equals the length of the right side.[1]

  2. Image titled Find the Perimeter of a Trapezoid Step 2

    2

    Plug the side lengths into the formula. If you do not know the length of all four sides of the trapezoid, you cannot use this formula.

    • For example, if you have a trapezoid with a top base of 2 cm, a bottom base of 3 cm, and two side lengths of 1 cm, your formula will look like this:
      P=2+3+1+1

    Advertisement

  3. Image titled Find the Perimeter of a Trapezoid Step 3

    3

    Add the side lengths together. This will give you the perimeter of your trapezoid.

    4. Advertisement

  1. Image titled Find the Perimeter of a Trapezoid Step 4

    1

    Divide the trapezoid into a rectangle and two right triangles. To do this, draw the height from both top vertices.

    • If you cannot form two right triangles because one side of the trapezoid is perpendicular to the base, just note that this side will have the same measurement as the height, and divide the trapezoid into one rectangle and one right triangle.

  2. Image titled Find the Perimeter of a Trapezoid Step 5

    2

    Label each height line. Since these are opposite sides of a rectangle, they will be the same length.[2]

    • For example, if you have a trapezoid with a height of 6 cm, you should draw a line from each top vertex extending down to the bottom base. Label each line 6 cm.

  3. Image titled Find the Perimeter of a Trapezoid Step 6

    3

    Label the length of the middle section of the bottom base. (This is the bottom side of the rectangle.) The length will equal the length of the top base (the top side of the rectangle), because opposite sides of a rectangle are of equal length.[3]
     If you do not know the length of the top base, you cannot use this method.

    • For example, if the top base of the trapezoid is 6 cm, then the middle section of the bottom base is also 6 cm.

  4. Image titled Find the Perimeter of a Trapezoid Step 7

    4

    Set up the Pythagorean Theorem formula for the first right triangle. The formula is a^{{2}}+b^{{2}}=c^{{2}}, where c is the length of the hypotenuse of the right triangle (the side opposite the right angle), a is the height of the right triangle, and b is the length of the base of the triangle.[4]

  5. Image titled Find the Perimeter of a Trapezoid Step 8

    5

  6. Image titled Find the Perimeter of a Trapezoid Step 9

    6

    Square the known values in the equation. Then, subtract to isolate the b variable.

  7. Image titled Find the Perimeter of a Trapezoid Step 10

    7

    Take the square root to find the value of b. (For complete instructions on how to simplify square roots, you can read Simplify a Square Root.) The result will give you the value of the missing base of your first right triangle. Label this length on the base of your triangle.

  8. Image titled Find the Perimeter of a Trapezoid Step 11

    8

    Find the missing length of the second right triangle. To do this, set up the Pythagorean Theorem formula for the second triangle, and follow the steps to find the length of the missing side. If you are working with an isosceles trapezoid, which is a trapezoid in which the two non-parallel sides are the same length,[5]
     the two right triangles are congruent, so you can simply carry the value from the first triangle over to the second triangle.

  9. Image titled Find the Perimeter of a Trapezoid Step 12

    9

    Add up all the side lengths of the trapezoid. The perimeter of any polygon is the sum of all sides: P=T+B+L+R. For the bottom base, you will add the bottom side of the rectangle, plus the bases of the two triangles. You will likely have square roots in your answer. For complete instructions on how to add square roots, you can read the article Add Square Roots. You can also use a calculator to convert the square roots to decimals.

    10. Advertisement

  1. Image titled Find the Perimeter of a Trapezoid Step 13

    1

    Divide the trapezoid into a rectangle and two right triangles. To do this, draw the height from both top vertices.

    • If you cannot form two right triangles because one side of the trapezoid is perpendicular to the base, just note that this side will have the same measurement as the height, and divide the trapezoid into one rectangle and one right triangle.

  2. Image titled Find the Perimeter of a Trapezoid Step 14

    2

    Label each height line. Since these are opposite sides of a rectangle, they will be the same length.[6]

    • For example, if you have a trapezoid with a height of 6 cm, you should draw a line from each top vertex extending down to the bottom base. Label each line 6 cm.

  3. Image titled Find the Perimeter of a Trapezoid Step 15

    3

    Label the length of the middle section of the bottom base. (This is the bottom side of the rectangle.) This length will be equal to the length of the top base, because opposite sides of a rectangle are of equal length.[7]

    • For example, if the top base of the trapezoid is 6 cm, then the middle section of the bottom base is also 6 cm.

  4. Image titled Find the Perimeter of a Trapezoid Step 16

    4

  5. Image titled Find the Perimeter of a Trapezoid Step 17

    5

    Plug the known values into the sine ratio. Make sure you use the height of the triangle as the length of the opposite side in the formula. You will solve for H.

    • For example, if the given interior angle is 35 degrees, and the height of the triangle is 6 cm, your formula will look like this:
      sin(35)={frac {6}{H}}

  6. Image titled Find the Perimeter of a Trapezoid Step 18

    6

    Find the sine of the angle. Do this by using the SIN button on a scientific calculator. Plug this value into the ratio.

    • For example, by using a calculator you will find that the sine of a 35 degree angle is .5738 (rounded). So your formula will now be:
      .5738={frac {6}{H}}

  7. Image titled Find the Perimeter of a Trapezoid Step 19

    7

    Solve for H. To do this, multiply each side by H, then divide each side by the angle sine. Or, you can simply divide the height of the triangle by the angle sine.

  8. Image titled Find the Perimeter of a Trapezoid Step 20

    8

    Find the length of the hypotenuse of the second right triangle. Set up the sine ratio (sin theta ={frac {{text{opposite}}}{{text{hypotenuse}}}}) for the second given interior angle. This will give you the length of the hypotenuse, which is also the first side of the trapezoid.

  9. Image titled Find the Perimeter of a Trapezoid Step 21

    9

    Set up the Pythagorean Theorem formula for the first right triangle. The Pythagorean Theorem formula is a^{{2}}+b^{{2}}=c^{{2}}, where the length of the hypotenuse is c, and the height of the triangle is a.

  10. Image titled Find the Perimeter of a Trapezoid Step 22

    10

  11. Image titled Find the Perimeter of a Trapezoid Step 23

    11

    Solve for b. This will give you the length of base of the first right triangle, and the first missing section of the trapezoid’s bottom base.

  12. Image titled Find the Perimeter of a Trapezoid Step 24

    12

  13. Image titled Find the Perimeter of a Trapezoid Step 25

    13

    Add up all the side lengths of the trapezoid. The perimeter of any polygon is the sum of all sides: P=T+B+L+R. For the bottom base, you will add the bottom side of the rectangle, plus the bases of the two triangles.

    • For example, 6+(8.5639+6+6)+10.4566+8.4854=45.5059
      So, the approximate perimeter of your trapezoid is 45.5059 cm.

    14. Advertisement

Add New Question

  • Question

    How can I solve the hypotenuse of a right triangle with a height of 2ft?

    Donagan

    You don’t have enough information to find the hypotenuse. You would need the lengths of both legs or the size of at least one of the acute angles or the area of the triangle.

  • Question

    How do I find the area without knowing the length of the sides of the trapezoid?

    Donagan

    You would have to know the height of the trapezoid (h) and the lengths of both parallel sides (a and b). The area formula is [h(a + b)] / 2.

  • Question

    Why are there so many formulas?

    Donagan

    It’s because there are several possible sets of known dimensions regarding a trapezoid.

Ask a Question

200 characters left

Include your email address to get a message when this question is answered.

Submit

Advertisement

  • Use the laws of special triangles to find the missing lengths of special triangles without using sine or the Pythagorean Theorem. The laws apply to a 30-60-90 triangle, or a 90-45-45 triangle.

  • Use a scientific calculator to find the sine of an angle by entering the angle measurement, then hitting the “SIN” button. You can also use a trigonometry table.

Thanks for submitting a tip for review!

Advertisement

Things You’ll Need

  • Calculator
  • Pencil
  • Paper

References

About This Article

Article SummaryX

To find the perimeter of a trapezoid if you know the length of both sides and the bases, add together the length of all 4 sides. If you know the height, both side lengths, and the top base length, draw a straight line down from each top corner to form a square and 2 triangles. Then, use the Pythagorean Theorem to find the length of the base of each triangle. Add the length of each triangle base to the length of the top base, then add that to the top base and both sides to get the perimeter. To learn more about using the Pythagorean Theorem, keep reading!

Did this summary help you?

Thanks to all authors for creating a page that has been read 264,525 times.

Did this article help you?

Источник

Добавить комментарий