Площадь квадрата можно найти с помощью двух основных формул:
1) Через сторону.
2) Через диагональ.
Как найти площадь квадрата, если известна его сторона.
Как известно, квадрат – это частный случай прямоугольника, у которого все стороны равны.
Площадь прямоугольника равна произведению 2 его сторон:
Sпр = a * b, a и b – стороны прямоугольника.
В случае с квадратом a = b.
Таким образом, площадь квадрата будет находиться по формуле:
Sкв = a².
Например, если сторона квадрата равна 10 см., то его площадь = 10 * 10 = 100 см².
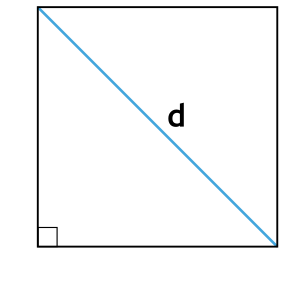
Как найти площадь квадрата, если известна его диагональ.
Диагональ делит квадрат на 2 прямоугольных треугольника. При этом диагональ является гипотенузой, а стороны квадрата – катетами.
Нам нужно выразить квадрат стороны через теорему Пифагора. Согласно данной теореме:
a*a + b*b = c*c.
a и b – катеты, c – гипотенуза.
В нашем случае a = b, а гипотенуза – это диагональ d.
Перепишем формулу в виде:
2a² = d².
a² = d² / 2.
Таким образом, если известна диагональ квадрата, то его площадь равна половине квадрата этой диагонали.
Например, если диагональ равна 10 см., то площадь квадрата = 10 * 10 / 2 = 50 см².

{S = a ^2}
На этой странице вы найдете удобный калькулятор для расчета площади квадрата и формулы, которые помогут найти площадь квадрата через его сторону, диагональ, периметр, а также радиусы вписанной и описанной окружности.
Квадрат – четырёхугольник, у которого все углы прямые (90 градусов) и все стороны равны между собой. Из-за своих свойств квадрат часто называют правильным четырехугольником.
Содержание:
- калькулятор площади квадрата
- формула площади квадрата через сторону
- формула площади квадрата через диагональ
- формула площади квадрата через радиус вписанной окружности
- формула площади квадрата через радиус описанной окружности
- формула площади квадрата через периметр
- примеры задач
Формула площади квадрата через сторону
S = a ^2
a – сторона квадрата
Формула площади квадрата через диагональ
S=dfrac{d^2}{2}
d – диагональ квадрата
Формула площади квадрата через радиус вписанной окружности
S = 4r^2
r – радиус вписанной окружности
Формула площади квадрата через радиус описанной окружности
S = 2R^2
R – радиус описанной окружности
Формула площади квадрата через периметр
S = dfrac{P^2}{16}
P – периметр квадрата
Примеры задач на нахождение площади квадрата
Задача 1
Найдите площадь квадрата если его диагональ равна 1.
Решение
Для решения задачи воспользуемся формулой.
S = dfrac{d^2}{2} = dfrac{1^2}{2} = dfrac{1}{2} = 0.5 : см^2
Ответ: 0.5 см²
Проверим ответ на калькуляторе .
Задача 2
Найдите площадь квадрата, описанного вокруг окружности радиуса 83.
Решение
Для решения этой задачи используем формулу площади квадрата через радиус описанной окружности.
S = 2R^2 = 2 cdot 83^2 = 2 cdot 6889 = 13778 : см^2
Ответ: 13778 см²
Проверим ответ с помощью калькулятора .
Задача 3
Найдите площадь квадрата если его сторона равна 8 см.
Решение
Используем первую формулу.
S = a ^2 = 8 ^2 = 64 : см^2
Ответ: 64 см²
Проверим результат на калькуляторе .
Задача 4
Найдите площадь квадрата периметр которого равен 456 см.
Решение
Используем формулу для площади квадрата через периметр.
S = dfrac{P^2}{16} = dfrac{456^2}{16} = dfrac{456 cdot cancel{456}^{ : 57}}{cancel{16}^{ : 2}} = dfrac{57 cdot cancel{456}^{ : 228}}{cancel{2}^{ : 1}} = 57 cdot 228 = 12996 : см^2
Ответ: 12996 см²
Проверка .
Задача 5
Найдите площадь квадрата со стороной 15 см.
Решение
Воспользуемся формулой площади квадрата через сторону.
S = a ^2 = 15 ^2 = 225 : см^2
Ответ: 225 см²
Проверка .
-
1
Write it down. Let’s say you’re working with a square with a side length of 3 centimeter (1.2 in). Write it down.
-
2
Understand the formula for the area of a square(Area=side^2). To calculate the area of any rectangle, you need to multiply its length by width. But since all squares have equal length sides, you can just multiply the distance by itself. If the length of a side of a square is 3 centimeter (1.2 in), then you just have to square 3 centimeter (1.2 in) to find the area of a square. 3 centimeter (1.2 in) x 3 centimeter (1.2 in) = 9 cm2.[1]
Advertisement
-
3
Be sure to state your answer in square units. Then you’re done.[2]
- Squaring the side of a square is the same thing as multiplying the square’s height times its base.
Advertisement
-
1
Take the measurement of the length of the diagonal of the square.
-
2
Understand the formula for the area using a known diagonal. Area = (diagonal^2)/2.[3]
-
3
Multiply the length of this diagonal’s measurement by itself. Square the length of the diagonal. Let’s say you’re working with a square with a diagonal that is 5 centimeter (2.0 in) long. Now, square this number. 5 centimeter (2.0 in) x 5 centimeter (2.0 in) = 25 cm2.
-
4
Divide the current number by 2. Continuing the calculation, 25 cm2 is divided by 2. This gives 12.5 cm2. You’re done.
Advertisement
-
1
Multiply the perimeter by 1/4 to find the length of a side. This is the same as dividing the perimeter by 4. Since there are four sides to a square and each side is of equal length, you can find the length of a square just by dividing the perimeter by 4. Let’s say the perimeter of the square you’re working with is 20 centimeter (7.9 in). Just multiply 20 centimeter (7.9 in) by 1/4: 20 centimeter (7.9 in) x 1/4 = 5 centimeter (2.0 in). You know that the length of a side of the square is 5 centimeter (2.0 in).[4]
-
2
Multiply the length of the side by itself. Square the length of the side. Now that you know that the length of a side is 5 centimeter (2.0 in), you can square it to get the area of the square. Area = (5 cm)2 = 25 centimeter (9.8 in).2[5]
Advertisement
Add New Question
-
Question
If the area of the square is 9 cm, what is the volume of the cube?
Take the square root of 9 and cube it. The answer will be expressed in cm³.
-
Question
What is the formula to calculate the area of an isosceles triangle that has a base and equal sides?
Multiply the length of the base of the triangle by 0.5, and then multiply the length from the base to the highest point of the triangle. A = 0.5 x b x h.
-
Question
A square is 1 cm by 1 cm; what is the area?
Multiply 1 cm by 1 cm: the area is 1 square cm.
See more answers
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
Advertisement
Video
References
About This Article
Article SummaryX
To find the area of a square, use the formula a = side^2, where side is the length of one of the sides of the square. If you only know the perimeter of the square, you can find the area by dividing the perimeter by 4, which will give you the length of each side, and then plugging the side into the formula a = side^2. If you want to learn how to find the area of a square if you only know the length of a diagonal, keep reading!
Did this summary help you?
Thanks to all authors for creating a page that has been read 246,729 times.
Reader Success Stories
-
“This article really helped me in my assignment. I answered all of it and got a high score.”