Рассмотрим понятия угловой скорости и углового ускорения при вращении твердого тела в теории и на примерах решения задач.
Угловая скорость
Угловой скоростью называют скорость вращения тела, определяющуюся приращением угла поворота тела за некоторый промежуток (единицу) времени.
Обозначение угловой скорости: ω (омега).
Рассмотрим некоторое твердое тело, вращающееся относительно неподвижной оси.
С этим телом свяжем воображаемую плоскость П, которая совершает вращение вместе с заданным телом.
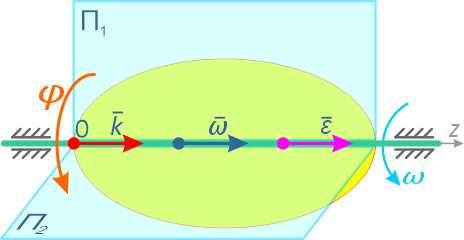
Вращательное движение определяется двугранным углом φ между двумя плоскостями, проходящими через ось вращения. Изменение этого угла с течением времени есть закон вращательного движения:
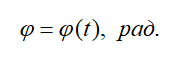
Положительным считается угол, откладываемый против хода часовой стрелки, если смотреть навстречу выбранному направлению оси вращения Oz. Угол измеряется в радианах.
Быстрота изменения угла φ (перемещения плоскости П из положения П1 в положение П2) – это и есть угловая скорость:
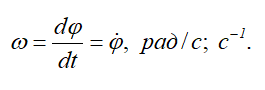
Приняв вектор k как единичный орт положительного направления оси, получим:
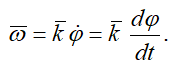
Вектор угловой скорости – скользящий вектор: он может быть приложен к любой точке оси вращения и всегда направлен вдоль оси, при положительном значении угловой скорости направления ω и k совпадают, при отрицательном – противоположны.
Формулы угловой скорости
Формула для расчета угловой скорости в зависимости от заданных параметров вращения может иметь вид:
- если известно количество оборотов n за единицу времени t:
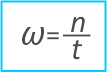
- если задан угол поворота φ за единицу времени:
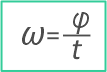
- если известна окружная скорость точки тела v и расстояние от оси вращения до этой точки r:
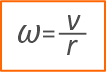
Размерности угловой скорости:
- Количество оборотов за единицу времени [об/мин], [c-1].
- Угол поворота за единицу времени [рад/с].
Определение угловой скорости
Пример: Диск вращается относительно своего центра.
Известна скорость v некоторой точки A, расположенной на расстоянии r от центра вращения диска.
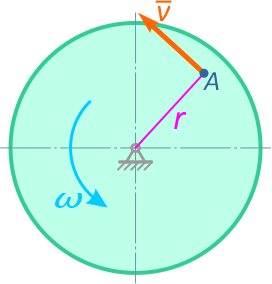
Определить величину и направление угловой скорости диска ω, если v = 5 м/с, r = 70 см.
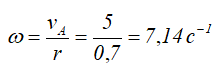
Таким образом, угловая скорость диска составляет 7,14 оборотов в секунду. Направление угловой скорости можно определить по направлению скоростей её точек.
Вектор скорости точки A стремится повернуть диск относительно центра вращения против хода часовой стрелки, следовательно, направление угловой скорости вращения диска имеет такое же направление.
Другие примеры решения задач >
Угловое ускорение
Угловое ускорение характеризует величину изменения угловой скорости при вращении твердого тела:
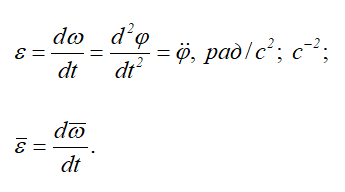
Обозначение: ε (Эпсилон)
Единицы измерения углового ускорения: [рад/с2], [с-2]
Вектор углового ускорения так же направлен по оси вращения. При ускоренном вращении их направления совпадают, при замедленном — противоположны.
Другими словами, при положительном ускорении угловая скорость нарастает (вращение ускоряется), а при отрицательном — уменьшается (вращение замедляется).
Для некоторых частных случаев вращательного движения твердого тела могут быть использованы формулы:
Расчет углового ускорения
Пример: По заданному значению касательной составляющей полного ускорения aτ точки B, расположенной на расстоянии r от центра вращения колеса.
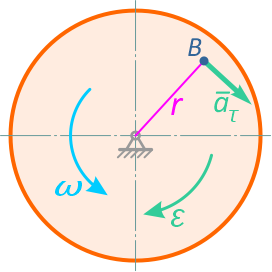
Требуется определить величину и направление углового ускорения колеса ε, если aτ = 10 м/с2, r = 50 см.
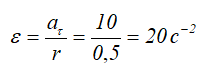
Угловое ускорение колеса в заданный момент времени составляет 20 оборотов за секунду в квадрате. Направление углового ускорения определяется по направлению тангенциального ускорения точки.
Здесь, угловое ускорение направлено противоположно направлению угловой скорости вращения колеса. Это означает, что вращение колеса замедляется.
В технике угловая скорость часто задается в оборотах в минуту n [об/мин]. Один оборот – это 2π радиан:
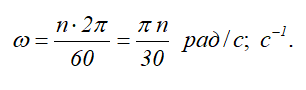
Например, тело совершающее 1,5 оборота за одну секунду имеет угловую скорость
ω = 1,5 с-1 = 9,42 рад/с.
Смотрите также:
- Примеры расчета угловой скорости и ускорения
- Скорости и ускорения точек вращающегося тела
Покажем рисунок.
Момент сил которые действуют на барабан определим по формуле:
М = J∙ε (1), М = FН∙R (2).
J – момент инерции маховика, ε – угловое ускорение движения маховика, FН – сила натяжения нити, R – радиус барабана.
Момент инерции однородного диска определяется по формуле:
[ J=frac{{{m}_{1}}cdot {{R}^{2}}}{2}(3). ]
Определим угловое ускорение движения диска. Груз из состояния покоя движется равноускоренно. Сравним (1) и (2) выразим силу натяжения нити.
[ begin{align}
& varepsilon =frac{a}{R} (4).M=frac{{{m}_{1}}cdot {{R}^{2}}}{2}cdot frac{a}{R},M=frac{{{m}_{1}}cdot Rcdot a}{2},(5),{{F}_{H}}cdot R=frac{{{m}_{1}}cdot Rcdot a}{2}, \
& {{F}_{H}}=frac{{{m}_{1}}cdot a}{2}(6). \
end{align} ]
Используя второй закон Ньютона определим силу натяжения нити.
[ begin{align}
& vec{F}=mcdot vec{a}, {{{vec{F}}}_{H}}+mcdot vec{g}=mcdot vec{a}, \
& Oy: -{{F}_{H}}+mcdot g=mcdot a, {{F}_{H}}=mcdot g-mcdot a (7). \
end{align} ]
(6) подставим в (7) выразим ускорение.
[ begin{align}
& mcdot g-mcdot a=frac{{{m}_{1}}cdot a}{2},2cdot mcdot g-2cdot mcdot a={{m}_{1}}cdot a,a=frac{2cdot mcdot g}{2cdot m+{{m}_{1}}}(8). \
& a=frac{2cdot 2cdot 10}{2cdot 2+9}=3. \
end{align}
]
Ответ: 3 м/с2.
Барабан косилки – измельчителя достигает рабочей частоты вращения в 1500 об/мин через 2 мин от начала движения. Вычислить угловое ускорение барабана, тангенциальное и полное ускорение точек на его поверхности. Диаметр барабана 570 мм.
Угловая скорость через 2 мин после начала:
$w_2=2pi n=frac{2*3,14*1500}{60}=157$ рад/с
Угловое ускорение:
$varepsilon=frac{w_2-w_1}{t}=frac{157-0}{2*60}approx 1,3$ рад/с^2
Линейная скорость через 2 мин после начала:
$v_2=w_2R=157*frac{0,57}{2}approx 44,75$ м/с
Тангенциальное ускорение:
$a_{tau}=frac{v_2-v_1}{dt}=frac{44,75-0}{2*60-0}approx 0,373$ м/с^2
Нормальное ускорение в момент через 2 минуты после начала:
$a_n=frac{v_2^2}{R}=frac{44,75^2}{frac{0,57}{2}}approx 7027$ м/с^2
Полное ускорение:
$a=sqrt{a_{tau}^2+a_n^2}$
$a=sqrt{0,373^2+7027^2}approx 7927$ м/с^2
Download Article
Different ways to calculate rotation speed
Download Article
Most people have a general understanding of the idea of velocity and acceleration. Velocity is the measure of how fast an object is moving, and acceleration is the measure of how quickly the object’s velocity is changing (i.e., speeding up or slowing down). When the object is moving in a circle, such as a spinning tire or a rotating CD, velocity and acceleration are generally measured by the angle of rotation. They are then called angular velocity and angular acceleration. If you know the object’s velocity over some period of time, you can calculate its average angular acceleration. Alternatively, you may have a function to calculate the object’s position. With this information, you can calculate its angular acceleration at any chosen instant.
-

1
Determine the function for angular position. In some cases, you may be provided with a function or formula that predicts or assigns the position of an object with respect to time. In other cases, you may derive the function from repeated experiments or observations. For this article, we assume that the function has been provided or previously calculated.[1]
-

2
Find the function for angular velocity. Velocity is the measure of how fast an object changes its position. In layman’s terms, we think of this as its speed. In mathematical terms, the change of position over time can be found by finding the derivative of the position function. The symbol for angular velocity is
. Angular velocity is generally measured in units of radians divided by time (radians per minute, radians per second, etc.).[2]
Advertisement
-

3
Find the function for angular acceleration. Acceleration is the measure of how fast an object’s velocity is changing over time. You can mathematically calculate the angular acceleration by finding the derivative of the function for angular velocity. Angular acceleration is generally symbolized with
, the Greek letter alpha. Angular acceleration is reported in units of velocity per time, or generally radians divided by time squared (radians per second squared, radians per minute squared, etc.).[3]
-

4
Apply the data to find instantaneous acceleration. Once you have derived the function for instantaneous acceleration as the derivative of velocity, which in turn is the derivative of position, you are ready to calculate the instantaneous angular acceleration of the object at any chosen time.[4]
Advertisement
-

1
-

2
Measure final angular velocity. The second piece of information that you need is the angular velocity of the spinning or rotating object at the end of the time period that you want to measure. This is to be called the “final” velocity.[6]
- A compact disc plays in the machine by rotating at an angular velocity of 160 radians per second.
- The roller coaster, after applying its brakes to the spinning wheels, ultimately reaches an angular velocity of zero when it stops. This will be its final angular velocity.
-

3
Measure the elapsed time. To calculate the average angular velocity of the spinning or rotating object, you need to know the amount of time that passes during your observation. This can be found by direct observation and measurement, or the information can be provided for a given problem.[7]
- The owner’s manual for the CD player provides the information that the CD reaches its playing speed in 4.0 seconds.
- From observations of roller coasters being tested, it has been found that they can come to a complete stop within 2.2 seconds from when the brakes are initially applied.
-

4
Calculate the average angular acceleration. If you know the initial angular velocity, the final angular velocity, and the elapsed time, fill that data into the equation and find the average angular acceleration.[8]
Advertisement
-

1
Understand the concept of angular motion. When people think of the speed of an object, they often consider linear motion – that is, objects traveling mostly in a straight line. This would include a car, a plane, a ball that is thrown or any number of other objects. However, angular motion describes objects that spin or rotate. Think of the earth spinning on its axis. The position or speed of the earth can be measured with angular quantities. A spinning compact disc (or record player, if you’re old enough), electrons on their axes, or the wheels of a car on the axle are other examples of rotating objects that can be measured through angular motion.[9]
-

2
Visualize angular position. When you measure the position of a moving vehicle, for example, you can measure the distance traveled in a straight line from the starting point. With a rotating object, the measurement is generally done in terms of the angle around a circle. By convention, the starting or “zero” point is generally a horizontal radius from the center to the right side of the circle. The distance traveled is measured by the size of the angle
, measured from that horizontal radius.[10]
- The angle that is being measured is commonly represented by
, the Greek letter theta.
- Positive motion is measured in a counterclockwise direction. Negative motion is measured in a clockwise direction.
- The angle that is being measured is commonly represented by
-

3
Measure angular motion in radians. Linear travel is generally measured in some unit of distance, such as miles, meters, inches or some other unit of length. Rotational or angular motion is generally measured in units called radian. A radian is a fraction of the circle. For standard reference, mathematicians use the “unit circle,” which has a standard radius of 1 unit.[11]
-

4
Understand the concept of angular acceleration. Angular acceleration is the measurement of how fast or slow a rotating object is changing its velocity. In other words, is the spinning speeding up or slowing down? If you know the angular velocity at a starting time and then at a later ending time, you can calculate the average angular acceleration over that time interval. If you know the function for the object’s position, you can use calculus to derive the instantaneous angular acceleration at any chosen time.[12]
- People often use the word “acceleration” to mean speeding up, and “deceleration” to mean slowing down. In mathematical and physical terms, however, only the word “acceleration” is used. If the object is speeding up, the acceleration is positive. If it is slowing down, the acceleration is negative.
Advertisement
Add New Question
-
Question
What are the formulas to find the initial acceleration of an object?

Initial acceleration generally has to be given as a condition of the problem or the experiment.
-
Question
What is the direction of radial and tangential acceleration and how do they affect each other?

Angular (or radial) measurements are generally counterclockwise. Tangential acceleration means the straight line direction of the tangent at some measured point along the circle. The tangent is a line that is perpendicular to the radius at that point.
-
Question
How can you find angular acceleration in revolutions per second squared?

This article shows how to find acceleration in radians per second squared. To convert the number of radians to the number of revolutions, recall that 1 full circle (or 1 revolution) is equal to 2pi radians. This is roughly equivalent to 6.28 radians per revolution. If you know the acceleration in radians per second squared, divide that answer by 6.28 to get revolutions per second squared.
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
Advertisement
Video
-
Remember to express final results with the proper units. Angular position is usually expressed in radians. Angular velocity is expressed in radians per time. Angular acceleration is expressed in units of radians per time squared.
Thanks for submitting a tip for review!
Advertisement
References
About This Article
Article SummaryX
To calculate instantaneous angular acceleration, start by determining the function for angular position, or the position of the object with respect to time. Next, find the angular velocity, which is the measure of how fast the object changes its position. Then, find the derivative of the function for angular velocity in order to determine the function for angular acceleration. Finally, plug in the data to find the instantaneous acceleration of the object at any chosen time. To learn more, including how to calculate average angular acceleration, read on.
Did this summary help you?
Thanks to all authors for creating a page that has been read 90,827 times.
