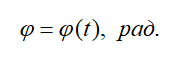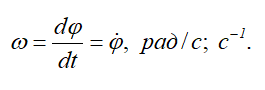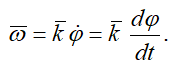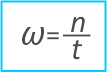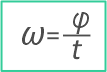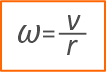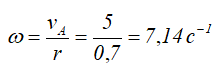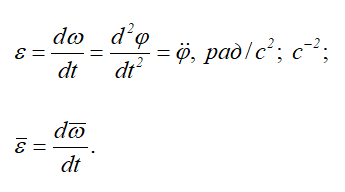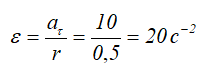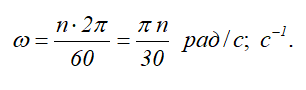Как найти угловую скорость часовой стрелки часов

Популярное на сайте:

Как быстро выучить стихотворение наизусть? Запоминание стихов является стандартным заданием во многих школах. 

Как научится читать по диагонали? Скорость чтения зависит от скорости восприятия каждого отдельного слова в тексте. 

Как быстро и эффективно исправить почерк?  Люди часто предполагают, что каллиграфия и почерк являются синонимами, но это не так.

Как научится говорить грамотно и правильно? Общение на хорошем, уверенном и естественном русском языке является достижимой целью. 

Рассмотрим понятия угловой скорости и углового ускорения при вращении твердого тела в теории и на примерах решения задач.

Угловая скорость

Угловой скоростью называют скорость вращения тела, определяющуюся приращением угла поворота тела за некоторый промежуток (единицу) времени.

Обозначение угловой скорости: ω (омега).

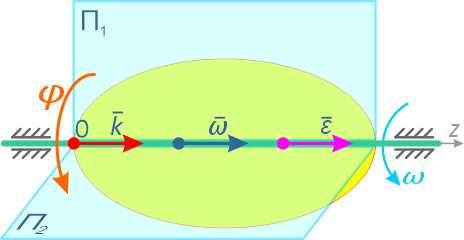
Рассмотрим некоторое твердое тело, вращающееся относительно неподвижной оси.

С этим телом свяжем воображаемую плоскость П, которая совершает вращение вместе с заданным телом.

Вращательное движение определяется двугранным углом φ между двумя плоскостями, проходящими через ось вращения. Изменение этого угла с течением времени есть закон вращательного движения:

Положительным считается угол, откладываемый против хода часовой стрелки, если смотреть навстречу выбранному направлению оси вращения Oz. Угол измеряется в радианах.

Быстрота изменения угла φ (перемещения плоскости П из положения П₁ в положение П₂) – это и есть угловая скорость:

Приняв вектор k как единичный орт положительного направления оси, получим:

Вектор угловой скорости – скользящий вектор: он может быть приложен к любой точке оси вращения и всегда направлен вдоль оси, при положительном значении угловой скорости направления ω и k совпадают, при отрицательном – противоположны.

Формулы угловой скорости

Формула для расчета угловой скорости в зависимости от заданных параметров вращения может иметь вид:

1. если известно количество оборотов n за единицу времени t:
   
2. если задан угол поворота φ за единицу времени:
   
3. если известна окружная скорость точки тела v и расстояние от оси вращения до этой точки r:
   

Размерности угловой скорости:

• Количество оборотов за единицу времени [об/мин], [c^-1].
• Угол поворота за единицу времени [рад/с].

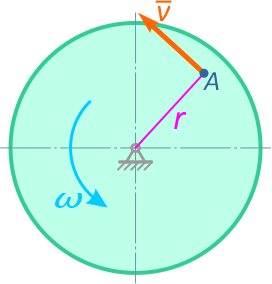
Определение угловой скорости

Пример: Диск вращается относительно своего центра.
Известна скорость v некоторой точки A, расположенной на расстоянии r от центра вращения диска.

Определить величину и направление угловой скорости диска ω, если v = 5 м/с, r = 70 см.

Таким образом, угловая скорость диска составляет 7,14 оборотов в секунду. Направление угловой скорости можно определить по направлению скоростей её точек.

Вектор скорости точки A стремится повернуть диск относительно центра вращения против хода часовой стрелки, следовательно, направление угловой скорости вращения диска имеет такое же направление.

Другие примеры решения задач >

Угловое ускорение

Угловое ускорение характеризует величину изменения угловой скорости при вращении твердого тела:

Обозначение: ε (Эпсилон)

Единицы измерения углового ускорения: [рад/с²], [с^-2]

Вектор углового ускорения так же направлен по оси вращения. При ускоренном вращении их направления совпадают, при замедленном — противоположны.

Другими словами, при положительном ускорении угловая скорость нарастает (вращение ускоряется), а при отрицательном — уменьшается (вращение замедляется).

Для некоторых частных случаев вращательного движения твердого тела могут быть использованы формулы:

Расчет углового ускорения

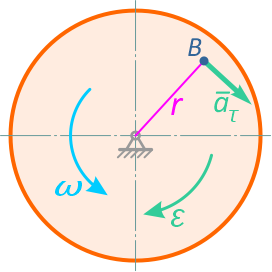
Пример: По заданному значению касательной составляющей полного ускорения a_τ точки B, расположенной на расстоянии r от центра вращения колеса.

Требуется определить величину и направление углового ускорения колеса ε, если a_τ = 10 м/с², r = 50 см.

Угловое ускорение колеса в заданный момент времени составляет 20 оборотов за секунду в квадрате. Направление углового ускорения определяется по направлению тангенциального ускорения точки.

Здесь, угловое ускорение направлено противоположно направлению угловой скорости вращения колеса. Это означает, что вращение колеса замедляется.

В технике угловая скорость часто задается в оборотах в минуту n [об/мин]. Один оборот – это 2π радиан:

Например, тело совершающее 1,5 оборота за одну секунду имеет угловую скорость

ω = 1,5 с^-1 = 9,42 рад/с.

Смотрите также:

• Примеры расчета угловой скорости и ускорения
• Скорости и ускорения точек вращающегося тела

In everyday discourse, “speed” and “velocity” are often used interchangeably. In physics, however, these terms have specific and distinct meanings. “Speed” is the rate of displacement of an object in space, and it is given only by a number with specific units (often in meters per second or miles per hour). Velocity, on the other hand, is a speed coupled to a direction. Speed, then, is called a scalar quantity, whereas velocity is a vector quantity.

When a car is zipping along a highway or a baseball is whizzing through the air, the speed of these objects is measured in reference to the ground, whereas the velocity incorporates more information. For example, if you’re in a car traveling at 70 miles per hour on Interstate 95 on the East Coast of the United States, it’s also helpful to know whether it is headed northeast toward Boston or south toward Florida. With baseball, you might want to know if its y-coordinate is changing more rapidly than its x-coordinate (a fly ball) or if the reverse is true (a line drive). But what about the spinning of the tires or the rotation (spin) of the baseball as the car and the ball move toward their ultimate destination? For these kinds of questions, physics offers the concept of ‌angular‌ ‌velocity‌.

The Basics of Motion

Things move through three-dimensional physical space in two main ways: translation and rotation. Translation is the displacement of the entire object from one location to another, like a car driving from New York City to Los Angeles. Rotation, on the other hand, is the cyclical motion of an object around a fixed point. Many objects, such as the baseball in the above example, exhibit both types of movement at the same time; as a fly ball moves through the air from home plate toward the outfield fence, it also spins at a given rate around its own center.

Describing these two kinds of motion are treated as separate physics problems; that is, when calculating the distance the ball travels through the air based on things like its initial launch angle and the speed with which it leaves the bat, you can ignore its rotation, and when calculating its rotation you can treat it as sitting in one place for present purposes.

The Angular Velocity Equation

First, when you are talking about “angular” anything, be it velocity or some other physical quantity, recognize that, because you are dealing with angles, you’re talking about traveling in circles or portions thereof. You may recall from geometry or trigonometry that the circumference of a circle is its diameter times the constant pi, or ‌πd‌. (The value of pi is about 3.14159.) This is more commonly expressed in terms of the circle’s radius ‌r‌;, which is half the diameter, making the circumference ‌2πr‌.

In addition, you have probably learned somewhere along the way that a circle consists of 360 degrees (360°). If you move a distance S along a circle, than the angular displacement θ is equal to S/r. One full revolution, then, gives 2πr/r, which just leaves 2 * π rad. That means angles can be expressed in terms of pi, or in other words, as radians.

Taking all of these pieces of information together, you can express angles, or portions of a circle, in units other than degrees:

180^circ = pi text{rad} \ text{} \ text{Radians} = text{degrees} cdotfrac{pi}{180^circ}

Whereas linear velocity is expressed in length per unit time, the units of angular velocity are measured in radians per unit time, usually per second.

If you know that a particle is moving in a circular path with a velocity ‌v‌ at a distance ‌r‌ from the center of the circle, with the direction of ‌v‌ always being perpendicular to the radius of the circle, then the average angular velocity can be written as:

omega = frac{Delta v}{r}

where angular velocity ‌ω‌ is the Greek letter omega. Angular velocity units are radians per second; you can also treat this unit as “reciprocal seconds,” because v/r yields m/s divided by m, or s^-1, meaning that radians technically have no units.

Rotational Motion Equations

The angular acceleration formula is derived in the same essential way as the angular velocity formula: We can find angular acceleration by finding the change in angular velocity over a certain change in time. This is average angular acceleration:

alpha = frac{Delta omega}{Delta t}

If angular acceleration is zero, then the object has uniform circular motion meaning there is constant angular velocity (i.e. the velocity vector will have a constant magnitude).

Other Quantities of Angular Rotation

Rotational kinematics also has more descriptive quantities involved; tangential velocity, tangential acceleration, and centripetal acceleration that are all key pieces of circular motion.

‌α‌, as you probably know, is the Greek letter “alpha,” and it denotes angular acceleration, while ‌ω‌ references angular velocity. When discussing tangential quantities, this refers to a linear speed or acceleration whose vector is a straight line tangential to the circle. These quantities are measured in traditional kinematic units where tangential velocity is meters per second and tangential acceleration is meters per second squared. Given tangential velocity ‌v‌ and tangential acceleration ‌a:‌

a = frac{v}{t}

Curiously enough, however, rotational motion boasts another kind of acceleration, called centripetal (“center-seeking”) acceleration. This is given by the expression:

a_c = frac{v^2}{r}

Centripetal force is then given with Newton’s second equation:

F_c = frac{mv^2}{r}

This acceleration is directed toward the point around which the object in question is rotating. This may seem strange, since the object is getting no closer to this central point since the radius ‌r‌ is fixed. Think of centripetal acceleration as a free-fall in which there is no danger of the object hitting the ground, because the force drawing the object toward it (usually gravity) is exactly offset by the tangential (linear) acceleration described by the first equation in this section. If ‌a_c‌ were not equal to ‌a‌, the object would either fly off into space or soon crash into the middle of the circle.

Related Quantities and Expressions

Although angular velocity is usually expressed, as noted, in radians per second, there may be instances in which it is preferable or necessary to use degrees per second instead, or conversely, to convert from degrees to radians before solving a problem.

Say you were told that a light source rotates through 90° every second at a constant velocity. What is its angular velocity in radians?

First, remember that 2π radians = 360°, and set up a proportion:

frac{360}{2pi}=frac{90}{omega}implies 360omega =180piimplies omega =frac{pi}{2}

The answer is one-half pi radians per second.

If you were further told that the light beam has a range of 10 meters, what would be the tip of the beam’s linear velocity ‌v‌, its angular acceleration ‌α‌ and its centripetal acceleration ‌a_c‌?

To solve for ‌v‌, from above, v = ωr, where ω = π/2 and r = 10m:

frac{pi}{2} 10=15.7text{ m/s}

To find ‌α‌, assume the angular speed is reached in 1 second, then:

alpha = frac{omega}{t}=frac{pi /2}{1}=frac{pi}{2}text{ rad/s}^2

(Note that this only works for problems in which the angular velocity is constant.)

Finally, also from above,

a_c=frac{v^2}{r}=frac{15.7^2}{10}=24.65text{ m/s}^2

Angular Velocity vs. Linear Velocity

Building on the previous problem, imagine yourself on a very large merry-go-round, one with an unlikely radius of 10 kilometers (10,000 meters). This merry-go-round makes one complete revolution every 1 minute and 40 seconds, or every 100 seconds.

One consequence of the difference between angular velocity, which is independent of the distance from the axis of rotation, and linear circular velocity, which is not, is that two people experiencing the same ‌ω‌ may be undergoing vastly different physical experiences. If you happen to be 1 meter from the center if this putative, massive merry-go-round, your linear (tangential) velocity is:

v=omega r = frac{2pi}{100}(1)=0.0628text{ m/s}

or 6.29 cm (less than 3 inches) per second.

But if you’re on the rim of this monster, your linear velocity is:

v=omega r = frac{2pi}{100}(10000)=628text{ m/s}

That’s about 1,406 miles per hour, faster than a bullet. Hang on!

Angular Momentum

Angular momentum, L, is based upon the moment of inertia and the angular velocity given in the equation (also related to mass, radius, and velocity):

L = I omega = rmv

The center of mass plays a critical role in defining angular momentum through the moment of inertia, and the center of rotation also impacts this quantity, as the radius could be changing with respect to rotation angle.